ASTM D5270-96(2002)
(Test Method)Standard Test Method for Determining Transmissivity and Storage Coefficient of Bounded, Nonleaky, Confined Aquifers
Standard Test Method for Determining Transmissivity and Storage Coefficient of Bounded, Nonleaky, Confined Aquifers
SIGNIFICANCE AND USE
Assumptions:
5.1.1 The well discharges at a constant rate.
5.1.2 Well is of infinitesimal diameter and is open through the full thickness of the aquifer.
5.1.3 The nonleaky confined aquifer is homogeneous, isotropic, and areally extensive except where limited by linear boundaries.
5.1.4 Discharge from the well is derived initially from storage in the aquifer; later, movement of water may be induced from a constant-head boundary into the aquifer.
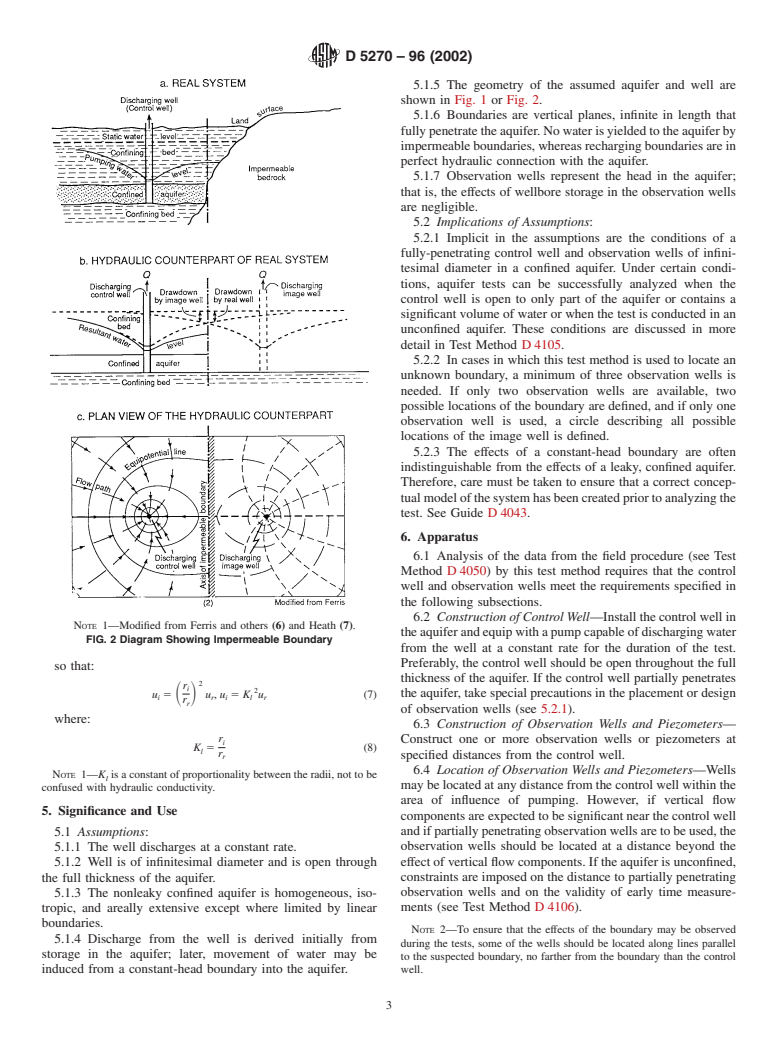
5.1.5 The geometry of the assumed aquifer and well are shown in Fig. 1 or Fig. 2.
5.1.6 Boundaries are vertical planes, infinite in length that fully penetrate the aquifer. No water is yielded to the aquifer by impermeable boundaries, whereas recharging boundaries are in perfect hydraulic connection with the aquifer.
5.1.7 Observation wells represent the head in the aquifer; that is, the effects of wellbore storage in the observation wells are negligible.
Implications of Assumptions:
5.2.1 Implicit in the assumptions are the conditions of a fully-penetrating control well and observation wells of infinitesimal diameter in a confined aquifer. Under certain conditions, aquifer tests can be successfully analyzed when the control well is open to only part of the aquifer or contains a significant volume of water or when the test is conducted in an unconfined aquifer. These conditions are discussed in more detail in Test Method D 4105.
5.2.2 In cases in which this test method is used to locate an unknown boundary, a minimum of three observation wells is needed. If only two observation wells are available, two possible locations of the boundary are defined, and if only one observation well is used, a circle describing all possible locations of the image well is defined.
5.2.3 The effects of a constant-head boundary are often indistinguishable from the effects of a leaky, confined aquifer. Therefore, care must be taken to ensure that a correct conceptual model of the system has been created prior to analyzing ...
SCOPE
1.1 This test method covers an analytical procedure for determining the transmissivity, storage coefficient, and possible location of boundaries for a confined aquifer with a linear boundary. This test method is used to analyze water-level or head data from one or more observation wells or piezometers during the pumping of water from a control well at a constant rate. This test method also applies to flowing artesian wells discharging at a constant rate. With appropriate changes in sign, this test method also can be used to analyze the effects of injecting water into a control well at a constant rate.
1.2 The analytical procedure in this test method is used in conjunction with the field procedure in Test Method D 4050.
1.3 Limitations—The valid use of this test method is limited to determination of transmissivities and storage coefficients for aquifers in hydrogeologic settings with reasonable correspondence to the assumptions of the Theis nonequilibrium method (see Test Method D 4106) (see 5.1), except that the aquifer is limited in areal extent by a linear boundary that fully penetrates the aquifer. The boundary is assumed to be either a constant-head boundary (equivalent to a stream or lake that hydraulically fully penetrates the aquifer) or a no-flow (impermeable) boundary (equivalent to a contact with a significantly less permeable rock unit). The Theis nonequilibrium method is described in Test Methods D 4105 and D 4106.
1.4 The values stated in SI units are to be regarded as standard.
1.5 This standard does not purport to address all of the safety concerns, if any, associated with its use. It is the responsibility of the user of this standard to establish appropriate safety and health practices and determine the applicability of regulatory limitations prior to use.
General Information
Relations
Standards Content (Sample)
NOTICE: This standard has either been superseded and replaced by a new version or withdrawn.
Contact ASTM International (www.astm.org) for the latest information
Designation: D 5270 – 96 (Reapproved 2002)
Standard Test Method for
Determining Transmissivity and Storage Coefficient of
Bounded, Nonleaky, Confined Aquifers
This standard is issued under the fixed designation D5270; the number immediately following the designation indicates the year of
original adoption or, in the case of revision, the year of last revision.Anumber in parentheses indicates the year of last reapproval.A
superscript epsilon (´) indicates an editorial change since the last revision or reapproval.
1. Scope D653 Terminology Relating to Soil, Rock, and Contained
Fluids
1.1 This test method covers an analytical procedure for
D4043 Guide for Selection of Aquifer-Test Method in
determiningthetransmissivity,storagecoefficient,andpossible
Determining Hydraulic Properties by Well Techniques
location of boundaries for a confined aquifer with a linear
D4050 Test Method (Field Procedure) for Withdrawal and
boundary. This test method is used to analyze water-level or
Injection Well Tests for Determining Hydraulic Properties
head data from one or more observation wells or piezometers
of Aquifer Systems
during the pumping of water from a control well at a constant
D4105 Test Method (Analytical Procedure) for Determin-
rate. This test method also applies to flowing artesian wells
ing Transmissivity and Storage Coefficient of Nonleaky
discharging at a constant rate. With appropriate changes in
Confined Aquifers by the Modified Theis Nonequilibrium
sign, this test method also can be used to analyze the effects of
Method
injecting water into a control well at a constant rate.
D4106 Test Method (Analytical Procedure) for Determin-
1.2 The analytical procedure in this test method is used in
ing Transmissivity and Storage Coefficient of Nonleaky
conjunction with the field procedure in Test Method D4050.
Confined Aquifers by the Theis Nonequilibrium Method
1.3 Limitations—Thevaliduseofthistestmethodislimited
D4750 Test Method for Determining Subsurface Liquid
todeterminationoftransmissivitiesandstoragecoefficientsfor
Levels in a Borehole or Monitoring Well (Observation
aquifers in hydrogeologic settings with reasonable correspon-
Well)
dence to the assumptions of the Theis nonequilibrium method
(see Test Method D4106) (see 5.1), except that the aquifer is
3. Terminology
limitedinarealextentbyalinearboundarythatfullypenetrates
3.1 Definitions:
the aquifer. The boundary is assumed to be either a constant-
3.1.1 constant-head boundary—the conceptual representa-
head boundary (equivalent to a stream or lake that hydrauli-
tion of a natural feature such as a lake or river that effectively
cally fully penetrates the aquifer) or a no-flow (impermeable)
fully penetrates the aquifer and prevents water-level change in
boundary (equivalent to a contact with a significantly less
the aquifer at that location.
permeable rock unit). The Theis nonequilibrium method is
3.1.2 equipotential line—a line connecting points of equal
described in Test Methods D4105 and D4106.
hydraulic head. A set of such lines provides a contour map of
1.4 The values stated in SI units are to be regarded as
a potentiometric surface.
standard.
3.1.3 image well—an imaginary well located opposite a
1.5 This standard does not purport to address all of the
control well such that a boundary is the perpendicular bisector
safety concerns, if any, associated with its use. It is the
of a straight line connecting the control and image wells; used
responsibility of the user of this standard to establish appro-
to simulate the effect of a boundary on water-level changes.
priate safety and health practices and determine the applica-
3.1.4 impermeable boundary—the conceptual representa-
bility of regulatory limitations prior to use.
tion of a natural feature such as a fault or depositional contact
2. Referenced Documents that places a boundary of significantly less-permeable material
laterally adjacent to an aquifer.
2.1 ASTM Standards:
3.1.5 See Terminology D653 for other terms.
3.2 Symbols and Dimensions:
3.2.1 K [nd]—constant of proportionality, r /r .
1 l i r
ThistestmethodisunderthejurisdictionofASTMCommitteeD18onSoiland
3 −1
3.2.2 Q[L T ]—discharge.
RockandisthedirectresponsibilityofSubcommitteeD18.21onGroundWaterand
Vadose Zone Investigations.
Current edition approved July 10, 2002. Published February 1997. Originally
published as D5270–92. Last previous edition D5270–92. Annual Book of ASTM Standards, Vol 04.08.
Copyright © ASTM International, 100 Barr Harbor Drive, PO Box C700, West Conshohocken, PA 19428-2959, United States.
D 5270 – 96 (2002)
3.2.3 r [L]—radial distance from control well.
3.2.4 r [L]—distance from observation well to image well.
i
3.2.5 r [L]—distancefromobservationwelltocontrolwell.
r
3.2.6 S [nd]—storage coefficient.
3.2.7 s [L]—drawdown.
3.2.8 s [L]—component of drawdown due to image well.
i
3.2.9 s [L]—drawdown at an observation well.
o
3.2.10 s [L]—componentofdrawdownduetocontrolwell.
r
2 −1
3.2.11 T[L T ]—transmissivity.
3.2.12 t [T]—time since pumping or injection began.
3.2.13 t [T]—time at projection of zero drawdown.
o
4. Summary of Test Method
4.1 This test method prescribes two analytical procedures
for analysis of a field test. This test method requires pumping
water from, or injecting water into, a control well that is open
to the entire thickness of a confined bounded aquifer at a
constant rate and measuring the water-level response in one or
more observation wells or piezometers. The water-level re-
sponse in the aquifer is a function of the transmissivity and
storagecoefficientoftheaquifer,andthelocationandnatureof
the aquifer boundary or boundaries. Drawdown or build up of
the water level is analyzed as a departure from the type curve
defined by the Theis nonequilibrium method (see Test Method
D4106)orfromstraight-linesegmentsdefinedbythemodified
Theis nonequilibrium method (see Test Method D4105).
4.2 Aconstant-head boundary such as a lake or stream that
fully penetrates the aquifer prevents drawdown or build up of
head at the boundary, as shown in Fig. 1. Likewise, an
impermeable boundary provides increased drawdown or build
up of head, as shown in Fig. 2. These effects are simulated by
treating the aquifer as if it were infinite in extent and
introducing an imaginary well or “image well” on the opposite
side of the boundary a distance equal to the distance of the
controlwellfromtheboundary.Alinebetweenthecontrolwell
NOTE 1—Modified from Ferris and others (6) and Heath (7).
and the image well is perpendicular to the boundary. If the
FIG. 1 Diagram Showing Constant-Head Boundary
boundary is a constant-head boundary, the flux from the image
well is opposite in sign from that of the control well; for
example,theimageofadischargingcontrolwellisaninjection
r S
u 5 (2)
well, whereas the image of an injecting well is a discharging 4Tt
well. If the boundary is an impermeable boundary, the flux
where:
from the image well has the same sign as that from the control
2y
` e
well. Therefore, the image of a discharging well across an
dy 5 W u
~ !
*
u y
impermeable boundary is a discharging well. Because the
2 3 4
u u u
effects are symmetrical, only discharging control wells will be
520.577216 2log u 1 u 2 1 2 1.
e
2!2 3!3 4!4
described in the remainder of this test method, but this test
(3)
method is equally applicable, with the appropriate change in
4.4 According to the principle of superposition, the draw-
sign, to control wells into which water is injected.
down at any point in the aquifer is the sum of the drawdown
4.3 Solution—The solution given by Theis (1) can be
due to the real and image wells (1) and (2):
expressed as follows:
2y s 5 s 6 s (4)
o r i
Q ` e
s 5 dy (1)
*
4pT y Equation (4) can be rewritten as follows:
u
Q Q
and:
s 5 [W~u ! 6 W~u !# 5 ( W~u! (5)
o r i
4pT 4pT
where:
2 2
r S r S
The boldface numbers given in parentheses refer to a list of references at the r i
u 5 , u 5 (6)
r i
end of the text. 4Tt 4Tt
D 5270 – 96 (2002)
5.1.5 The geometry of the assumed aquifer and well are
shown in Fig. 1 or Fig. 2.
5.1.6 Boundaries are vertical planes, infinite in length that
fullypenetratetheaquifer.Nowaterisyieldedtotheaquiferby
impermeableboundaries,whereasrechargingboundariesarein
perfect hydraulic connection with the aquifer.
5.1.7 Observation wells represent the head in the aquifer;
that is, the effects of wellbore storage in the observation wells
are negligible.
5.2 Implications of Assumptions:
5.2.1 Implicit in the assumptions are the conditions of a
fully-penetrating control well and observation wells of infini-
tesimal diameter in a confined aquifer. Under certain condi-
tions, aquifer tests can be successfully analyzed when the
control well is open to only part of the aquifer or contains a
significant volume of water or when the test is conducted in an
unconfined aquifer. These conditions are discussed in more
detail in Test Method D4105.
5.2.2 In cases in which this test method is used to locate an
unknown boundary, a minimum of three observation wells is
needed. If only two observation wells are available, two
possible locations of the boundary are defined, and if only one
observation well is used, a circle describing all possible
locations of the image well is defined.
5.2.3 The effects of a constant-head boundary are often
indistinguishable from the effects of a leaky, confined aquifer.
Therefore, care must be taken to ensure that a correct concep-
tualmodelofthesystemhasbeencreatedpriortoanalyzingthe
test. See Guide D4043.
6. Apparatus
6.1 Analysis of the data from the field procedure (see Test
Method D4050) by this test method requires that the control
well and observation wells meet the requirements specified in
the following subsections.
6.2 Construction of Control Well—Installthecontrolwellin
NOTE 1—Modified from Ferris and others (6) and Heath (7).
theaquiferandequipwithapumpcapableofdischargingwater
FIG. 2 Diagram Showing Impermeable Boundary
from the well at a constant rate for the duration of the test.
Preferably, the control well should be open throughout the full
so that:
thickness of the aquifer. If the control well partially penetrates
r
i 2
u 5 u , u 5 K u (7) the aquifer, take special precautions in the placement or design
S D
i r i l r
r
r
of observation wells (see 5.2.1).
where:
6.3 Construction of Observation Wells and Piezometers—
r
Construct one or more observation wells or piezometers at
i
K 5 (8)
l
r
specified distances from the control well.
r
6.4 Location of Observation Wells and Piezometers—Wells
NOTE 1—K is a constant of proportionality between the radii, not to be
l
maybelocatedatanydistancefromthecontrolwellwithinthe
confused with hydraulic conductivity.
area of influence of pumping. However, if vertical flow
5. Significance and Use
componentsareexpectedtobesignificantnearthecontrolwell
andifpartiallypenetratingobservationwellsaretobeused,the
5.1 Assumptions:
5.1.1 The well discharges at a constant rate. observation wells should be located at a distance beyond the
effectofverticalflowcomponents.Iftheaquiferisunconfined,
5.1.2 Well is of infinitesimal diameter and is open through
the full thickness of the aquifer. constraints are imposed on the distance to partially penetrating
observation wells and on the validity of early time measure-
5.1.3 The nonleaky confined aquifer is homogeneous, iso-
tropic, and areally extensive except where limited by linear ments (see Test Method D4106).
boundaries.
NOTE 2—To ensure that the effects of the boundary may be observed
5.1.4 Discharge from the well is derived initially from
during the tests, some of the wells should be located along lines parallel
storage in the aquifer; later, movement of water may be
to the suspected boundary, no farther from the boundary than the control
induced from a constant-head boundary into the aquifer. well.
D 5270 – 96 (2002)
7. Procedure
Error less than, %: 1 2 5 10
For u smaller than: 0.03 0.05 0.1 0.15
7.1 The general procedure consists of conducting the field
procedure for withdrawal or injection wells tests (see Test
7.3.2.1 The value of u decreases as time, t, increases and
Method D4050) and analyzing the field data, as addressed in
decreases as radial distance, r, decreases. Therefore, for large
this test method.
values of t and small values of r, the terms to the right of log u
e
7.2 Analysis of the field data consists of two steps: deter-
in Eq 3 may be neglected, as recognized by Theis (1). The
mination of the properties of the aquifer and the nature and
modified Theis equation can then be written as follows:
distance to the image well from each observation well, and
Q r S
determination of the location of the boundary.
s 5 20.577216 2log (9)
S S DD
e
4pT 4Tt
7.3 Two methods of analysis can be used to determine the
from which it has been shown by Lohman (4) that:
aquifer properties and the nature and distance to the image
well. One method is based on the Theis nonequilibrium
2.3Q
T 5 (10)
method; the other method is based on the modified Theis
4pDs
nonequilibrium method.
where:
7.3.1 Theis Nonequilibrium Method—ExpressionsinEq5-8
Ds = the drawdown (measured or projected) over one log
are used to generate a family of curves of 1/u versus ( W (u)
r
cycle of time.
for values of K for recharging and discharging image wells as
l
shown in Fig. 3 (2). Table 1 gives values of W (u) versus 1/u.
8. Calculation and Interpretation of Results
This table may be used to create a table of (W (u) versus 1/u
8.1 Determine the aquifer properties and the nature and
for each value of K by picking values for W (u ) and W (u),
l r i
distance to the image well by either the Theis nonequilibrium
and computing the ( W (u) for the each value of 1/u.
method or the modified Theis method.
7.3.1.1 Transmissivity, storage coefficient, and the possible
8.1.1 Theis Nonequilibrium Method—The graphical proce-
location of one or more boundaries are calculated from
dure for solution by the Theis nonequilibrium method is based
parameters determined from the match point and a curve
ontherelationshipbetween (W(u)and s,andbetween 1/uand
selected from a family of type curves.
t/r .
7.3.2 Modified Theis Nonequilibrium Method—The sum of
8.1.1.1 Plot the log of values of (W (u) on the vertical
the terms to the right of log u in Eq 3 is not significant when
e coordinate and 1/u on the horizontal coordinate. Plot a family
u becomes small, that is, equal to or less than 0.01.
of curves for several values of K, for both recharging and
l
discharging images. This plot (see Fig. 3) is referred to as a
NOTE 3—The limiting value for u of less than 0.01 may be excessively
family of type curves. Plots of the family of type curves are
restrictive in som
...







Questions, Comments and Discussion
Ask us and Technical Secretary will try to provide an answer. You can facilitate discussion about the standard in here.