ASTM E2555-07
(Practice)Standard Practice for Factors and Procedures for Applying the MIL-STD-105 Plans in Life and Reliability Inspection
Standard Practice for Factors and Procedures for Applying the MIL-STD-105 Plans in Life and Reliability Inspection
SCOPE
1.1 This practice presents a procedure and related tables of factors for adapting Practice E 2234 (equivalent to MIL-STD-105) sampling plans to acceptance sampling inspection when the item quality of interest is life length or reliability. Factors are provided for three alternative criteria for lot evaluation: mean life, hazard rate, and reliable life. Inspection of the sample is by attributes with testing truncated at the end of some prearranged period of time. The Weibull distribution, together with the exponential distribution as a special case, is used as the underlying statistical model.
1.2 A system of units is not specified by this practice.
1.3 This standard does not purport to address all of the safety concerns, if any, associated with its use. It is the responsibility of the user of this standard to establish appropriate safety and health practices and determine the applicability of regulatory limitations prior to use.
General Information
Relations
Standards Content (Sample)
NOTICE: This standard has either been superseded and replaced by a new version or withdrawn.
Contact ASTM International (www.astm.org) for the latest information
An American National Standard
Designation:E2555–07
Standard Practice for
Factors and Procedures for Applying the MIL-STD-105 Plans
in Life and Reliability Inspection
This standard is issued under the fixed designation E2555; the number immediately following the designation indicates the year of
original adoption or, in the case of revision, the year of last revision. A number in parentheses indicates the year of last reapproval. A
superscript epsilon (´) indicates an editorial change since the last revision or reapproval.
1. Scope 3.1.2.2 Discussion—Thisdefinitionsupersedesthatgivenin
MEL-STD-105E.
1.1 This practice presents a procedure and related tables of
3.1.2.3 Discussion—A sampling plan and an AQL are
factors for adapting Practice E2234 (equivalent to MIL-STD-
chosen in accordance with the risk assumed. Use of a value of
105) sampling plans to acceptance sampling inspection when
AQL for a certain defect or group of defects indicates that the
the item quality of interest is life length or reliability. Factors
sampling plan will accept the great majority of the lots or
are provided for three alternative criteria for lot evaluation:
batches provided the process average level of percent defective
mean life, hazard rate, and reliable life. Inspection of the
(or defects per hundred units) in these lots or batches are no
sampleisbyattributeswithtestingtruncatedattheendofsome
greater than the designated value of AQL. Thus, the AQL is a
prearranged period of time. The Weibull distribution, together
designated value of percent defective (or defects per hundred
with the exponential distribution as a special case, is used as
units) for which lots will be accepted most of the time by the
the underlying statistical model.
sampling procedure being used. The sampling plans provided
1.2 A system of units is not specified by this practice.
herein are so arranged that the probability of acceptance at the
1.3 This standard does not purport to address all of the
designated AQL value depends upon the sample size, being
safety concerns, if any, associated with its use. It is the
generally higher for large samples than for small ones, for a
responsibility of the user of this standard to establish appro-
given AQL. The AQL alone does not identify the chances of
priate safety and health practices and determine the applica-
accepting or rejecting individual lots or batches but more
bility of regulatory limitations prior to use.
directly relates to what might be expected from a series of lots
2. Referenced Documents
or batches, provided the steps indicated in this refer to the
operating characteristic curve of the plan to determine the
2.1 ASTM Standards:
relative risks.
E456 Terminology Relating to Quality and Statistics
3.1.3 consumer’s risk, n—probability that a lot having
E2234 Practice for Sampling a Stream of Product by
specified rejectable quality level will be accepted under a
Attributes Indexed by AQL
defined sampling plan.
3. Terminology
3.1.4 double sampling plan, n—a multiple sampling plan in
which up to two samplings can be taken and evaluated to
3.1 Definitions:
accept or reject a lot.
3.1.1 The terminology defined inTerminology E456 applies
3.1.5 limiting quality level (LQL), n—quality level having a
to this practice unless modified herein.
specified consumer’s risk for a given sampling plan.
3.1.2 acceptance quality level (AQL), n—quality limit that
3.1.6 lot, n—a definite quantity of a product or material
is the worst tolerable process average when a continuing series
accumulated under conditions that are considered uniform for
of lots is submitted for acceptance sampling. E2234
sampling purposes.
3.1.2.1 Discussion—This term is often referred to as the
3.1.6.1 Discussion—The lot for sampling may differ from a
“acceptance quality limit.”
collection of units designated as a batch for other purposes, for
example, production, shipment, and so forth.
This practice is under the jurisdiction ofASTM Committee E11 on Quality and
3.1.7 multiple sampling plan, n—a sampling plan in which
Statistics and is the direct responsibility of Subcommittee E11.30 on Statistical
successive samples from a lot are drawn and after each sample
Quality Control.
Current edition approved March 1, 2007. Published April 2007. DOI: 10.1520/ is inspected a decision is made to accept the lot, reject the lot,
E2555-07.
or to take another sample, based on quality level of the
For referenced ASTM standards, visit the ASTM website, www.astm.org, or
combined samples.
contact ASTM Customer Service at service@astm.org. For Annual Book of ASTM
Standards volume information, refer to the standard’s Document Summary page on
the ASTM website.
Copyright © ASTM International, 100 Barr Harbor Drive, PO Box C700, West Conshohocken, PA 19428-2959, United States.
E2555–07
3.1.7.1 Discussion—When the quality is much less or much 3.2.7 Weibull distribution, n—probability distribution hav-
more than the AQL, the decision can be made on the first ing cumulative distribution:
sample, which is smaller than that of a single sampling plan
b
t – g
function F~t! 5 1 – exp – , t.g and probability density
S S D D
with equivalent acceptance quality level. For samples that are
h
closetotheAQLinquality,additionalsamplesarerequiredand
b21 b
b t – g t – g
the total sample size will be larger than the corresponding function f~t! 5 exp –
S D S S D D
h h h
single sampling plan.
3.2.7.1 Discussion—TheWeibulldistributioniswidelyused
3.1.8 sample, n—group of items, observations, test results,
for modeling product life. It can take a wide variety of shapes
or portions of material taken from a large collection of items,
andalsothecharacteristicsofothertypesofdistributionsbased
observations,testresults,orquantitiesofmaterialthatservesto
on the value of its parameters. g is called the location,
provide information that may be used as a basis for making a
minimum life, or threshold parameter and defines the lower
decision concerning the larger collection. E2234
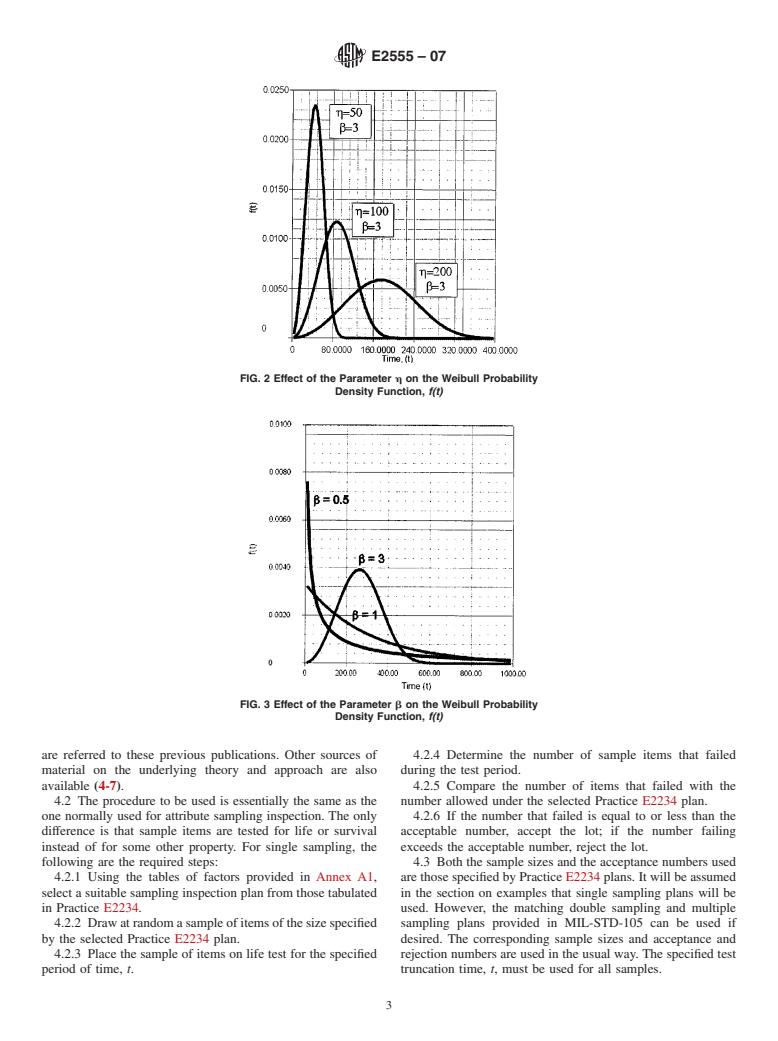
limit of the distribution (Fig. 1). h is called the scale or
3.2 Definitions of Terms Specific to This Standard:
characteristic life parameter and is equal to the 63.2 percentile
3.2.1 acceptance number, n—the maximum number of
of the distribution, minus g (Fig. 2). b is the shape parameter
failed items allowed in the sample for the lot to be accepted
(Fig. 3). The exponential distribution is the special case where
using a single or multiple sampling plan.
g = 0 and b=l.
3.2.2 hazard rate, n—differential fraction of items failing at
time t among those surviving up to time t, symbolized by h(t).
4. Significance and Use
3.2.2.1 Discussion—h(t) is also referred to as the instanta-
4.1 The procedure and tables presented in this practice are
neous failure rate at time t. It is related to the probability
based on the use of the Weibull distribution in acceptance
density and cumulative distribution functions by h(t) = f(t)/
sampling inspection. Details of this work, together with tables
(l – F(t)).
of sampling plans of other forms, have been published previ-
3.2.3 mean life, n—average time that items in the lot or
ously. See Refs (1-3). Since the basic computations required
population are expected to operate before failure.
havealreadybeenmade,ithasbeenquiteeasytoprovidethese
3.2.3.1 Discussion—Thismetricisoftenreferredtoasmean
new factors. No changes in method or details of application
time to failure (MTTF) or mean time before failure (MTBF).
have been made over those described in the publications
3.2.4 rejection number, n—the minimum number of failed
referencedabove.Forthisreason,thetextportionofthisreport
items in the sample that will cause the lot to be rejected under
has been briefly written. Readers interested in further details
a given sampling plan.
3.2.5 reliable life (r ), n—life beyond which some specified
r
proportion, r, of the items in the lot or population will survive.
3.2.6 test truncation time (t), n—amount of time sampled 3
The boldface numbers in parentheses refer to the list of references at the end of
items are allowed to be tested. this standard.
FIG. 1 Effect of the Parameter g on the Weibull Probability
Density Function, f(t)
E2555–07
FIG. 2 Effect of the Parameter h on the Weibull Probability
Density Function, f(t)
FIG. 3 Effect of the Parameter b on the Weibull Probability
Density Function, f(t)
are referred to these previous publications. Other sources of 4.2.4 Determine the number of sample items that failed
material on the underlying theory and approach are also during the test period.
available (4-7). 4.2.5 Compare the number of items that failed with the
4.2 The procedure to be used is essentially the same as the number allowed under the selected Practice E2234 plan.
one normally used for attribute sampling inspection. The only 4.2.6 If the number that failed is equal to or less than the
difference is that sample items are tested for life or survival acceptable number, accept the lot; if the number failing
instead of for some other property. For single sampling, the exceeds the acceptable number, reject the lot.
following are the required steps: 4.3 Both the sample sizes and the acceptance numbers used
4.2.1 Using the tables of factors provided in Annex A1, are those specified by Practice E2234 plans. It will be assumed
select a suitable sampling inspection plan from those tabulated in the section on examples that single sampling plans will be
in Practice E2234. used. However, the matching double sampling and multiple
4.2.2 Drawatrandomasampleofitemsofthesizespecified sampling plans provided in MIL-STD-105 can be used if
by the selected Practice E2234 plan. desired. The corresponding sample sizes and acceptance and
4.2.3 Place the sample of items on life test for the specified rejection numbers are used in the usual way. The specified test
period of time, t. truncation time, t, must be used for all samples.
E2555–07
4.4 The probability of acceptance for a lot under this terms of these ratios, the probability of acceptance will be high
procedure depends only on the probability of a sample item for lots whose mean life meets the specified requirement. The
failing before the end of the test truncation time, t. For this actualprobabilityofacceptancewillvaryfromplantoplanand
reason, the actual life at failure need not be determined; only maybereadfromtheassociatedoperatingcharacteristiccurves
thenumberofitemsfailingisofinterest.Liferequirementsand supplied in MDL-STD-105. The curves are entered by using
test time specifications need not necessarily be measured in the corresponding p’(%) value. Annex Table 1B lists 100t/µ
chronologicaltermssuchasminutesorhours.Forexample,the ratios at the LQLfor the quality level at which the consumer’s
life measure may be cycles of operation, revolutions, or miles risk is 0.10. Annex Table 1C lists corresponding 100t/µ ratios
of travel. for a consumer’s risk of 0.05.
4.5 Theunderlyinglifedistributionassumedinthisstandard 4.8.1 These ratios are to be used directly for the usual case
is the Weibull distribution (note that the exponential distribu- for which the value for the Weibull location or threshold
tion is a special case of the Weibull). The Weibull model has parameter (g) can be assumed as zero. If g is not zero but has
three parameters. One parameter is a scale or characteristic life someotherknownvalue,allthatshallbedoneistosubtractthe
parameter. For these plans and procedures, the value for this value for g from t to get t and from m to get m . These
0 0
parameter need not be known; the techniques used are inde- transformed values, t and m , are then employed in the use of
0 0
pendent of its magnitude. A second parameter is a location or the tables and for all other computations.Asolution in terms of
“guaranteedlife”parameter.Intheseplansandprocedures,itis m and t can then be converted back to actual or absolute
0 0
assumed that this parameter has a value of zero and that there values by adding the value for g to each.
is some risk of item failure right from the start of life. If this is
5. Examples, Mean Life Ratio
not the case for some applications, a simple modification in
5.1 A Practice E2234 acceptance sampling inspection plan
procedure is available. The third parameter, and the one of
is to be applied to incoming lots of product for which the mean
importance, is the shape parameter, b. The magnitude of the
item life is the property of interest.An acceptable mean life of
conversion factors used in the procedures described in this
2000 h has been specified, and under the plan, used lots with a
report depends directly on the value for this parameter. For this
mean life of this value or greater shall have a high probability
reason, the magnitude of the parameter shall be known through
of acceptance. A testing truncation time of t = 250 h has been
experience with the product or shall be estimated from past
specified. From past experience it has been determined that the
research, engineering, or inspection data. Estimation proce-
Weibull distribution can be used as a life-length model and a
dures are available and are outlined in Ref (1).
shape parameter value of 2.5 and a location or threshold
4.6 Forthecommoncaseofrandomchancefailureswiththe
parameter value of 0 can be assumed. Single sampling is to be
failurerateconstantovertime,ratherthanfailuresasaresultof
used. A sample of as many as 300 items or so can be tested at
“infant mortality” or wearout, a value of 1 for the shape
one time. An appropriate sampling inspection plan shall be
parameter shall be assumed. With this parameter value, the
selected. Also, the consumer’s risk under use of the selected
Weibull distribution reduces to the exponential. Tables of
plan shall be determined.
conversion factors are provided in Annex A1 for 15 selected
5.1.1 Computation of the 100t/µ ratio at the AQL gives
shape parameter values ranging from ⁄2 to 10, the range
100t/µ = 100 3 250/2000 = 12.5. Examination of the ratios in
commonly encountered in industrial and technical practice.
the column for a shape parameter of 2.5 in Annex Table 1A
The value 1, used for the exponential case, is included. Factors
discloses a value of 12.4 for anAQLof 0.40 in p’(%) terms.A
for other required shape parameter values within this range
plan with this AQL is accordingly to be used. Reference now
may be obtained approximately by interpolation. A more
to Practice E2234 indicates for Sample Size Code Letter M the
complete discussion of the relationship between failure pat-
sample size is 315; this value will accordingly be used.
terns and the Weibull parameters can be found in Refs (1-3).
ExaminationoftheMasterTableforNormalInsp
...







Questions, Comments and Discussion
Ask us and Technical Secretary will try to provide an answer. You can facilitate discussion about the standard in here.