ASTM D3404-91(2013)
(Guide)Standard Guide for Measuring Matric Potential in Vadose Zone Using Tensiometers
Standard Guide for Measuring Matric Potential in Vadose Zone Using Tensiometers
SIGNIFICANCE AND USE
4.1 Movement of water in the unsaturated zone is of considerable interest in studies of hazardous-waste sites (1, 2, 3, 4)2; recharge studies (5, 6); irrigation management (7, 8, 9); and civil-engineering projects (10, 11). Matric-potential data alone can be used to determine direction of flow (11) and, in some cases, quantity of water flux can be determined using multiple tensiometer installations. In theory, this technique can be applied to almost any unsaturated-flow situation whether it is recharge, discharge, lateral flow, or combinations of these situations.
4.2 If the moisture-characteristic curve is known for a soil, matric-potential data can be used to determine the approximate water content of the soil (10). The standard tensiometer is used to measure matric potential between the values of 0 and -867 cm of water; this range includes most values of saturation for many soils (12).
4.3 Tensiometers directly and effectively measure soil-water tension, but they require care and attention to detail. In particular, installation needs to establish a continuous hydraulic connection between the porous material and soil, and minimal disturbance of the natural infiltration pattern are necessary for successful installation. Avoidance of errors caused by air invasion, nonequilibrium of the instrument, or pressure-sensor inaccuracy will produce reliable values of matric potential.
4.4 Special tensiometer designs have extended the normal capabilities of tensiometers, allowing measurement in cold or remote areas, measurement of matric potential as low as -153 m of water (-15 bars), measurement at depths as deep as 6 m (recorded at land surface), and automatic measurement using as many as 22 tensiometers connected to a single pressure transducer, but these require a substantial investment of effort and money.
4.5 Pressure sensors commonly used in tensiometers include vacuum gages, mercury manometers, and pressure transducers. Only tensiometers equipped with pres...
SCOPE
1.1 This guide covers the measurement of matric potential in the vadose zone using tensiometers. The theoretical and practical considerations pertaining to successful onsite use of commercial and fabricated tensiometers are described. Measurement theory and onsite objectives are used to develop guidelines for tensiometer selection, installation, and operation.
1.2 The values stated in SI units are to be regarded as the standard. The inch-pound units given in parentheses are for information only.
1.3 This standard does not purport to address all of the safety concerns, if any, associated with its use. It is the responsibility of the user of this standard to establish appropriate safety and health practices and determine the applicability of regulatory limitations prior to use.
1.4 This guide offers an organized collection of information or a series of options and does not recommend a specific course of action. This document cannot replace education or experience and should be used in conjunction with professional judgment. Not all aspects of this guide may be applicable in all circumstances. This ASTM standard is not intended to represent or replace the standard of care by which the adequacy of a given professional service must be judged, nor should this document be applied without consideration of a project's many unique aspects. The word“ Standard” in the title of this document means only that the document has been approved through the ASTM consensus process.
General Information
Relations
Standards Content (Sample)
NOTICE: This standard has either been superseded and replaced by a new version or withdrawn.
Contact ASTM International (www.astm.org) for the latest information
Designation: D3404 − 91(Reapproved 2013)
Standard Guide for
Measuring Matric Potential in Vadose Zone Using
Tensiometers
This standard is issued under the fixed designation D3404; the number immediately following the designation indicates the year of
original adoption or, in the case of revision, the year of last revision.Anumber in parentheses indicates the year of last reapproval.A
superscript epsilon (´) indicates an editorial change since the last revision or reapproval.
1. Scope environmental changes. Parameters affecting pressure-sensor
hysteresis are temperature and measured pressure.
1.1 This guide covers the measurement of matric potential
2.1.3 precision (repeatability)—the variability among nu-
in the vadose zone using tensiometers. The theoretical and
practical considerations pertaining to successful onsite use of merous measurements of the same quantity.
commercial and fabricated tensiometers are described. Mea-
2.1.4 resolution—the smallest division of the scale used for
surement theory and onsite objectives are used to develop
a measurement, and it is a factor in determining precision and
guidelines for tensiometer selection, installation, and opera-
accuracy.
tion.
3. Summary of Guide
1.2 The values stated in SI units are to be regarded as the
standard. The inch-pound units given in parentheses are for
3.1 The measurement of matric potential in the vadose zone
information only.
can be accomplished using tensiometers that create a saturated
1.3 This standard does not purport to address all of the hydraulic link between the soil water and a pressure sensor.A
safety concerns, if any, associated with its use. It is the variety of commercial and fabricated tensiometers are com-
responsibility of the user of this standard to establish appro- monly used.Asaturated porous ceramic material that forms an
priate safety and health practices and determine the applica- interface between the soil water and bulk water inside the
bility of regulatory limitations prior to use. instrument is available in many shapes, sizes, and pore
1.4 This guide offers an organized collection of information diameters. A gage, manometer, or electronic pressure trans-
or a series of options and does not recommend a specific ducer is connected to the porous material with small- or
course of action. This document cannot replace education or large-diameter tubing. Selection of these components allows
experienceandshouldbeusedinconjunctionwithprofessional the user to optimize one or more characteristics, such as
judgment. Not all aspects of this guide may be applicable in all
accuracy, versatility, response time, durability, maintenance,
circumstances. This ASTM standard is not intended to repre- extent of data collection, and cost.
sent or replace the standard of care by which the adequacy of
a given professional service must be judged, nor should this
4. Significance and Use
document be applied without consideration of a project’s many
4.1 Movement of water in the unsaturated zone is of
unique aspects. The word“ Standard” in the title of this
considerable interest in studies of hazardous-waste sites (1, 2,
document means only that the document has been approved
3, 4) ; recharge studies (5, 6); irrigation management (7, 8, 9);
through the ASTM consensus process.
and civil-engineering projects (10, 11). Matric-potential data
alone can be used to determine direction of flow (11) and, in
2. Terminology
some cases, quantity of water flux can be determined using
2.1 Definitions of Terms Specific to This Standard:
multiple tensiometer installations. In theory, this technique can
2.1.1 accuracy of measurement—the difference between the
be applied to almost any unsaturated-flow situation whether it
value of the measurement and the true value.
is recharge, discharge, lateral flow, or combinations of these
2.1.2 hysteresis—that part of inaccuracy attributable to the
situations.
tendency of a measurement device to lag in its response to
4.2 If the moisture-characteristic curve is known for a soil,
matric-potentialdatacanbeusedtodeterminetheapproximate
watercontentofthesoil (10).Thestandardtensiometerisused
ThisguideisunderthejurisdictionofASTMCommitteeD18onSoilandRock
and is the direct responsibility of Subcommittee D18.21 on Groundwater and
Vadose Zone Investigations.
Current edition approved June 15, 2013. Published June 2013. Originally
approved in 1991. Last previous edition approved in 2004 as D3404–91 (2004). The boldface numbers in parentheses refer to a list of references at the end of
DOI: 10.1520/D3404-91R13. this standard.
Copyright © ASTM International, 100 Barr Harbor Drive, PO Box C700, West Conshohocken, PA 19428-2959. United States
D3404 − 91 (2013)
to measure matric potential between the values of 0 and-867 using Darcy’s Law. If, instead, K is known as a function of θ,
cm of water; this range includes most values of saturation for onsite moisture-content profiles (obtained, for example, from
many soils (12).
neutron-scattering methods) can be used to estimated K, and
combined with matric-potential data to estimate flux. In either
4.3 Tensiometersdirectlyandeffectivelymeasuresoil-water
case, the accuracy of the flux estimate needs to be assessed
tension, but they require care and attention to detail. In
dK dK
particular,installationneedstoestablishacontinuoushydraulic
carefully. For many porous media, and are large, within
dψ dθ
connection between the porous material and soil, and minimal
certain ranges of ψ or θ, making estimates of K particularly
disturbance of the natural infiltration pattern are necessary for
sensitive to onsite-measurement errors of ψ or θ. (Onsite-
successful installation. Avoidance of errors caused by air
measurement errors of ψ also have direct effect on π(ψ+ Z)in
invasion, nonequilibrium of the instrument, or pressure-sensor
Darcy’s Law). Other sources of error in flux estimates can
inaccuracy will produce reliable values of matric potential.
result from inaccurate data used to establish the K(ψ)or K(θ)
4.4 Special tensiometer designs have extended the normal
functions (accurate measurement of very small permeability
capabilities of tensiometers, allowing measurement in cold or
values is particularly difficult) (15); use of an analytical
remote areas, measurement of matric potential as low as-153
expression for K(ψ)or K(θ) that facilitates computer
m of water (-15 bars), measurement at depths as deep as 6 m
simulation, but only approximates the measured data; an
(recorded at land surface), and automatic measurement using
insufficient density of onsite measurements to define ad-
as many as 22 tensiometers connected to a single pressure
equately the θ or ψ profile, which can be markedly nonlinear;
transducer, but these require a substantial investment of effort
onsite soil parameters that are different from those used to
and money.
establish K(ψ)or K(θ); and invalid assumptions about the state
4.5 Pressure sensors commonly used in tensiometers in-
of onsite hysteresis. Despite the possibility of large errors,
clude vacuum gages, mercury manometers, and pressure trans-
certain flow situations occur where these errors are minimized
ducers. Only tensiometers equipped with pressure transducers
and fairly accurate estimates of flux can be obtained (6, 16) .
allow for the automated collection of large quantities of data.
Themethodhasasoundtheoreticalbasisandrefinementofthe
However,theuserneedstobeawareofthepressure-transducer
theory to match measured data markedly would improve
specifications, particularly temperature sensitivity and long-
reliability of the estimates.
term drift. Onsite measurement of known zero and “full-scale”
readings probably is the best calibration procedure; however,
5.3 The concept of fluid tension refers to the difference
onsitetemperaturemeasurementorperiodicrecalibrationinthe
between standard atmospheric pressure and the absolute fluid
laboratory may be sufficient.
pressure.Values of tension and pressure are related as follows:
5. Measurement Theory
T 5 P 2 P (2)
F AT F
5.1 In the absence of osmotic effects, unsaturated flow
where:
obeys the same laws that govern saturated flow: Darcy’s Law
M
T =
F
the tension of an elemental volume of fluid, ,
F G
and the Equation of Continuity, that were combined as the 2
LT
Richards’ Equation (13). Baver et al. (14) presents Darcy’s
Law for unsaturated flow as follows:
P = the absolute pressure of the standard atmosphere,
AT
M
q52Kπ ψ1Z (1)
~ !
, and
F G
LT
where:
L
q =
P = theabsolutepressureofthesameelementalvolumeor
F
the specific flow, ,
F G
T
M
L
K =
fluid .
F G
the unsaturated hydraulic conductivity, ,
F G LT
T
ψ = the matric potential of the soil water at a point, [L],
Soil-water tension (or soil-moisture tension) similarly is
Z = theelevationatthesamepoint,relativetosomedatum,
equaltothedifferencebetweensoil-gaspressureandsoil-water
[L], and
pressure. Thus:
−1
π = the gradient operator, [L ].
T 1P 5 P (3)
W G W
The sum of ψ+ Z commonly is referred to as the hydraulic
head.
where:
T = the tension of an elemental volume of soil water,
5.2 Unsaturatedhydraulicconductivity, K,canbeexpressed
W
M
asafunctionofeithermatricpotential, ψ,orwatercontent, θ[L
,
F G
LT
3 of water/L of soil], although both functions are affected by
hysteresis (5). If the wetting and drying limbs of the K(ψ)
P = the absolute pressure of the surrounding soil gas,
G
function are known for a soil, time series of onsite matric-
M
potential profiles can be used to determine which limb is more
, and
F G
LT
appropriate to describe the onsite K(ψ), the corresponding
values of the hydraulic-head gradient, and an estimate of flux
D3404 − 91 (2013)
P = the absolute pressure of the same elemental volume of
W
M
soil water, .
F G
LT
In this guide, for simplicity, soil-gas pressure is assumed to
be equal to 1 atm, except as noted. Various units are used to
expresstensionorpressureofsoilwater,andarerelatedtoeach
other by the equation:
1.000bar 5 100.0kPa 5 0.9869atm5 (4)
1020cmofwaterat4°C5
1020 g per cm ina standard
gravitationalfield.
Astandard gravitational field is assumed in this guide; thus,
centimetres of water at 4°C are used interchangeably with
grams per square centimetre.
5.4 The negative of soil-water tension is known formally as
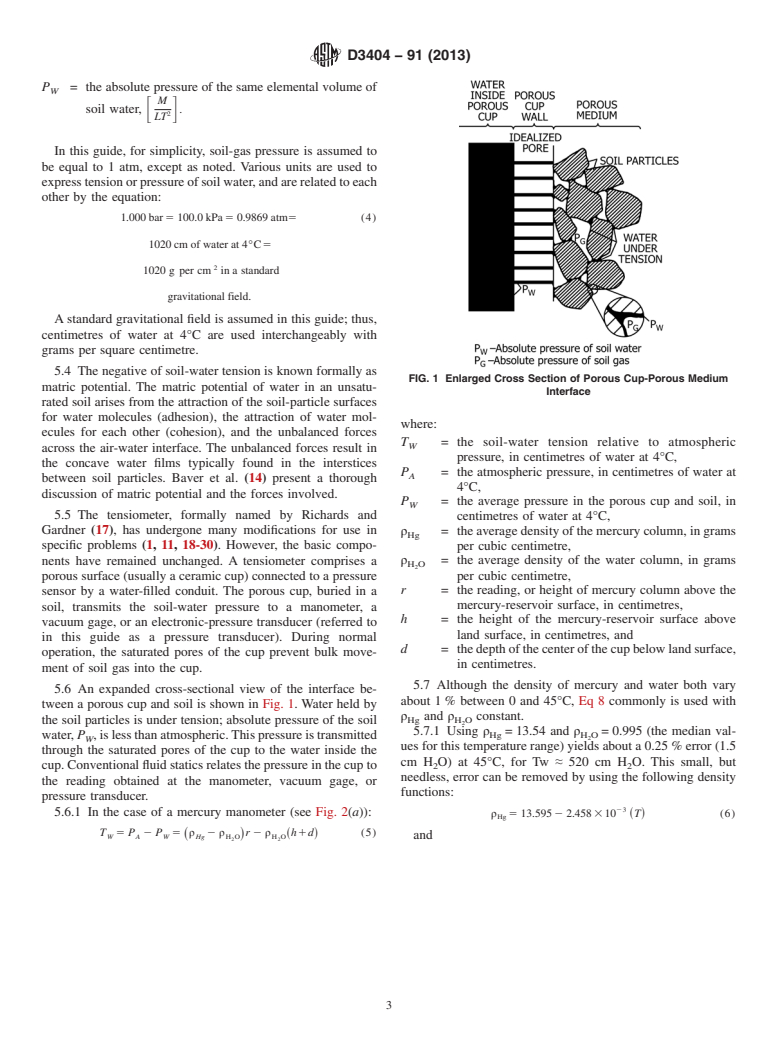
FIG. 1 Enlarged Cross Section of Porous Cup-Porous Medium
matric potential. The matric potential of water in an unsatu-
Interface
rated soil arises from the attraction of the soil-particle surfaces
for water molecules (adhesion), the attraction of water mol-
where:
ecules for each other (cohesion), and the unbalanced forces
T = the soil-water tension relative to atmospheric
W
across the air-water interface. The unbalanced forces result in
pressure, in centimetres of water at 4°C,
the concave water films typically found in the interstices
P = the atmospheric pressure, in centimetres of water at
A
between soil particles. Baver et al. (14) present a thorough
4°C,
discussion of matric potential and the forces involved.
P = the average pressure in the porous cup and soil, in
W
5.5 The tensiometer, formally named by Richards and
centimetres of water at 4°C,
Gardner (17), has undergone many modifications for use in ρ = theaveragedensityofthemercurycolumn,ingrams
Hg
specific problems (1, 11, 18-30). However, the basic compo-
per cubic centimetre,
ρ = the average density of the water column, in grams
nents have remained unchanged. A tensiometer comprises a
H O
porous surface (usually a ceramic cup) connected to a pressure per cubic centimetre,
r = the reading, or height of mercury column above the
sensor by a water-filled conduit. The porous cup, buried in a
mercury-reservoir surface, in centimetres,
soil, transmits the soil-water pressure to a manometer, a
h = the height of the mercury-reservoir surface above
vacuum gage, or an electronic-pressure transducer (referred to
land surface, in centimetres, and
in this guide as a pressure transducer). During normal
d = thedepthofthecenterofthecupbelowlandsurface,
operation, the saturated pores of the cup prevent bulk move-
in centimetres.
ment of soil gas into the cup.
5.7 Although the density of mercury and water both vary
5.6 An expanded cross-sectional view of the interface be-
about 1% between 0 and 45°C, Eq 8 commonly is used with
tween a porous cup and soil is shown in Fig. 1. Water held by
ρ and ρ constant.
Hg H O
the soil particles is under tension; absolute pressure of the soil
5.7.1 Using ρ =13.54 and ρ =0.995 (the median val-
water,P ,islessthanatmospheric.Thispressureistransmitted Hg H O
W
uesforthistemperaturerange)yieldsabouta0.25%error(1.5
through the saturated pores of the cup to the water inside the
cm H O) at 45°C, for Tw ≈ 520 cm H O. This small, but
cup.Conventionalfluidstaticsrelatesthepressureinthecupto 2 2
needless, error can be removed by using the following density
the reading obtained at the manometer, vacuum gage, or
functions:
pressure transducer.
5.6.1 In the case of a mercury manometer (see Fig. 2(a)):
ρ 5 13.595 2 2.458 310 ~T! (6)
Hg
T 5 P 2 P 5 ρ 2 ρ r 2 ρ h1d (5)
~ !
W A W ~ Hg H O! H O
and
2 2
D3404 − 91 (2013)
FIG. 3 Porous-Cup and Tube Designs
FIG. 2 Three Common Types of Tensiometers: (a) Manometer;
porous cup to its midpoint in a container of water. This setting
(b) Vacuum Gage; and (c) Pressure Transducer
isdoneatthealtitudeatwhichthetensiometerwillbeusedand
it needs to be repeated periodically after installation either by
removing the tensiometer from the soil or by unscrewing the
25 26 2
ρ 5 0.999714.879 310 ~T! 2 5.909 310 ~T! (7)
H O
gage and measuring a tension equal to that used in the original
where: ρ and ρ are as defined above, and calibration. The gage then reads directly the tension in the
Hg H O
T=average temperature of the column, in °C. porous cup. Use of a vacuum gage without an adjustable zero
5.7.2 Average temperature of the buried segment of water reading could result in inaccurate measurements because the
column can be estimated with a thermocouple or thermistor in zero reading could become negative and, therefore, would be
contact with the tubing, buried at about 45% of the depth of indeterminate.
the porous cup. Air temperature is an adequate estimate for
5.9 Pressure transducers convert pressure, or pressure
exposed segments.
difference, into a voltage (or current) signal. The pressure
5.8 Most vacuum gages used with tensiometers are gradu- transducer can be connected remotely to the porous cup with
ated in bars (and centibars) and have an adjustable zero- tubing (21, 23), attached directly to the cup (18, 31),or
reading. The zero adjustment is used to offset the effects of transportedbetweensites (23).Anabsolutepressur
...







Questions, Comments and Discussion
Ask us and Technical Secretary will try to provide an answer. You can facilitate discussion about the standard in here.