ASTM F83-71(2002)
(Practice)Standard Practice for Definition and Determination of Thermionic Constants of Electron Emitters
Standard Practice for Definition and Determination of Thermionic Constants of Electron Emitters
ABSTRACT
This practice covers the definition and interpretation of the commonly used thermionic constants of electron emitters with appended standard methods of measurement. Cathode materials shall alternatively be evaluated by relating the temperature-limited emission to fundamental properties of the emitter, particularly the work function. Comparisons are made between emitters using the thermionic constants such as the work function, emission constant, and the temperature dependence of the work function. These thermionic constants are geometry and field effects-independent, but exhibit variations under different conditions. The pertinent equations the Richardson-Dushman equation of electron emission to evaluate the effective work function, which in turn, shall be used to find the Richardson work function and the true work function. Sample computations are also detailed.
SCOPE
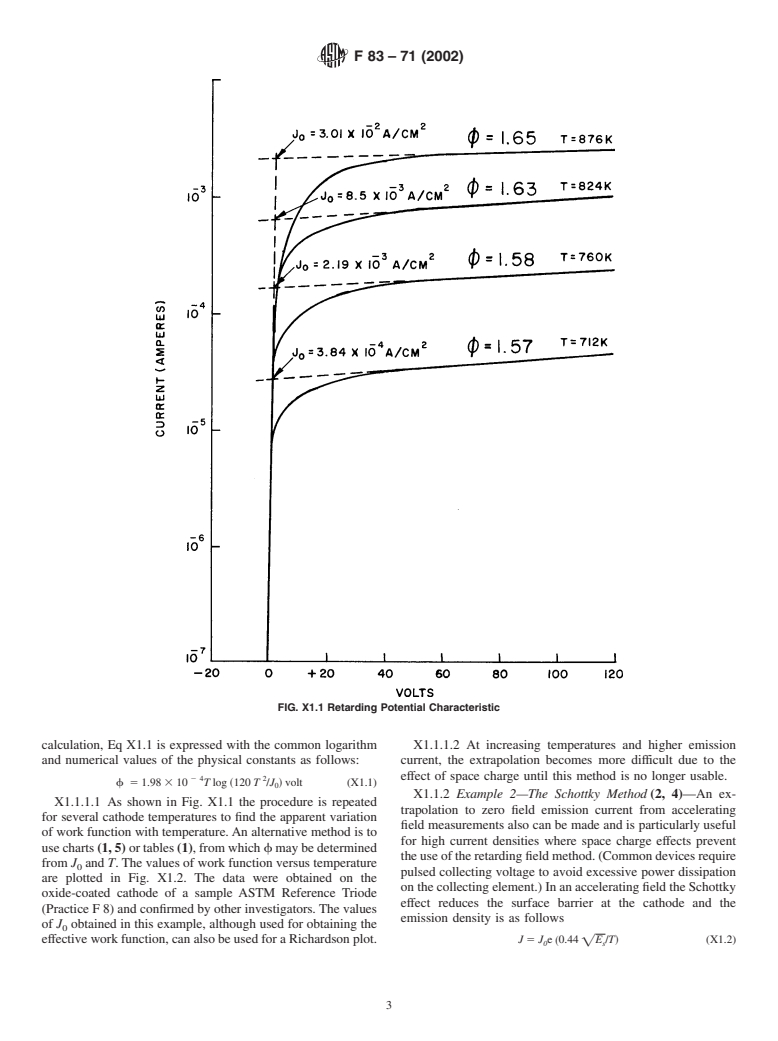
1.1 This practice covers the definition and interpretation of the commonly used thermionic constants of electron emitters (1, 2, 3), with appended standard methods of measurement.
General Information
Relations
Standards Content (Sample)
NOTICE: This standard has either been superseded and replaced by a new version or withdrawn.
Contact ASTM International (www.astm.org) for the latest information
Designation: F 83 – 71 (Reapproved 2002)
Standard Practice for
Definition and Determination of Thermionic Constants of
Electron Emitters
ThisstandardisissuedunderthefixeddesignationF83;thenumberimmediatelyfollowingthedesignationindicatestheyearoforiginal
adoption or, in the case of revision, the year of last revision.Anumber in parentheses indicates the year of last reapproval.Asuperscript
epsilon (e) indicates an editorial change since the last revision or reapproval.
INTRODUCTION
Cathode materials are often evaluated by an emission test which in some ways measures the
temperature-limited emission. A more basic approach to this problem is to relate the emission to
fundamental properties of the emitter, in particular, the work function. Comparisons are conveniently
made between emitters using the thermionic constants, that is, the work function, the emission
constant, and the temperature dependence of the work function. These quantities are independent of
geometry and field effects when properly measured. Although referred to as “constants” these
quantities show variations under different conditions. Considerable confusion exists over the
definition, interpretation, and usage of these terms and, hence, there is a need for at least a general
agreement on nomenclature.
1. Scope
where:
J = emission current density in A/cm measured under
1.1 This practice covers the definition and interpretation of
specified field conditions except zero field.
the commonly used thermionic constants of electron emitters
(J = emission current density in A/cm measured un-
(1, 2, 3), with appended standard methods of measurement.
der zero field conditions.)
2. Referenced Documents
A = the theoretical emission constant, which is calculated
from fundamental physical constants, with its value
2.1 ASTM Standards:
2 2
generally taken as 120 A/cm ·K . A more exact calcu-
F 8 Practice for Testing Electron Tube Materials Using
lation (3) gives 120.17 which is used in determining
Reference Triodes
the effective work function.
3. Terminology T = cathode temperature, K.
e = electronic charge, C.
3.1 Definitions:
e = natural logarithmic base.
3.1.1 effective work function, f—the work function ob-
k = Boltzmann’s constant.
tained by the direct substitution of experimentally determined
f = work function, V.
values of emission current density and temperature into the
The form of Eq 1 is a simplified form of the emission
Richardson-Dushman equation of electron emission of the
equationwhichassumeszeroreflectioncoefficientforelectrons
form:
with energy normally sufficient for emission at the emitter
2 2ef/kT
J 5 AT e (1)
surface. The effective work function is an empirical quantity
and represents an average of the true work function, giving the
For direct calculation of the work function, this is conve-
maximum information obtainable from a single measurement
niently put in the form:
of the thermionic emission.
f5~kT/e! ln ~AT /J! (2)
3.1.2 Richardson work function, f —the work function
usually obtained graphically from a Richardson plot, which is
a plot of ln (J/T ) versus l/T using data of emission measure-
This practice is under the jurisdiction ofASTM Committee F01 on Electronics
and is the direct responsibility of Subcommittee F01.03 on Metallic Materials.
ments at various temperatures. It is the work function obtained
Current edition approved March 31, 1971. Published May 1971. Originally
from Eq 1, with the value of A determined graphically, instead
published as F 83 – 67 T. Last previous edition F 83 – 67 T.
2 of using the theoretical value. For better visualization of the
The boldface numbers in parentheses refer to references at the end of this
Richardson plot, Eq 1 may be put in the form:
practice.
Discontinued, see 1971 Annual Book of ASTM Standards, Part 43.
Copyright © ASTM International, 100 Barr Harbor Drive, PO Box C700, West Conshohocken, PA 19428-2959, United States.
F 83 – 71 (2002)
representing the value at 0 K. The effective work function at
ln ~J/T ! 5 lnA2~e/kT!f (3)
temperature T is then equal to f + aT. Substituting this into
It can be seen (Fig. X1.4) that the Richardson work function
the equation gives:
f is obtained from the slope of the graph, and the emission
2 2~e/kT!~f
2 01aT!
J 5 AT e (4)
constant A from the intercept (l/T = 0) on the ln (J/T ) axis.
The Richardson work function is also an empirical quantity. Its
which can be put in the form:
value is found with reasonable accuracy from the graph.
2ea/k 2 2ef
0/kT
J5~Ae !T e (5)
However, large errors in the value of Amay be expected (4).
It can be seen from Eq 5 that a Richardson plot slope would
Considering only one factor, a slight inaccuracy in the mea-
determine f and a value of the emission constant e−ea/ktimes
surement of temperature introduces a large error in the value of 0
the theoretical value A. The form of Eq 4 is that used for
A. Values of A obtained on practical emitters can range from
calculation of the effective work function, with f + aT
about 0.1 to 200 A/cm ·K . 0
substitutedfortheeffectiveworkfunction f.Itcanbeseenthat
3.1.3 true work function, f—the difference between the
t
f , the value at zero temperature, is what would be obtained
Fermi energy and the surface potential energy, which is the
from a straight-line Richardson plot. These observations are
maximum potential energy of an electron at the surface of the
summarized in the following equations:
emitter, or the energy just necessary to remove an electron
f5f 1aT (6)
from the emitter. The true work function, f, is expressed in 0
t
ea/k
volts or sometimes as ef in electron volts. For a polycrystal-
t ~TheoreticalA/RichardsonA! 5 e (7)
line surface, the true work function will vary with position on
a~k/e! ln TheoreticalA/RichardsonA! (8)
~
the surface. It will also be a function of temperature. The true
The above expressions are useful in equating and interpret-
work function is primarily a theoretical concept used in
ing the effective and Richardson constants. For example, if the
analysis involving a theoretical model of the surface.
thermionicconstantsofanemitterarespecifiedbytheeffective
work function and temperature coefficient, the equivalent
4. Interpretation and Relation of Terms
Richardson work function and emission constant may be
4.1 Both the effective (f) and the Richardson (f ) work
0 calculated from the equation. Although a as determined here
functions are derived from the same basic equation for electron
serves the purpose of relating the work functions, it should not
emission. They differ in the manner of applying the equation.
be regarded as a true measure of the temperature coefficient.
The effective work function represents a direct computation
Other methods, such as the cathode cooling effect of electron
using the the
...







Questions, Comments and Discussion
Ask us and Technical Secretary will try to provide an answer. You can facilitate discussion about the standard in here.