ASTM E799-92(1998)

Standard Practice for Determining Data Criteria and Processing for Liquid Drop Size Analysis

SCOPE
1.1 This practice gives procedures for determining appropriate sample size, size class widths, characteristic drop sizes, and dispersion measure of drop size distribution. The accuracy of and correction procedures for measurements of drops using particular equipment are not part of this practice. Attention is drawn to the types of sampling (spatial, flux-sensitive, or neither) with a note on conversion required (methods not specified). The data are assumed to be counts by drop size. The drop size is assumed to be the diameter of a sphere of equivalent volume.  
1.2 The analysis applies to all liquid drop distributions except where specific restrictions are stated.

General Information

• Status: Historical
• Publication Date: 09-Jun-1998
• ICS: 07.030 - Physics. Chemistry
• Technical Committee: E29 - Particle and Spray Characterization
• Drafting Committee: E29.02 - Non-Sieving Methods

Relations

• Replaced By: ASTM E799-03 - Standard Practice for Determining Data Criteria and Processing for Liquid Drop Size Analysis
• Effective Date: 10-May-2003
• Referred By: ASTM E1620-97(2022) - Standard Terminology Relating to Liquid Particles and Atomization
• Effective Date: 10-Jun-1998
• Referred By: ASTM E1617-09(2019) - Standard Practice for Reporting Particle Size Characterization Data
• Effective Date: 10-Jun-1998
• Referred By: ASTM E2872-14(2019) - Standard Guide for Determining Cross-Section Averaged Characteristics of a Spray Using Laser-Diffraction Instruments in a Wind Tunnel Apparatus
• Effective Date: 10-Jun-1998
• Referred By: ASTM E1260-03(2020) - Standard Test Method for Determining Liquid Drop Size Characteristics in a Spray Using Optical Nonimaging Light-Scattering Instruments
• Effective Date: 10-Jun-1998
• Referred By: ASTM E1458-12(2022) - Standard Test Method for Calibration Verification of Laser Diffraction Particle Sizing Instruments Using Photomask Reticles
• Effective Date: 10-Jun-1998
• Referred By: ASTM E2798-19 - Standard Test Method for Characterization of Performance of Pesticide Spray Drift Reduction Adjuvants for Ground Application
• Effective Date: 10-Jun-1998

Standards Content (Sample)

NOTICE: This standard has either been superceded and replaced by a new version or discontinued.
Contact ASTM International (www.astm.org) for the latest information.
Designation: E 799 – 92 (Reapproved 1998)
Standard Practice for Determining
Data Criteria and Processing for Liquid Drop Size Analysis
This standard is issued under the fixed designation E 799; the number immediately following the designation indicates the year of
original adoption or, in the case of revision, the year of last revision. A number in parentheses indicates the year of last reapproval. A
superscript epsilon (e) indicates an editorial change since the last revision or reapproval.
1. Scope the swept volume. Optical scattering devices sensing continu-
ously may be difficult to identify as flux-sensitive, spatial, or
1.1 This practice gives procedures for determining appro-
neither due to instantaneous sampling of the sensors and the
priate sample size, size class widths, characteristic drop sizes,
measurable accumulation and relaxation time of the sensors.
and dispersion measure of drop size distribution. The accuracy
For widely spaced particles sampling may resemble temporal
of and correction procedures for measurements of drops using
and for closely spaced particles it may resemble spatial. A
particular equipment are not part of this practice. Attention is
flux-sensitive set of data is proportional to flux density: number
drawn to the types of sampling (spatial, flux-sensitive, or
per (unit area 3 unit time).
neither) with a note on conversion required (methods not
3.1.3 representative, adj—indicates that sufficient data have
specified). The data are assumed to be counts by drop size. The
been obtained to make the effect of random fluctuations
drop size is assumed to be the diameter of a sphere of
acceptably small. For temporal observations this requires
equivalent volume.
sufficient time duration or sufficient total of time durations. For
1.2 The analysis applies to all liquid drop distributions
spatial observations this requires a sufficient number of obser-
except where specific restrictions are stated.
vations. A spatial sample of one flash photograph is usually not
2. Referenced Documents representative since the drop population distribution fluctuates
with time. 1000 such photographs exhibiting no correlation
2.1 ASTM Standards: ASTM Standard:
with the fluctuations would most probably be representative. A
E 1296 Terminology Relating to Liquid Particle Statistics
temporal sample observed over a total of periods of time that
3. Terminology
is long compared to the time lapse between extreme fluctua-
tions would most probably be representative.
3.1 Definitions of Terms Specific to This Standard:
3.1.4 local, adj—indicates observations of a very small part
3.1.1 spatial, adj—describes the observation or measure-
(volume or area) of a larger region of concern.
ment of drops contained in a volume of space during such short
3.2 Symbols:Symbols—Representative Diameters:
intervals of time that the contents of the volume observed do
¯
3.2.1 ( D ) is defined to be such that:
not change during any single observation. Examples of spatial pq
p
sampling are single flash photography or laser holography. Any
( D
i i
~p2q!
¯
D 5 (1)
pq
sum of such photographs would also constitute spatial sam- q
( D
i i
pling. A spatial set of data is proportional to concentration:
number per unit volume.
where:
3.1.2 flux-sensitive, adj—describes the observation of mea-
¯ ¯
D = the overbar in D designates an averaging
surement of the traffic of drops through a fixed area during
process,
intervals of time. Examples of flux-sensitive sampling are the
¯
(p−q)p>q = the algebraic power of D ,
collection for a period of time on a stationary slide or in a pq
p and q = the integers 1, 2, 3 or 4,
sampling cell, or the measurement of drops passing through a
D = the diameter of the ith drop, and
i
plane (gate) with a shadowing on photodiodes or by using
p q
( = the summation of D or D , representing
i i i
capacitance changes. An example that may be characterized as
all drops in the sample.
neither flux-sensitive nor spatial is a collection on a slide
0=p and q = values 0, 1, 2, 3, or 4.
moving so that there is measurable settling of drops on the slide
( D is the total number of drops in the sample, and some
i i
in addition to the collection by the motion of the slide through
of the more common representative diameters are:
This practice is under the jurisdiction of ASTM Committee E-29 on Particle
Size Measurement and is the direct responsibility of Subcommittee E29.04 on
Liquid Particle Measurement.
Current edition approved Dec. 15, 1992. Published February 1993. Originally
published as E 799 – 81. Last previous edition E 799 – 87. This notation follows: Mugele, R.A. and Evans, H.D., “Droplet Size Distribu-
Annual Book of ASTM Standards, Vol 14.02. tion in Sprays,” Ind. Engnrg. Chem. Vol 43, No. 6 (1951), pp. 1317-1324.
Copyright © ASTM International, 100 Barr Harbor Drive, PO Box C700, West Conshohocken, PA 19428-2959, United States.
NOTICE: This standard has either been superceded and replaced by a new version or discontinued.
Contact ASTM International (www.astm.org) for the latest information.
E 799
¯ 3.2.5 D = upper-boundary diameter of drops in the kth
D = linear (arithmetic) mean diameter, kub
¯ size class.
D = surface area mean diameter,
¯
D = volume mean diameter, 3.2.6 D = lower-boundary diameter of drops in the kth
klb
¯
D = volume/surface mean diameter (Sauter), and size class.
¯
D = mean diameter over volume (De Broukere or Her-
4. Significance and Use
dan).
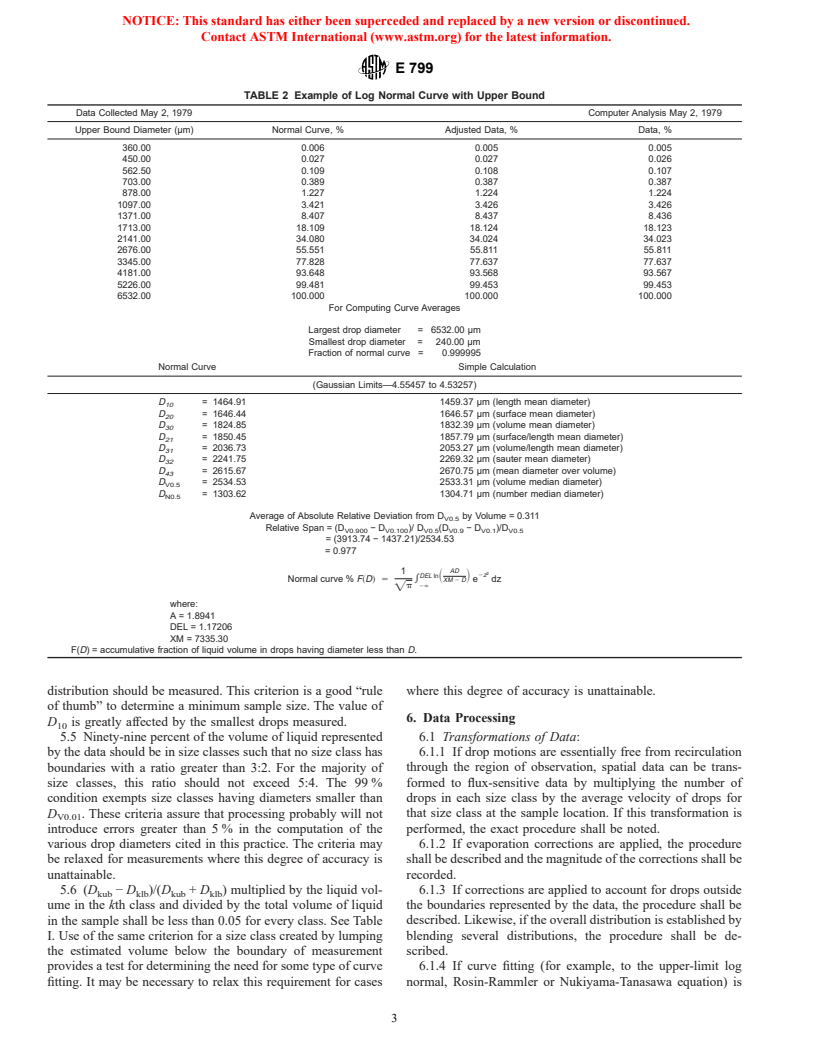
See Table 1 for numerical examples. 4.1 These criteria and procedures provide a uniform base
3.2.2 D ,D ,D , and D are diameters such that the for analysis of liquid drop data.
Nf Lf Af Vf
fraction, f, of the total number, length of diameters, surface
5. Test Data
area, and volume of drops, respectively, contain precisely all of
the drops of smaller diameter. Some examples are: 5.1 Specify the data as temporal or spatial. If the data cannot
be so specified, describe the sampling procedure. Also specify
whether the data are local (that is, in a very small section of the
D = number median diameter,
N0.5
space of liquid drop dispersion), and whether the data are
D = length median diameter,
L0.5
representative (that is, a good description of the distribution of
D = surface area median diameter,
A0.5
concern). Report the fluids, fluid properties, and pertinent
D = volume median diameter, and
V0.5
operating conditions.
D = drop diameter such that 90 % of the total liquid
V0.9
5.1.1 A graph form for reporting data is given in Fig. 1.
volume is in drops of smaller diameter.
5.2 Report the largest and smallest drops of the entire
See Table 2 for numerical examples.
sample, the number of drops in each size class, and the class
3.2.3
boundaries. Also report the sampling volume, area, and lapse
¯
log ~D ! 5 ( log ~D !/n (2)
gm i i of time, if available and applicable.
5.3 Estimate the total volume of liquid in the sample that
includes measured drops and the liquid in the sample that is not
where:
measured. (The volume outside the range of sizes permitted by
n = number of drops,
the measuring technique might be estimated by graphical
¯
D = the geometric mean diameter
gm
extrapolation of a histogram or by a curve fitting technique.)
3.2.4
5.4 The ratio of the volume of the largest drop to the total
D 5 D (3)
RR VF
volume of liquid in the sample should be less than the tolerable
fractional error in the desired representation. See Table 1. All
where:
of the drops in the sample at the large-drop end of the
f = 1 − 1/e ’ .6321
D = Rosin-Rammler Diameter fitting the Rosin-
RR
Rammler distribution factor (See Terminology
These criteria ensure that processing probably will not introduce error greater
E 1296)
than5%inthe computation of the various drop sizes used to characterize the spray.
TABLE 1 Sample Data Calculation Table
r A
Size Class Bounds No. of Sum of D in Each Size Class
i
Class Vol. % Cum. %
(Diameter Drops in
B
Width in Class by Vol.
2 3 4
D D D D
in Micrometres) Class
i i i i
3 6 9 12
240–360 120 65 19.5 3 10 5.9 3 10 1.8 3 10 1. 3 10 0.005 0.005
360–450 90 119 48.2 19.6 8.0 3 0.021 0.026
450–562.5 112.5 232 117.4 59.7 30.5 16 0.081 0.107
562.5–703 140.5 410 259.4 164.8 105.2 67 0.280 0.387
703–878 175 629 497.2 394.7 314.5 252 0.837 1.224
878–1097 219 849 838.4 831.3 827.6 827 2.202 3.426
1097–1371 274 990 1221.7 1513.7 1883.2 2352 5.010 8.436
1371–1713 342 981 1512.7 2342.1 3641.1 5683 9.687 18.123
1713–2141 428 825 1589.8 3076.1 5976.2 11657 15.900 34.023
2141–2676 535 579 1394.5 3372.5 8189.2 19965 21.788 55.811
2676–3345 669 297 894.1 2702.8 8203.5 24999 21.826 77.637
3345–4181 836 111 417.7 1578.2 5987.6 22807 15.930 93.567
4181–5226 1045 21 98.8 466.5 2212.1 10532 5.885 99.453
5226–6532 1306 1 5.9 34.7 348.5 1534 0.547 100.000
r 3 6 9 12
Totals of D in (k = 6109 8915.3 3 10 16562.6 3 10 37729.0 3 10 100695 3 10
i
¯ ¯ ¯ ¯
entire sample D = 1300 D = 1460 D = 1860 D = 2280 D = 2670
N0.5 10 21 32 43
¯ ¯
D = 1650 D = 2060
20 31
¯
D = 1830
D = 2540 Worst case class width
V0.5
348.5 669
5 0.009 Relative Span 5 D 2 D !/D 5 3900–14200/2530 5 0.98 3 0.21826 5 0.024
~ ~
V0.9 V0.5 V0.5
37729 2676 1 3345
Less than 1%, adequate sample size Adequate class sizes
A
The individual entries are the values for each k as used in 5.2.1 (E
...