ASTM E561-98

Standard Practice for R-Curve Determination

SCOPE
1.1 This practice covers the determination of resistance to fracturing of metallic materials by R-curves using either the center-cracked tension panel M(T), the compact specimen C(T), or the crack-line-wedge-loaded specimen C(W), to deliver applied stress intensity factor, K, to the material. An -curve is a continuous record of toughness development in terms of KR plotted against crack extension in the material as a crack is driven under a continuously increased stress intensity factor, .  
1.2 Materials that can be tested for -curve development are not limited by strength, thickness, or toughness, so long as specimens are of sufficient size to remain predominantly elastic throughout the duration of the test.  
1.3 Specimens of standard proportions are required, but size is variable, to be adjusted for yield strength and toughness of the materials.  
1.4 Only three of the many possible specimen types that could be used to develop -curves are covered in this practice.  
1.5 This standard does not purport to address all of the safety concerns, if any, associated with its use. It is the responsibility of the user of this standard to establish appropriate safety and health practices and determine the applicability of regulatory limitations prior to use.

General Information

• Status: Historical
• Publication Date: 09-Dec-1998
• ICS: 77.040.10 - Mechanical testing of metals
• Technical Committee: E08 - Fatigue and Fracture
• Drafting Committee: E08.07 - Fracture Mechanics

Relations

• Replaced By: ASTM E561-05 - Standard Test Method for <bdit>K-R</bdit> Curve Determination
• Effective Date: 01-Jun-2005
• Referred By: ASTM B645-21 - Standard Practice for Linear-Elastic Plane-Strain Fracture Toughness Testing of Aluminum Alloys
• Effective Date: 10-Dec-1998
• Referred By: ASTM E647-23a - Standard Test Method for Measurement of Fatigue Crack Growth Rates
• Effective Date: 10-Dec-1998
• Referred By: ASTM E1823-23 - Standard Terminology Relating to Fatigue and Fracture Testing
• Effective Date: 10-Dec-1998
• Referred By: ASTM D7779-20 - Standard Test Method for Determination of Fracture Toughness of Graphite at Ambient Temperature
• Effective Date: 10-Dec-1998
• Referred By: ASTM E2472-12(2018)e2 - Standard Test Method for Determination of Resistance to Stable Crack Extension under Low-Constraint Conditions
• Effective Date: 10-Dec-1998
• Referred By: ASTM B909-21a - Standard Guide for Plane Strain Fracture Toughness Testing of Non-Stress Relieved Aluminum Products
• Effective Date: 10-Dec-1998
• Referred By: ASTM B646-19 - Standard Practice for Fracture Toughness Testing of Aluminum Alloys
• Effective Date: 10-Dec-1998
• Referred By: ASTM E740/E740M-03(2016) - Standard Practice for Fracture Testing with Surface-Crack Tension Specimens
• Effective Date: 10-Dec-1998

Standards Content (Sample)

NOTICE: This standard has either been superseded and replaced by a new version or withdrawn.
Contact ASTM International (www.astm.org) for the latest information
Designation:E561–98
Standard Practice for
R-Curve Determination
This standard is issued under the fixed designation E561; the number immediately following the designation indicates the year of
original adoption or, in the case of revision, the year of last revision.Anumber in parentheses indicates the year of last reapproval.A
superscript epsilon (e) indicates an editorial change since the last revision or reapproval.
1. Scope 3.1.1 crack size, a (L)—a lineal measure of a principal
planar dimension of a crack. This measure is commonly used
1.1 This practice covers the determination of resistance to
in the calculation of quantities descriptive of the stress and
fracturing of metallic materials by R-curves using either the
displacement fields, and is often also termed crack length.
center-cracked tension panel M(T), the compact specimen
3.1.1.1 Discussion—In practice, the value of a is obtained
C(T), or the crack-line-wedge-loaded specimen C(W), to
from procedures for measurement of physical crack size, a ,
deliver applied stress intensity factor, K, to the material. An p
original crack size, a , and effective crack size, a , as appro-
o e
R-curve is a continuous record of toughness development in
priate to the situation being considered.
terms of K plotted against crack extension in the material as a
R
3.1.2 physical crack size, a (L)—the distance from a
p
crack is driven under a continuously increased stress intensity
reference position to the observed crack front. This distance
factor, K.
mayrepresentanaveragefromseveralmeasurementsalongthe
1.2 Materialsthatcanbetestedfor R-curvedevelopmentare
crack front. The reference position depends on the specimen
not limited by strength, thickness, or toughness, so long as
form, and it is normally taken to be either the boundary, or a
specimensareofsufficientsizetoremainpredominantlyelastic
plane containing either the load line or the centerline of a
throughout the duration of the test.
specimen or plate.
1.3 Specimensofstandardproportionsarerequired,butsize
3.1.3 original crack size, a (L)—the physical crack size at
o
is variable, to be adjusted for yield strength and toughness of
the start of testing.
the materials.
3.1.4 effective crack size, a (L)—the physical crack size
e
1.4 Only three of the many possible specimen types that
augmented for the effects of crack-tip plastic deformation.
could be used to develop R-curves are covered in this practice.
3.1.4.1 Discussion—Sometimes the effective crack size, a ,
e
1.5 This standard does not purport to address all of the
is calculated from a measured value of a physical crack size,
safety concerns, if any, associated with its use. It is the
a , plus a calculated value of a plastic-zone adjustment, r .A
p Y
responsibility of the user of this standard to establish appro-
preferred method for calculation of a compares compliance
priate safety and health practices and determine the applica- e
from the secant of a load-deflection trace with the elastic
bility of regulatory limitations prior to use.
compliance from a calibration of the specimen.
2. Referenced Documents
3.1.5 plastic-zone adjustment, r (L)—an addition to the
Y
physicalcracksizetoaccountforplastic,crack-tipdeformation
2.1 ASTM Standards:
effects on the linear-elastic stress field.
E399 Test Method for Plane-Strain Fracture Toughness of
3.1.5.1 Discussion—Commonlytheplastic-zoneadjustment
Metallic Materials
is given by:
E616 Terminology Relating to Fracture Testing
E647 Test Method for Measurement of Fatigue Crack
1 K
r 5 ,forplane2stressmode1,and
Growth Rates Y 2
2p
s
Y
3. Terminology a K
r 5 ,forplane2strainmodeI,
Y
2p
s
3.1 Definitions: Y
1 1
where a . ⁄3 to ⁄4 and s is the effective yield strength.
Y
In this practice, plane-stress mode 1 is assumed.
This practice is under the jurisdiction ofASTM Committee E-8 on Fatigue and
3.1.6 crack extension, Da (L)—an increase in crack size.
Fracture and is the direct responsibility of Subcommittee E08.07 on Linear–Elastic
3.1.6.1 Discussion—For example, Da or Da is the differ-
Fracture. p e
CurrenteditionapprovedDecember10,1998.PublishedMarch1999.Originally ence between the crack size, either a (physical crack size) or
p
published as a proposed recommended practice in 1974. Last previous edition
a (effective crack size), and a (original crack size).
e o
E561–94.
Annual Book of ASTM Standards, Vol 03.01.
Copyright © ASTM International, 100 Barr Harbor Drive, PO Box C700, West Conshohocken, PA 19428-2959, United States.
E561
−3/2
3.1.7 stress–intensity factor, K, K,K,K (FL )—the
1 2 3
magnitude of the ideal-crack-tip stress field (a stress-field
singularity) for a particular mode in a homogeneous, linear-
elastic body.
3.1.7.1 Discussion—Values of K for modes 1, 2, and 3 are
given by:
1/2
limit
K 5 @s ~2 p r! #,
1 y
r→o
1/2
limit
K 5 @t ~2 p r! #,and
2 xy
r→o
1/2
limit
K 5 @t ~2 p r! #.
3 yz
r→o
where r 5a distance directly forward from the crack tip to a location
where the significant stress is calculated.
FIG. 1 Schematic Representation of R-Curve and Applied K
3.1.7.2 Discussion—In this practice, plane-stress mode 1 is
Curves to Predict Instability;K ,P ,a , Corresponding to an
c 3 c
assumed.
Initial Crack Size, a
o
−3/2
3.1.8 crack-extension resistance, K (FL ), and G or
R R
−1
J (FL )—a measure of the resistance to crack extension
R
4.3 Methods of measuring crack growth and of making
expressedinthesameunitsasthestress-intensityfactor, K,the
plastic-zone corrections to the physical crack length are pre-
crack-extension force, G, or values of J derived using the
scribed. Expressions for the calculation of crack-extension
J-integral concept.
force are shown.
3.1.8.1 Discussion—See definition of R-curve.
3.1.9 R-curve—a plot of crack-extension resistance as a 5. Significance and Use
function of slow-stable crack extension, Da or Da .
p e 5.1 R-curves characterize the resistance to fracture of ma-
3.1.9.1 Discussion—For specimens discussed in Practice
terials during incremental slow-stable crack extension and
E561,influenceofin-planegeometryappearstobenegligible, resultfromgrowthoftheplasticzoneasthecrackextendsfrom
but R-curves normally depend upon specimen thickness and, a sharp notch. They provide a record of the toughness
for some materials, upon temperature and strain rate. development as a crack is driven stably under increasing
applied K. They are dependent upon specimen thickness,
3.1.10 crack displacement (L)—the separation vector be-
temperature, and strain rate.
tween two points (on the surfaces of a deformed crack) that
5.2 For an untested geometry, the R-curve can be matched
were coincident on the surfaces of an ideal crack in the
with the applied K curves to estimate the load necessary to
undeformed condition.
cause unstable crack propagation at K (1) . (See Fig. 1.) In
c
3.1.10.1 Discussion—In this practice, displacement, v, is
making this estimate, R-curves are regarded as though they are
total displacement as measured by clip gages or other devices
independent of starting crack length, a , and the specimen
spanning the crack. Measurement points on C(W) and C(T)
configuration in which they are developed. For a given
specimens are identified as locations V , V1, and V2.
material, material thickness, and test temperature, they appear
3.2 Definitions of Terms Specific to This Standard:
to be a function of crack extension, Da, only (2). To predict
3.2.1 plane-stress fracture toughness, K —in Practice
c crack instability in a component, the R-curve may be posi-
E561, the value of K at the instability condition determined
R tionedasinFig.1sothattheorigincoincideswiththeassumed
fromthetangencybetweentheR-curveandtheappliedKcurve
initial crack length, a . Applied K curves for a given configu-
of the specimen.
ration can be generated by assuming applied loads or stresses
3.2.1.1 Discussion—See the discussion of plane-strain frac- and calculating applied K as a function of crack length using
ture toughness in Terminology E616. the appropriate expression for K of the configuration. The
unique curve that develops tangency with the R-curve defines
3.2.2 fixed-load or fixed-displacement applied K curves—
the critical load or stress that will cause onset of unstable
curves obtained from a fracture mechanics analysis for the test
fracturing.
specimen configuration. Assume a fixed applied load or dis-
5.3 If the K-gradient (slope of the applied K curve) of the
placement, then generate a curve of K versus the crack size as
specimen chosen to develop an R-curve has negative charac-
the independent variable.
teristics (Note 1), as in the crack-line-wedge-loaded specimen
of this method, it may be possible to drive the crack until a
4. Summary of Practice
maximumorplateautoughnesslevelisreached (3, 4, 5).When
4.1 During slow-stable fracturing, the developing crack
a specimen with positive K-gradient characteristics (Note 2) is
growth resistance, K , is equal to applied K. The crack is
R
used, the extent of the R-curve which can be developed is
driven forward by increments of increased load or displace-
terminated when the crack becomes unstable.
ment. Measurements are made at each increment for calcula-
tion of K values which are individual data points lying on the
R-curve for the material.
The boldface numbers in parentheses refer to the list of references appended to
4.2 The crack starter is a low-stress-level fatigue crack. this practice.
E561
NOTE 1—Fixeddisplacementincrack-line-loadedspecimensresultina
6.3.1 Where wedge loading is used, a low-taper-angle
decrease of K with crack extension.
wedge with a polished finish and split-pin arrangement shown
NOTE 2—With load control, K usually increases with crack extension
in Fig. 3 is used. Sketches of a segmented split-pin system
and instability will occur at maximum load.
which has proved effective for maintaining the load line
independent of rotation of the specimen arms are provided in
6. Apparatus
Fig. 4. It has been found convenient to use a wedge whose
6.1 Grips and Fixtures for Middle Cracked Tension Speci-
included angle is 3°. With proper lubrication and system
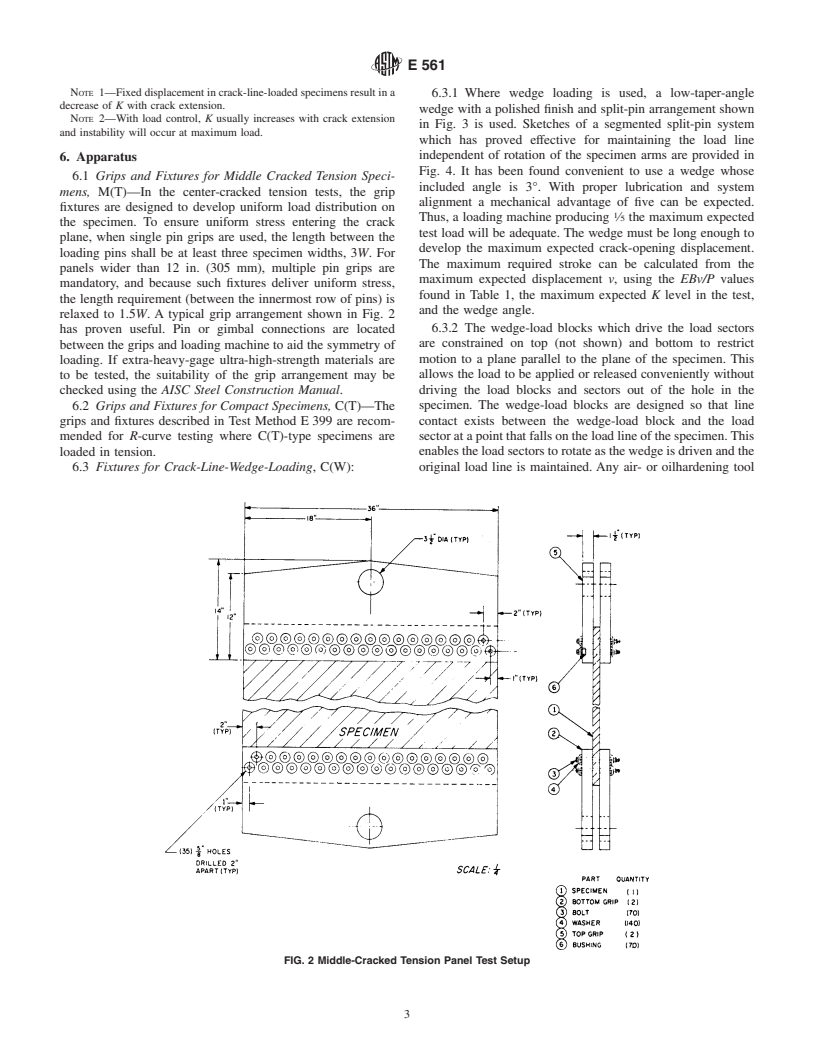
mens, M(T)—In the center-cracked tension tests, the grip
alignment a mechanical advantage of five can be expected.
fixtures are designed to develop uniform load distribution on
Thus, a loading machine producing ⁄5 the maximum expected
the specimen. To ensure uniform stress entering the crack
test load will be adequate. The wedge must be long enough to
plane, when single pin grips are used, the length between the
develop the maximum expected crack-opening displacement.
loading pins shall be at least three specimen widths, 3W. For
The maximum required stroke can be calculated from the
panels wider than 12 in. (305 mm), multiple pin grips are
maximum expected displacement v, using the EBv/P values
mandatory, and because such fixtures deliver uniform stress,
found in Table 1, the maximum expected K level in the test,
the length requirement (between the innermost row of pins) is
and the wedge angle.
relaxed to 1.5W. A typical grip arrangement shown in Fig. 2
has proven useful. Pin or gimbal connections are located 6.3.2 The wedge-load blocks which drive the load sectors
are constrained on top (not shown) and bottom to restrict
between the grips and loading machine to aid the symmetry of
loading. If extra-heavy-gage ultra-high-strength materials are motion to a plane parallel to the plane of the specimen. This
allows the load to be applied or released conveniently without
to be tested, the suitability of the grip arrangement may be
checked using the AISC Steel Construction Manual. driving the load blocks and sectors out of the hole in the
specimen. The wedge-load blocks are designed so that line
6.2 Grips and Fixtures for Compact Specimens, C(T)—The
grips and fixtures described in Test Method E399 are recom- contact exists between the wedge-load block and the load
mended for R-curve testing where C(T)-type specimens are sectoratapointthatfallsontheloadlineofthespecimen.This
loaded in tension. enablestheloadsectorstorotateasthewedgeisdrivenandthe
6.3 Fixtures for Crack-Line-Wedge-Loading, C(W): original load line is maintained. Any air- or oilhardening tool
FIG. 2 Middle-Cracked Tension Panel Test Setup
E561
TABLE 1 Dimensionless Stress Intensity Factors and Compliance in Plane Stress for the Recommended C(T) and C(W) Specimens
NOTE 1—H/W 50.6.
V at 0.1576W from loading pin centerline; see Fig. 8(a).
V at 0.25W from loading pin centerline; see Fig. 8(a).
C C C C
C(T) or C(W) C(T) EBv/P C(W) EBv/P C(T) or C(W) C(T) EBv/P C(W) EBv/P
A A
a/W a/W
1/2 B 1/2 B
KBW /P at V at V at V KBW /P at V at V at V
0 1 1 0 1 1
.350 6.392 29.89 25.82 22.83 .480 9.093 50.15 44.31 41.52
.355 6.475 30.44 26.33 23.35 .485 9.230 51.24 45.30 42.52
.360 6.558 31.01 26.85 23.88 .490 9.369 52.36 46.33 43.55
.365 6.644 31.59 27.38 24.43 .495 9.512 53.51 47.38 44.61
.370 6.730 32.20 27.94 24.99 .500 9.659 54.71 48.48 45.70
.375 6.818 32.82 28.50 25.57 .505 9.810 55.93 49.60 46.83
.380 6.906 33.45 29.08 26.16 .510 9.964 57.20 50.76 47.99
.385 6.988 34.10 29.68 26.76 .515 10.123 58.51 51.95 49.18
.390 7.090 34.77 30.29 27.38 .520 10.286 59.86 53.19 50.42
.395 7.183 35.46 30.91 28.02 .525 10.453 61.25 54.47 51.70
.400 7.279 36.16 31.55 28.67 .530 10.625 62.70 55.78 53.02
.405 7.376 36.88 32.21 29.33 .535 10.802 64.18 57.15 54.38
.410 7.475 37.62 32.88 30.01 .540 10.984 65.72 58.56 55.79
.415 7.576 38.37 33.57 30.71 .545 11.172 67.32 60.01 57.24
.420 7.678 39.15 34.27 31.42 .550 11.364 68.96 61.52 58.75
.425 7.783 39.94 34.99 32.15 .555 11.583 70.67 63.08 60.31
.430 7.890 40.75 35.73 32.90 .560 11.767 72.43 64.70 61.92
.435 7.999 41.59 36.49 33.67 .565 11.978 74.25 66.37 63.60
.440 8.110 42.44 37.27 34.45 .570 12.195 76.14 68.10 65.32
.445 8.223 43.31 38.07 35.25 .575 12.420 78.10 69.89 67.12
.450 8.340 44.21 38.89 36.08 .580 12.651 80.12 71.74 68.97
.455 8.458 45.14 39.73 36.93 .585 12.890 82.22 73.66 70.89
.460 8.580 46.08 40.60 37.80 .590 13.136 84.40 75.65 72.88
.465 8.704 47.06 41.49 38.69 .595 13.391 86.64 77.72 74.94
.470 8.830 48.06 42.40 39.61 .600 13.654 88.98 79.85 77.07
.475 8.960 49.09 43.34 40.55
A
Inverted form from Ref. (13):
2 3 4 5
a/W 5 C 1 C ~U! 1 C ~U! 1 C ~U! 1 C ~U! 1 C ~U!
0 1 2 3 4 5
1/2
U 5 1/@~EBv/P! 1 1#
C C C C C C
0 1 2 3 4 5
C(T) at V +1.0008 −4.4473 +15.400 −180.55 +870.92 −1411.3
C(T) at V +1.0010 −4.6695 +18.460 −236.82 +1214.9 −2143.6
Accuracy is a; 60.0005W
B
From Refs. (14, 15):
2 3 4
2 1 a/W 0.886 1 4.64 a/W 2 13.32 a/W 1 14.72 a/W 2 5.6 a/W
~ !@ ~ ! ~ ! ~ ! ~ ! #
1/2
KWB /P 5
3/2
~1 2 a/W!
C
From Ref. (12):
2 3 4
EBv/P 5 A 1 A ~a/W! 1 A ~a/W! 1 A ~a/W! 1 A ~a/W!
0 1 2 3
...