iTeh StandardsiTeh Standards
EURUSD
Your shopping cart is empty.
ASTM

ASTM G172-03

(Guide)

Standard Guide for Statistical Analysis of Accelerated Service Life Data

Standard Guide for Statistical Analysis of Accelerated Service Life Data

SIGNIFICANCE AND USE
The nature of accelerated service life estimation normally requires that stresses higher than those experienced during service conditions are applied to the material being evaluated. For non-constant use stress, such as experienced by time varying weather outdoors, it may in fact be useful to choose an accelerated stress fixed at a level slightly lower than (say 90 % of) the maximum experienced outdoors. By controlling all variables other than the one used for accelerating degradation, one may model the expected effect of that variable at normal, or usage conditions. If laboratory accelerated test devices are used, it is essential to provide precise control of the variables used in order to obtain useful information for service life prediction. It is assumed that the same failure mechanism operating at the higher stress is also the life determining mechanism at the usage stress. It must be noted that the validity of this assumption is crucial to the validity of the final estimate.
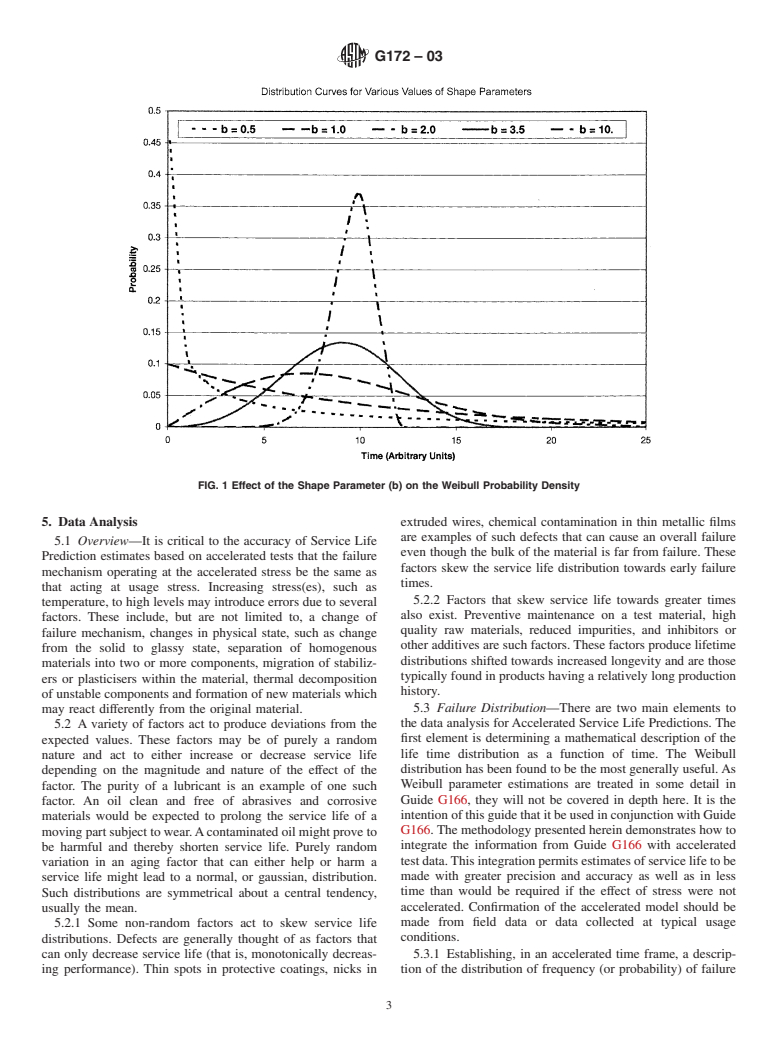
Accelerated service life test data often show different distribution shapes than many other types of data. This is due to the effects of measurement error (typically normally distributed), combined with those unique effects which skew service life data towards early failure time (infant mortality failures) or late failure times (aging or wear-out failures). Applications of the principles in this guide can be helpful in allowing investigators to interpret such data.
The choice and use of a particular acceleration model and life distribution model should be based primarily on how well it fits the data and whether it leads to reasonable projections when extrapolating beyond the range of data. Further justification for selecting models should be based on theoretical considerations.
Note 2—Accelerated service life or reliability data analysis packages are becoming more readily available in common computer software packages. This makes data reduction and analyses more directly accessible ...
SCOPE
1.1 This guide briefly presents some generally accepted methods of statistical analyses that are useful in the interpretation of accelerated service life data. It is intended to produce a common terminology as well as developing a common methodology and quantitative expressions relating to service life estimation.
1.2 This guide covers the application of the Arrhenius equation to service life data. It serves as a general model for determining rates at usage conditions, such as temperature. It serves as a general guide for determining service life distribution at usage condition. It also covers applications where more than one variable act simultaneously to affect the service life. For the purposes of this guide, the acceleration model used for multiple stress variables is the Eyring Model. This model was derived from the fundamental laws of thermodynamics and has been shown to be useful for modeling some two variable accelerated service life data. It can be extended to more than two variables.
1.3 Only those statistical methods that have found wide acceptance in service life data analyses have been considered in this guide.
1.4 The Weibull life distribution is emphasized in this guide and example calculations of situations commonly encountered in analysis of service life data are covered in detail. It is the intention of this guide that it be used in conjunction with Guide G 166.
1.5 The accuracy of the model becomes more critical as the number of variables increases and/or the extent of extrapolation from the accelerated stress levels to the usage level increases. The models and methodology used in this guide are shown for the purpose of data analysis techniques only. The fundamental requirements of proper variable selection and measurement must still be met for a meaningful model to result.

General Information

Status
Historical
Publication Date
09-Jan-2003
ICS
03.120.30 - Application of statistical methods
Technical Committee
G03 - Weathering and Durability
Drafting Committee
G03.08 - Service Life Prediction
Current Stage
Ref Project

Relations

Replaced By
ASTM G172-03(2010) - Standard Guide for Statistical Analysis of Accelerated Service Life Data
Effective Date
01-Jul-2010
Referred By
ASTM G141-09(2021) - Standard Guide for Addressing Variability in Exposure Testing of Nonmetallic Materials
Effective Date
10-Jan-2003

Buy Standard

ASTM G172-03 - Standard Guide for Statistical Analysis of Accelerated Service Life DataASTM G172-03 - Standard Guide for Statistical Analysis of Accelerated Service Life Data
PreviousNext
Guide
ASTM G172-03 - Standard Guide for Statistical Analysis of Accelerated Service Life Data
English language
12 pages
sale 15% off
Preview
sale 15% off
Preview

Standards Content (Sample)

NOTICE: This standard has either been superseded and replaced by a new version or withdrawn.
Contact ASTM International (www.astm.org) for the latest information
Designation:G172–03
Standard Guide for
1
Statistical Analysis of Accelerated Service Life Data
This standard is issued under the fixed designation G172; the number immediately following the designation indicates the year of
original adoption or, in the case of revision, the year of last revision.Anumber in parentheses indicates the year of last reapproval.A
superscript epsilon (´) indicates an editorial change since the last revision or reapproval.
1. Scope 2. Referenced Documents
2
1.1 This guide briefly presents some generally accepted 2.1 ASTM Standards:
methods of statistical analyses that are useful in the interpre- G166 Guide for Statistical Analysis of Service Life Data
tation of accelerated service life data. It is intended to produce G169 Guide forApplication of Basic Statistical Methods to
a common terminology as well as developing a common Weathering Tests
methodology and quantitative expressions relating to service
3. Terminology
life estimation.
1.2 This guide covers the application of the Arrhenius 3.1 Terms Commonly Used in Service Life Estimation:
3.1.1 accelerated stress—that experimental variable, such
equation to service life data. It serves as a general model for
determining rates at usage conditions, such as temperature. It as temperature, which is applied to the test material at levels
higher than encountered in normal use.
serves as a general guide for determining service life distribu-
tion at usage condition. It also covers applications where more 3.1.2 beginning of life—this is usually determined to be the
timeofdeliverytotheenduserorinstallationintofieldservice.
than one variable act simultaneously to affect the service life.
For the purposes of this guide, the acceleration model used for Exceptionsmayincludetimeofmanufacture,timeofrepair,or
other agreed upon time.
multiple stress variables is the Eyring Model. This model was
derivedfromthefundamentallawsofthermodynamicsandhas 3.1.3 cdf—the cumulative distribution function (cdf), de-
noted by F (t), represents the probability of failure (or the
been shown to be useful for modeling some two variable
accelerated service life data. It can be extended to more than population fraction failing) by time = (t). See 3.1.7.
3.1.4 completedata—acompletedatasetisonewhereallof
two variables.
1.3 Only those statistical methods that have found wide thespecimensplacedontestfailbytheendoftheallocatedtest
time.
acceptance in service life data analyses have been considered
3.1.5 end of life—occasionally this is simple and obvious,
in this guide.
1.4 TheWeibulllifedistributionisemphasizedinthisguide such as the breaking of a chain or burning out of a light bulb
filament. In other instances, the end of life may not be so
and example calculations of situations commonly encountered
in analysis of service life data are covered in detail. It is the catastrophicorobvious.Examplesmayincludefading,yellow-
ing, cracking, crazing, etc. Such cases need quantitative
intentionofthisguidethatitbeusedinconjunctionwithGuide
G166. measurements and agreement between evaluator and user as to
the precise definition of failure. For example, when some
1.5 The accuracy of the model becomes more critical as the
number of variables increases and/or the extent of extrapola- critical physical parameter (such as yellowing) reaches a
pre-defined level. It is also possible to model more than one
tion from the accelerated stress levels to the usage level
increases. The models and methodology used in this guide are failure mode for the same specimen (that is, the time to reach
a specified level of yellowing may be measured on the same
shown for the purpose of data analysis techniques only. The
fundamental requirements of proper variable selection and specimen that is also tested for cracking).
3.1.6 f(t)—the probability density function (pdf), equals the
measurement must still be met for a meaningful model to
result. probability of failure between any two points of time t and
(1)
t ;f(t)=dF~t! dt.Forthenormaldistribution,thepdfisthe
(2)
/
“bell shape” curve.
1
This guide is under the jurisdiction of ASTM Committee G03 on Weathering
2
and Durability and is the direct responsibility of Subcommittee G03.08 on Service For referenced ASTM standards, visit the ASTM website, www.astm.org, or
Life Prediction. contact ASTM Customer Service at service@astm.org. For Annual Book of ASTM
Current edition approved Jan. 10, 2003. Published February 2003. DOI: Standards volume information, refer to the standard’s Document Summary page on
10.1520/G0172-03. the ASTM website.
Copyright © ASTM International, 100 Barr Harbor Drive, PO Box C700, West Conshohocken, PA 19428-2959, United States.
1

---------------------- Page: 1 ----------------------
G172–03
3.1.7 F(t)—the probability that a random unit drawn from
where:
the population will fail by
...

Questions, Comments and Discussion

Ask us and Technical Secretary will try to provide an answer. You can facilitate discussion about the standard in here.

This May Also Interest You

ASTM
ASTM G172-19(Guide)
Standard Guide for Statistical Analysis of Accelerated Service Life Data

SIGNIFICANCE AND USE
4.1 The nature of accelerated service life estimation normally requires that stresses higher than those experienced during service conditions are applied to the material being evaluated. For non-constant use stress, such as experienced by time varying weather outdoors, it may in fact be useful to choose an accelerated stress fixed at a level slightly lower than (say 90 % of) the maximum experienced outdoors. By controlling all variables other than the one used for accelerating degradation, one may model the expected effect of that variable at normal, or usage conditions. If laboratory accelerated test devices are used, it is essential to provide precise control of the variables used in order to obtain useful information for service life prediction. It is assumed that the same failure mechanism operating at the higher stress is also the life determining mechanism at the usage stress. It must be noted that the validity of this assumption is crucial to the validity of the final estimate.  
4.2 Accelerated service life test data often show different distribution shapes than many other types of data. This is due to the effects of measurement error (typically normally distributed), combined with those unique effects which skew service life data towards early failure time (infant mortality failures) or late failure times (aging or wear-out failures). Applications of the principles in this guide can be helpful in allowing investigators to interpret such data.  
4.3 The choice and use of a particular acceleration model and life distribution model should be based primarily on how well it fits the data and whether it leads to reasonable projections when extrapolating beyond the range of data. Further justification for selecting models should be based on theoretical considerations.
Note 2: Accelerated service life or reliability data analysis packages are becoming more readily available in common computer software packages. This makes data reduction and analyses more direct...
SCOPE
1.1 This guide describes general statistical methods for analyses of accelerated service life data. It provides a common terminology and a common methodology for calculating a quantitative estimate of functional service life.  
1.2 This guide covers the application of two general models for determining service life distribution at usage condition. The Arrhenius model serves as a general model where a single stress variable, specifically temperature, affects the service life. It also covers the Eyring Model for applications where multiple stress variables act simultaneously to affect the service life.  
1.3 This guide emphasizes the use of the Weibull life distribution and is written to be used in combination with Guide G166.  
1.4 The uncertainty and reliability of every accelerated service life model becomes more critical as the number of stress variables increases and the extent of extrapolation from the accelerated stress levels to the usage level increases, or both. The models and methodology used in this guide are to provide examples of data analysis techniques only. The fundamental requirements of proper variable selection and measurement must still be met by the users for a meaningful model to result.  
1.5 This international standard was developed in accordance with internationally recognized principles on standardization established in the Decision on Principles for the Development of International Standards, Guides and Recommendations issued by the World Trade Organization Technical Barriers to Trade (TBT) Committee.

  • Guide
    12 pages
    English language
    sale 15% off
  • Guide
    12 pages
    English language
    sale 15% off
ASTM
ASTM E3080-23(Practice)
Standard Practice for Regression Analysis with a Single Predictor Variable

ABSTRACT
This practice covers regression analysis of a set of data to define the statistical relationship between two numerical variables for use in predicting one variable from the other. This practice is restricted in scope to consider only a single numerical response variable and a single numerical predictor variable. The objective is to obtain a regression model for use in predicting the value of the response variable Y for given values of the predictor variable X.
SIGNIFICANCE AND USE
4.1 Regression analysis is a procedure that uses data to study the statistical relationships between two or more variables (1, 2).3 This practice is restricted in scope to consider only a single numerical response variable and a single numerical predictor variable. The objective is to obtain a regression model for use in predicting the value of the response variable Y for given values of the predictor variable X.  
4.2 A regression model consists of: (1) a regression function that relates the mean values of the response variable distribution to fixed values of the predictor variable, and (2) a statistical distribution that describes the variability in the response variable values at a fixed value of the predictor variable.  
4.2.1 The regression analysis utilizes either experimental or observational data to estimate the parameters defining a regression model and their precision. Diagnostic procedures are utilized to assess the resulting model fit and can suggest other models for improved prediction performance.  
4.3 The information in this practice is arranged as follows.  
4.3.1 Section 5 gives a general outline of the steps in the regression analysis procedure. The subsequent sections cover procedures for estimation of specific regression models.  
4.3.2 Section 6 assumes a straight line relationship between the two variables. This is also known as the simple linear regression model or a first order model. This model should be used as a starting point for understanding the XY relationship and ultimately defining the best fitting model to the data.  
4.3.3 Section 7 considers a proportional relationship between the variables, where the ratio of one variable to the other is constant. The intercept is constrained to be zero. This model is useful for single point calibration, where a reference material is run periodically as a standard during routine testing to correct for drift in instrument performance over a given range of test results.  
4.3.4 Section 8 di...
SCOPE
1.1 This practice covers regression analysis of a set of data to define the statistical relationship between two numerical variables for use in predicting one variable from the other.  
1.2 The regression analysis provides graphical and calculational procedures for selecting the best statistical model that describes the relationship and for evaluation of the fit of the data to the selected model.  
1.3 The resulting regression model can be useful for developing process knowledge through description of the variable relationship, in making predictions of future values, in relating the precision of a test method to the value of the characteristic being measured, and in developing control methods for the process generating values of the variables.  
1.4 The system of units for this practice is not specified. Dimensional quantities in the practice are presented only as illustrations of calculation methods. The examples are not binding on products or test methods treated.  
1.5 This standard does not purport to address all of the safety concerns, if any, associated with its use. It is the responsibility of the user of this standard to establish appropriate safety, health, and environmental practices and determine the applicability of regulatory limitations prior to use.  
1.6 This international standard was developed in accordance with internationally recognized principles on standardization established in the Decision on Principles for the Development ...

  • Standard
    23 pages
    English language
    sale 15% off
  • Standard
    23 pages
    English language
    sale 15% off
ASTM
ASTM E2709-23(Practice)
Standard Practice for Demonstrating Capability to Comply with an Acceptance Procedure

ABSTRACT
This practice provides a general methodology for evaluating single-stage or multiple-stage acceptance procedures which involve a quality characteristic measured on a numerical scale. This methodology computes, at a prescribed confidence level, a lower bound on the probability of passing an acceptance procedure, using estimates of the parameters of the distribution of test results from a sampled population.
SIGNIFICANCE AND USE
4.1 This practice considers inspection procedures that may involve multiple-stage sampling, where at each stage one can decide to accept or to continue sampling, and the decision to reject is deferred until the last stage.  
4.1.1 At each stage there are one or more acceptance criteria on the test results; for example, limits on each individual test result, or limits on statistics based on the sample of test results, such as the average, standard deviation, or coefficient of variation (relative standard deviation).  
4.2 The methodology in this practice defines an acceptance region for a set of test results from the sampled population such that, at a prescribed confidence level, the probability that a sample from the population will pass the acceptance procedure is greater than or equal to a prespecified lower bound.  
4.2.1 Having test results fall in the acceptance region is not equivalent to passing the acceptance procedure, but provides assurance that a sample would pass the acceptance procedure with a specified probability.  
4.2.2 This information can be used for process demonstration, validation of test methods, and qualification of instruments, processes, and materials.  
4.2.3 This information can be used for lot release (acceptance), but the lower bound may be conservative in some cases.  
4.2.4 If the results are to be applied to future test results from the same process, then it is assumed that the process is stable and predictable. If this is not the case then there can be no guarantee that the probability estimates would be valid predictions of future process performance.  
4.3 This methodology was originally developed (1-4)3 for use in two specific quality characteristics of drug products in the pharmaceutical industry but will be applicable for acceptance procedures in all industries.  
4.4 Mathematical derivations would be required that are specific to the individual criteria of each test.
SCOPE
1.1 This practice provides a general methodology for evaluating single-stage or multiple-stage acceptance procedures which involve a quality characteristic measured on a numerical scale. This methodology computes, at a prescribed confidence level, a lower bound on the probability of passing an acceptance procedure, using estimates of the parameters of the distribution of test results from a sampled population.  
1.2 For a prescribed lower probability bound, the methodology can also generate an acceptance limit table, which defines a set of test method outcomes (for example, sample averages and standard deviations) that would pass the acceptance procedure at a prescribed confidence level.  
1.3 This approach may be used for demonstrating compliance with in-process, validation, or lot-release specifications.  
1.4 The system of units for this practice is not specified.  
1.5 This standard does not purport to address all of the safety concerns, if any, associated with its use. It is the responsibility of the user of this standard to establish appropriate safety, health, and environmental practices and determine the applicability of regulatory limitations prior to use.  
1.6 This international standard was developed in accordance with internationally recognized principles on standardization established in the Decision on Principles for the Development of International Standards, Guides and Recommendations issued by the World Trade Organization Technical Barriers to Trade (TBT) Committee.

  • Standard
    8 pages
    English language
    sale 15% off
  • Standard
    8 pages
    English language
    sale 15% off
ASTM
ASTM E141-10(2023)(Practice)
Standard Practice for Acceptance of Evidence Based on the Results of Probability Sampling

ABSTRACT
This practice presents rules for accepting or rejecting evidence based on a sample. Statistical evidence for this practice is in the form of an estimate of a proportion, an average, a total, or other numerical characteristic of a finite population or lot. This practice is an estimate of the result which would have been obtained by investigating the entire lot or population under the same rules and with the same care as was used for the sample. One purpose of this practice is to describe straightforward sample selection and data calculation procedures so that courts, commissions, etc. will be able to verify whether such procedures have been applied.
SIGNIFICANCE AND USE
4.1 This practice is designed to permit users of sample survey data to judge the trustworthiness of results from such surveys. Practice E105 provides a statement of principles for guidance of ASTM technical committees and others in the preparation of a sampling plan for a specific material. Guide E1402 describes the principal types of sampling designs. Practice E122 aids in deciding on the required sample size.  
4.2 Section 5 gives extended definitions of the concepts basic to survey sampling and the user should verify that such concepts were indeed used and understood by those who conducted the survey. What was the frame? How large (exactly) was the quantity N? How was the parameter θ estimated and its standard error calculated? If replicate subsamples were not used, why not? Adequate answers should be given for all questions. There are many acceptable answers to the last question.  
4.3 If the sample design was relatively simple, such as simple random or stratified, then fully valid estimates of sampling variance are easily available. If a more complex design was used then methods such as discussed in Ref (1)3 or in Guide E1402 may be acceptable. Use of replicate subsamples is the most straightforward way to estimate sampling variances when the survey design is complex.  
4.4 Once the survey procedures that were used satisfy Section 5, see if any increase in sample size is needed. The calculations for making it objectively are described in Section 6.  
4.5 Refer to Section 7 to guide in the interpretation of the uncertainty in the reported value of the parameter estimate, θ^, that is, the value of its standard error, se(θ^). The quantity se(θ^) should be reviewed to verify that the risks it entails are commensurate with the size of the sample.  
4.6 When the audit subsample shows that there was reasonable conformity with prescribed procedures and when the known instances of departures from the survey plan can be shown to have no appreciable effect on the est...
SCOPE
1.1 This practice presents rules for accepting or rejecting evidence based on a sample. Statistical evidence for this practice is in the form of an estimate of a proportion, an average, a total, or other numerical characteristic of a finite population or lot. It is an estimate of the result which would have been obtained by investigating the entire lot or population under the same rules and with the same care as was used for the sample.  
1.2 One purpose of this practice is to describe straightforward sample selection and data calculation procedures so that courts, commissions, etc. will be able to verify whether such procedures have been applied. The methods may not give least uncertainty at least cost, they should however furnish a reasonable estimate with calculable uncertainty.  
1.3 This practice is primarily intended for one-of-a-kind studies. Repetitive surveys allow estimates of sampling uncertainties to be pooled; the emphasis of this practice is on estimation of sampling uncertainty from the sample itself. The parameter of interest for this practice is effectively a constant. Thus, the principal inference is a simple point estimate to be used as if it were the unknown constant, rather than, for example, a forecast or prediction interval or distribution...

  • Standard
    6 pages
    English language
    sale 15% off
ASTM
ASTM E2862-23(Practice)
Standard Practice for Probability of Detection Analysis for Hit/Miss Data

SIGNIFICANCE AND USE
5.1 The POD analysis method described herein is based on a well-known and well established statistical regression method. It shall be used to quantify the demonstrated POD for a specific set of examination parameters and known range of discontinuity sizes under the following conditions.  
5.1.1 The initial response from a nondestructive evaluation inspection system is ultimately binary in nature (that is, hit or miss).  
5.1.2 Discontinuity size is the predictor variable and can be accurately quantified.  
5.1.3 A relationship between discontinuity size and POD exists and is best described by a generalized linear model with the appropriate link function for binary outcomes.  
5.2 This practice does not limit the use of a generalized linear model with more than one predictor variable or other types of statistical models if justified as more appropriate for the hit/miss data.  
5.3 If the initial response from a nondestructive evaluation inspection system is measurable and can be classified as a continuous variable (for example, data collected from an Eddy Current inspection system), then Practice E3023 may be more appropriate.  
5.4 Prior to performing the analysis it is assumed that the discontinuity of interest is clearly defined; the number and distribution of induced discontinuity sizes in the POD specimen set is known and well-documented; discontinuities in the POD specimen set are unobstructed; and the POD examination administration procedure (including data collection method) is well-designed, well-defined, under control, and unbiased. The analysis results are only valid if convergence is achieved and the model adequately represents the data.  
5.5 The POD analysis method described herein is consistent with the analysis method for binary data described in MIL-HDBK-1823A, and is included in several widely utilized POD software packages to perform a POD analysis on hit/miss data. It is also found in statistical software packages that have generalized line...
SCOPE
1.1 This practice covers the procedure for performing a statistical analysis on nondestructive testing hit/miss data to determine the demonstrated probability of detection (POD) for a specific set of examination parameters. Topics covered include the standard hit/miss POD curve formulation, validation techniques, and correct interpretation of results.  
1.2 The values stated in inch-pound units are to be regarded as standard. The values given in parentheses are mathematical conversions to SI units that are provided for information only and are not considered standard.  
1.3 This standard does not purport to address all of the safety concerns, if any, associated with its use. It is the responsibility of the user of this standard to establish appropriate safety, health, and environmental practices and determine the applicability of regulatory limitations prior to use.  
1.4 This international standard was developed in accordance with internationally recognized principles on standardization established in the Decision on Principles for the Development of International Standards, Guides and Recommendations issued by the World Trade Organization Technical Barriers to Trade (TBT) Committee.

  • Standard
    14 pages
    English language
    sale 15% off
  • Standard
    14 pages
    English language
    sale 15% off
ASTM
ASTM E1994-09(2023)(Practice)
Standard Practice for Use of Process Oriented AOQL and LTPD Sampling Plans

ABSTRACT
This practice is primarily a statement of principals for the guidance of ASTM technical committees and others in the use of average outgoing quality limit, AOQL, and lot tolerance percent defective, LTPD, sampling plans for determining acceptable of lots of product. Two general types of tables are given, one based on the concept of lot tolerance, LTPD, and the other on AOQL. For each of the types, tables are provided both for single sampling and for double sampling. Each of the individual tables constitutes a collection of solutions to the problem of minimizing the over-all amount of inspection.
SIGNIFICANCE AND USE
4.1 Two general types of tables (Note 1) are given, one based on the concept of lot tolerance, LTPD, and the other on AOQL. The broad conditions under which the different types have been found best adapted are indicated below.  
4.1.1 For each of the types, tables are provided both for single sampling and for double sampling. Each of the individual tables constitutes a collection of solutions to the problem of minimizing the over-all amount of inspection. Because each line in the tables covers a range of lot sizes, the AOQL values in the LTPD tables and the LTPD values in the AOQL tables are often conservative.
Note 1: Tables in Annex A1 – Annex A4 and parts of the text are reproduced by permission of John R. Wiley and Sons. More extensive tables and discussion of the methods will be found in that text.  
4.2 The sampling tables based on lot quality protection (LTPD) (the tables in Annex A1 and Annex A2) are perhaps best adapted to conditions where interest centers on each lot separately, for example, where the individual lot tends to retain its identity either from a shipment or a service standpoint. These tables have been found particularly useful in inspections made by the ultimate consumer or a purchasing agent for lots or shipments purchased more or less intermittently.  
4.3 The sampling tables based on average quality protection (AOQL) (the tables in Annex A3 and Annex A4) are especially adapted for use where interest centers on the average quality of product after inspection rather than on the quality of each individual lot and where inspection is, therefore, intended to serve, if necessary, as a partial screen for defective pieces. The latter point of view has been found particularly helpful, for example, in consumer inspections of continuing purchases of large quantities of a product and in manufacturing process inspections of parts where the inspection lots tend to lose their identity by merger in a common storeroom from which quantities are withdraw...
SCOPE
1.1 This practice is primarily a statement of principals for the guidance of ASTM technical committees and others in the use of average outgoing quality limit, AOQL, and lot tolerance percent defective, LTPD, sampling plans for determining acceptable of lots of product.  
1.2 This international standard was developed in accordance with internationally recognized principles on standardization established in the Decision on Principles for the Development of International Standards, Guides and Recommendations issued by the World Trade Organization Technical Barriers to Trade (TBT) Committee.

  • Standard
    23 pages
    English language
    sale 15% off
ASTM
ASTM E1970-23(Practice)
Standard Practice for Statistical Treatment of Thermoanalytical Data

SIGNIFICANCE AND USE
5.1 The standard deviation, or one of its derivatives, such as relative standard deviation or pooled standard deviation, derived from this practice, provides an estimate of precision in a measured value. Such results are ordinarily expressed as the mean value ± the standard deviation, that is, X ± s.  
5.2 If the measured values are, in the statistical sense, “normally” distributed about their mean, then the meaning of the standard deviation is that there is a 67 % chance, that is 2 in 3, that a given value will lie within the range of ± one standard deviation of the mean value. Similarly, there is a 95 % chance, that is 19 in 20, that a given value will lie within the range of ± two standard deviations of the mean. The two standard deviation range is sometimes used as a test for outlying measurements.  
5.3 The calculation of precision in the slope and intercept of a line, derived from experimental data, commonly is required in the determination of kinetic parameters, vapor pressure or enthalpy of vaporization. This practice describes how to obtain these and other statistically derived values associated with measurements by thermal analysis.
SCOPE
1.1 This practice details the statistical data treatment used in some thermal analysis methods.  
1.2 The method describes the commonly encountered statistical tools of the mean, standard derivation, relative standard deviation, pooled standard deviation, pooled relative standard deviation, the best fit to a (linear regression of a) straight line (or plane), and propagation of uncertainties for all calculations encountered in thermal analysis methods (see Practice E2586).  
1.3 Some thermal analysis methods derive the analytical value from the slope or intercept of a linear regression straight line (or plane) assigned to three or more sets of data pairs. Such methods may require an estimation of the precision in the determined slope or intercept. The determination of this precision is not a common statistical tool. This practice details the process for obtaining such information about precision.  
1.4 This standard does not purport to address all of the safety concerns, if any, associated with its use. It is the responsibility of the user of this standard to establish appropriate safety, health, and environmental practices and determine the applicability of regulatory limitations prior to use.  
1.5 This international standard was developed in accordance with internationally recognized principles on standardization established in the Decision on Principles for the Development of International Standards, Guides and Recommendations issued by the World Trade Organization Technical Barriers to Trade (TBT) Committee.

  • Standard
    5 pages
    English language
    sale 15% off
  • Standard
    5 pages
    English language
    sale 15% off
ASTM
ASTM E2334-09(2023)(Practice)
Standard Practice for Setting an Upper Confidence Bound for a Fraction or Number of Non-Conforming items, or a Rate of Occurrence for Non-Conformities, Using Attribute Data, When There is a Zero Response in the Sample

ABSTRACT
This practice presents methodology for the setting of an upper confidence bound regarding an unknown fraction or quantity non-conforming, or a rate of occurrence for nonconformities, in cases where the method of attributes is used and there is a zero response in a sample. Three cases are considered. In Case 1, the sample is selected from a process or a very large population of interest. In Case 2, a sample of n items is selected at random from a finite lot of N items. In Case 3, there is a process, but the output is a continuum, such as area (for example, a roll of paper or other material, a field of crop), volume (for example, a volume of liquid or gas), or time (for example, hours, days, quarterly, etc.) The sample size is defined as that portion of the �continuum� sampled, and the defined attribute may occur any number of times over the sampled portion.
SIGNIFICANCE AND USE
4.1 In Case 1, the sample is selected from a process or a very large population of interest. The population is essentially unlimited, and each item either has or has not the defined attribute. The population (process) has an unknown fraction of items p (long run average process non-conforming) having the attribute. The sample is a group of n discrete items selected at random from the process or population under consideration, and the attribute is not exhibited in the sample. The objective is to determine an upper confidence bound, pu, for the unknown fraction p whereby one can claim that p ≤ pu with some confidence coefficient (probability) C. The binomial distribution is the sampling distribution in this case.  
4.2 In Case 2, a sample of n items is selected at random from a finite lot of N items. Like Case 1, each item either has or has not the defined attribute, and the population has an unknown number, D, of items having the attribute. The sample does not exhibit the attribute. The objective is to determine an upper confidence bound, Du, for the unknown number D, whereby one can claim that D ≤ Du with some confidence coefficient (probability) C. The hypergeometric distribution is the sampling distribution in this case.  
4.3 In Case 3, there is a process, but the output is a continuum, such as area (for example, a roll of paper or other material, a field of crop), volume (for example, a volume of liquid or gas), or time (for example, hours, days, quarterly, etc.) The sample size is defined as that portion of the “continuum” sampled, and the defined attribute may occur any number of times over the sampled portion. There is an unknown average rate of occurrence, λ, for the defined attribute over the sampled interval of the continuum that is of interest. The sample does not exhibit the attribute. For a roll of paper, this might be blemishes per 100 ft2; for a volume of liquid, microbes per cubic litre; for a field of crop, spores per acre; for a time interval, ca...
SCOPE
1.1 This practice presents methodology for the setting of an upper confidence bound regarding a unknown fraction or quantity non-conforming, or a rate of occurrence for nonconformities, in cases where the method of attributes is used and there is a zero response in a sample. Three cases are considered.  
1.1.1 The sample is selected from a process or a very large population of discrete items, and the number of non-conforming items in the sample is zero.  
1.1.2 A sample of items is selected at random from a finite lot of discrete items, and the number of non-conforming items in the sample is zero.  
1.1.3 The sample is a portion of a continuum (time, space, volume, area, etc.) and the number of non-conformities in the sample is zero.  
1.2 Allowance is made for misclassification error in this practice, but only when misclassification rates are well understood or known and can be approximated numerically.  
1.3 The values stated in inch-pound units are to be regarded as standard. No other units of measurement are included in this standard.  
1.4 This ...

  • Standard
    10 pages
    English language
    sale 15% off
ASTM
ASTM D7915-22(Practice)
Standard Practice for Application of Generalized Extreme Studentized Deviate (GESD) Technique to Simultaneously Identify Multiple Outliers in a Data Set

SIGNIFICANCE AND USE
3.1 The GESD procedure can be used to simultaneously identify up to a pre-determined number of outliers (r) in a data set, without having to pre-examine the data set and make a priori decisions as to the location and number of potential outliers.  
3.2 The GESD procedure is robust to masking. Masking describes the phenomenon where the existence of multiple outliers can prevent an outlier identification procedure from declaring any of the observations in a data set to be outliers.  
3.3 The GESD procedure is automation-friendly, and hence can easily be programmed as automated computer algorithms.
SCOPE
1.1 This practice provides a step by step procedure for the application of the Generalized Extreme Studentized Deviate (GESD) Many-Outlier Procedure to simultaneously identify multiple outliers in a data set. (See Bibliography.)  
1.2 This practice is applicable to a data set comprising observations that is represented on a continuous numerical scale.  
1.3 This practice is applicable to a data set comprising a minimum of six observations.  
1.4 This practice is applicable to a data set where the normal (Gaussian) model is reasonably adequate for the distributional representation of the observations in the data set.  
1.5 The probability of false identification of outliers associated with the decision criteria set by this practice is 0.01.  
1.6 It is recommended that the execution of this practice be conducted under the guidance of personnel familiar with the statistical principles and assumptions associated with the GESD technique.  
1.7 This standard does not purport to address all of the safety concerns, if any, associated with its use. It is the responsibility of the user of this standard to establish appropriate safety, health, and environmental practices and determine the applicability of regulatory limitations prior to use.  
1.8 This international standard was developed in accordance with internationally recognized principles on standardization established in the Decision on Principles for the Development of International Standards, Guides and Recommendations issued by the World Trade Organization Technical Barriers to Trade (TBT) Committee.

  • Standard
    6 pages
    English language
    sale 15% off
  • Standard
    6 pages
    English language
    sale 15% off
ASTM
ASTM E122-17(2022)(Practice)
Standard Practice for Calculating Sample Size to Estimate, With Specified Precision, the Average for a Characteristic of a Lot or Process

ABSTRACT
This practice covers simple methods for calculating how many units to include in a random sample in order to estimate with a specified precision, a measure of quality for all the units of a lot of material or produced by a process. It also treats the common situation where the sampling units can be considered to exhibit a single source of variability; it does not treat multi-level sources of variability.
SIGNIFICANCE AND USE
4.1 This practice is intended for use in determining the sample size required to estimate, with specified precision, a measure of quality of a lot or process. The practice applies when quality is expressed as either the lot average for a given property, or as the lot fraction not conforming to prescribed standards. The level of a characteristic may often be taken as an indication of the quality of a material. If so, an estimate of the average value of that characteristic or of the fraction of the observed values that do not conform to a specification for that characteristic becomes a measure of quality with respect to that characteristic. This practice is intended for use in determining the sample size required to estimate, with specified precision, such a measure of the quality of a lot or process either as an average value or as a fraction not conforming to a specified value.
SCOPE
1.1 This practice covers simple methods for calculating how many units to include in a random sample in order to estimate with a specified precision, a measure of quality for all the units of a lot of material, or produced by a process. This practice will clearly indicate the sample size required to estimate the average value of some property or the fraction of nonconforming items produced by a production process during the time interval covered by the random sample. If the process is not in a state of statistical control, the result will not have predictive value for immediate (future) production. The practice treats the common situation where the sampling units can be considered to exhibit a single (overall) source of variability; it does not treat multi-level sources of variability.  
1.2 The system of units for this standard is not specified. Dimensional quantities in the standard are presented only as illustrations of calculation methods. The examples are not binding on products or test methods treated.  
1.3 This international standard was developed in accordance with internationally recognized principles on standardization established in the Decision on Principles for the Development of International Standards, Guides and Recommendations issued by the World Trade Organization Technical Barriers to Trade (TBT) Committee.

  • Standard
    5 pages
    English language
    sale 15% off