ASTM E598-96

Standard Test Method for Measuring Extreme Heat-Transfer Rates from High-Energy Environments Using a Transient, Null-Point Calorimeter

SCOPE
1.1 This test method covers the measurement of the heat-transfer rate or the heat flux to the surface of a solid body (test sample) using the measured transient temperature rise of a thermocouple located at the null point of a calorimeter that is installed in the body and is configured to simulate a semi-infinite solid. By definition the null point is a unique position on the axial centerline of a disturbed body which experiences the same transient temperature history as that on the surface of a solid body in the absence of the physical disturbance (hole) for the same heat-flux input.
1.2 Null-point calorimeters have been used to measure high convective or radiant heat-transfer rates to bodies immersed in both flowing and static environments of air, nitrogen, carbon dioxide, helium, hydrogen, and mixtures of these and other gases. Flow velocities have ranged from zero (static) through subsonic to hypersonic, total flow enthalpies from 1.16 to greater than 4.65 x 101 MJ/kg (5 x 10 2 to greater than 2 x 104 Btu/lb.), and body pressures from 105 to greater than 1.5 x 10 7 Pa (atmospheric to greater than 1.5 x 10 2 atm). Measured heat-transfer rates have ranged from 5.68 to 2.84 x 10 2 MW/m2 (5 x 102 to 2.5 104 Btu/ft2-sec).
1.3 The most common use of null-point calorimeters is to measure heat-transfer rates at the stagnation point of a solid body that is immersed in a high pressure, high enthalpy flowing gas stream, with the body axis usually oriented parallel to the flow axis (zero angle-of-attack). Use of null-point calorimeters at off-stagnation point locations and for angle-of-attack testing may pose special problems of calorimeter design and data interpretation.
1.4 This standard does not purport to address all of the safety concerns, if any, associated with its use. It is the responsibility of the user of this standard to establish appropriate safety and health practices and determine the applicability of regulatory limitations prior to use.

General Information

• Status: Historical
• Publication Date: 09-Oct-1996
• Technical Committee: E21 - Space Simulation and Applications of Space Technology
• Drafting Committee: E21.08 - Thermal Protection

Relations

• Replaced By: ASTM E598-96(2002) - Standard Test Method for Measuring Extreme Heat-Transfer Rates from High-Energy Environments Using a Transient, Null-Point Calorimeter
• Effective Date: 10-Oct-1996

Standards Content (Sample)

NOTICE: This standard has either been superseded and replaced by a new version or discontinued.
Contact ASTM International (www.astm.org) for the latest information.
Designation: E 598 – 96
Standard Test Method for
Measuring Extreme Heat-Transfer Rates from High-Energy
Environments Using a Transient, Null-Point Calorimeter
This standard is issued under the fixed designation E 598; the number immediately following the designation indicates the year of
original adoption or, in the case of revision, the year of last revision. A number in parentheses indicates the year of last reapproval. A
superscript epsilon (e) indicates an editorial change since the last revision or reapproval.
1. Scope Water-Cooled Calorimeter
E 511 Test Method for Measuring Heat Flux Using a
1.1 This test method covers the measurement of the heat-
Copper-Constantan Circular Foil, Heat-Flux Gage
transfer rate or the heat flux to the surface of a solid body (test
sample) using the measured transient temperature rise of a
3. Terminology
thermocouple located at the null point of a calorimeter that is
3.1 Symbols:
installed in the body and is configured to simulate a semi-
infinite solid. By definition the null point is a unique position
on the axial centerline of a disturbed body which experiences
a 5 Radius of null-point cavity, m (in.)
the same transient temperature history as that on the surface of
b 5 Distance from front surface of null-point calorimeter
a solid body in the absence of the physical disturbance (hole)
to the null-point cavity, m (in.)
for the same heat-flux input.
C 5 Specific heat capacity, J/kg–K (Btu/lb-°F)
p
1.2 Null-point calorimeters have been used to measure high
d 5 Diameter of null-point cavity, m (in.)
convective or radiant heat-transfer rates to bodies immersed in
k 5 Thermal conductivity, W/m–K (Btu/in.-sec-°F)
both flowing and static environments of air, nitrogen, carbon L 5 Length of null-point calorimeter, m (in.)
q 5 Calculated or measured heat flux or heat-transfer-rate,
dioxide, helium, hydrogen, and mixtures of these and other
2 2
gases. Flow velocities have ranged from zero (static) through W/m (Btu/ft -sec)
q 5 Constant heat flux or heat-transfer-rate, W/m (Btu/
subsonic to hypersonic, total flow enthalpies from 1.16 to
1 2
ft -sec)
greater than 4.65 3 10 MJ/kg (5 3 10 to greater than
4 5
R 5 Radial distance from axial centerline of TRAX ana-
2 3 10 Btu/lb.), and body pressures from 10 to greater than
7 2
lytical model, m (in.)
1.5 3 10 Pa (atmospheric to greater than 1.5 3 10 atm).
r 5 Radial distance from axial centerline of null-point
Measured heat-transfer rates have ranged from 5.68 to
2 2 2 4 2
cavity, m (in.)
2.84 3 10 MW/m (5 3 10 to 2.5 3 10 Btu/ft -sec).
T 5 Temperature, K (°F)
1.3 The most common use of null-point calorimeters is to
T 5 Temperature on axial centerline of null point, K (°F)
b
measure heat-transfer rates at the stagnation point of a solid
T 5 Temperature on surface of null-point calorimeter, K
s
body that is immersed in a high pressure, high enthalpy flowing
(°F)
gas stream, with the body axis usually oriented parallel to the
t 5 Time, sec
flow axis (zero angle-of-attack). Use of null-point calorimeters
Z 5 Distance in axial direction of TRAX analytical model,
at off-stagnation point locations and for angle-of-attack testing
m (in.)
may pose special problems of calorimeter design and data 2 2
a5 Thermal diffusivity, m /sec (in. /sec)
3 3
interpretation.
r5 Density, kg/m (lb/in. )
1.4 This standard does not purport to address all of the
safety concerns, if any, associated with its use. It is the 4. History of Test Method
responsibility of the user of this standard to establish appro-
4.1 From literature reviews it appears that Masters and Stein
priate safety and health practices and determine the applica-
(1) were the first to document the results of an analytical study
bility of regulatory limitations prior to use.
of the temperature effects of axial cavities drilled from the
backside of a wall which is heated on the front surface (see Fig.
2. Referenced Documents
1). These investigators were primarily concerned with the
2.1 ASTM Standards:
deviation of the temperature measured in the bottom of the
E 422 Test Method for Measuring Heat Flux Using a
cavity from the undisturbed temperature on the heated surface.
Since they were not in possession of either the computing
This test method is under the jurisdiction of ASTM Committee E-21 on Space
Simulation and Applications of Space Technology and is the direct responsibility of
Subcommittee E21.08 on Thermal Protection. Annual Book of ASTM Standards, Vol 15.03.
Current edition approved Oct. 10, 1996. Published December 1996. Originally The boldface numbers in parentheses refer to the list of references at the end of
e1
published as E 598 – 77. Last previous edition E 598 – 77 (1990). this test method.
Copyright © ASTM, 100 Barr Harbor Drive, West Conshohocken, PA 19428-2959, United States.
E 598
NOTE 1—1-T (0,t) 5 Surface temperature (x 5 0) of a solid, semi-infinite slab at some time, t.
s
NOTE 2—2-T (0,b,t) 5 Temperature at r 5 0, x 5 b of a slab with a cylindrical cavity at some time, t, heat flux, q, the same in both cases.
b
FIG. 1 Semi-infinite Slab with Cylindrical Cavity
power or the numerical heat conduction codes now available to 4.3 Howey and DeCristina (3) were the first to perform an
the analyst, Masters and Stein performed a rigorous math-
actual thermal analysis of this measurement concept. Although
ematical treatment of the deviation of the transient tempera- the explanation of modeling techniques is somewhat ambigu-
ture, T , on the bottom centerline of the cavity of radius, a, and
ous in their paper, it is obvious that they used a finite element,
b
thickness, b, from the surface temperature T . The results of two dimensional axisymmetric model to produce temperature
s
Masters and Stein indicated that the error in temperature
profiles in a geometry simulating the null-point calorimeter.
measurement on the bottom centerline of the cavity would Temperature histories at time intervals down to 0.010 sec were
decrease with increasing values of a/b and also decrease with
obtained for a high heat-flux level on the surface of the
increasing values of the dimensionless time, at/b , where a is
analytical model. Although the analytical results are not
the thermal diffusity of the wall material. They also concluded
presented in a format which would help the user/designer
that the most important factor in the error in temperature
optimize the sensor design, the authors did make significant
measurement was the ratio a/b and the error was independent
general conclusions about null point calorimeters. These in-
of the level of heat flux. The conclusions of Masters and Stein
clude: 1) “., thermocouple outputs can yield deceivingly fast
may appear to be somewhat elementary compared with our
response rates and erroneously high heating rates ( + 18 %)
knowledge of the null-point concept today. However, the
when misused in inverse one-dimensional conduction solu-
identification and documentation of the measurement concept
tions.” 2) “The prime reason for holding the thermocouple
was a major step in leading others to adapt this concept to the
depth at R/E 5 1.1 is to maximize thermocouple response at
transient measurement of high heat fluxes in ground test
high heating rates for the minimum cavity depth.” (Note: R
facilities.
and E as used by Howey and DeChristina are the same terms
4.2 Beck and Hurwicz (2) expanded the analysis of Masters
as a and b which are defined in 4.1 and are used throughout this
and Stein to include steady-state solutions and were the first to
document.) 3) A finite length null-point calorimeter body may
label the method of measurement “the null-point concept.”
be considered semi-infinite for:
They effectively used a digital computer to generate relatively
~at!
large quantities of analytical data from numerical methods. # 0.3
L
Beck and Hurwicz computed errors due to relatively large
thermocouple wires in the axial cavity and were able to suggest 4.4 Powers, Kennedy, and Rindal (4 and 5) were the first to
that the optimum placement of the thermocouple in the cavity document using null point calorimeters in the swept mode.
occurred when the ratio a/b was equal to 1.1. However, their This method which is now used in almost all arc facilities has
analysis like that of Masters and Stein was only concerned with the advantages of 1) measuring the radial distributions across
the deviation of the temperature in the axial cavity and did not the arc jet, and 2) preserving the probe/sensor structural
address the error in measured heat flux. integrity for repeated measurements. This technique involves
E 598
sweeping the probe/sensor through the arc-heated flow field at sophisticated heat conduction computer codes as well as an
a rate slow enough to allow the sensor to make accurate established numerical inverse heat conduction equation (6), the
measurements, yet fast enough to prevent model ablation. error in indicated heat flux is shown to be considerably higher
4.4.1 Following the pattern of Howey and DiCristina, Pow- than 20 % and is highly time dependent.
ers et. al. stressed the importance of performing thermal 4.5 The latest and most comprehensive thermal analysis of
analyses to “characterize the response of a typical real null the null-point calorimeter concept was performed by Kidd and
point calorimeter to individually assess a variety of potential documented in Refs (6 and 7). This analytical work was
errors, .”. Powers et. al. complain that Howey & DiCristina accomplished by using a finite element axisymmetric heat
“. report substantial errors in some cases, but present no conduction code (7). The finite element model simulating the
generalized results or design guide lines.” They state concern- null-point calorimeter system is comprised of 793 finite ele-
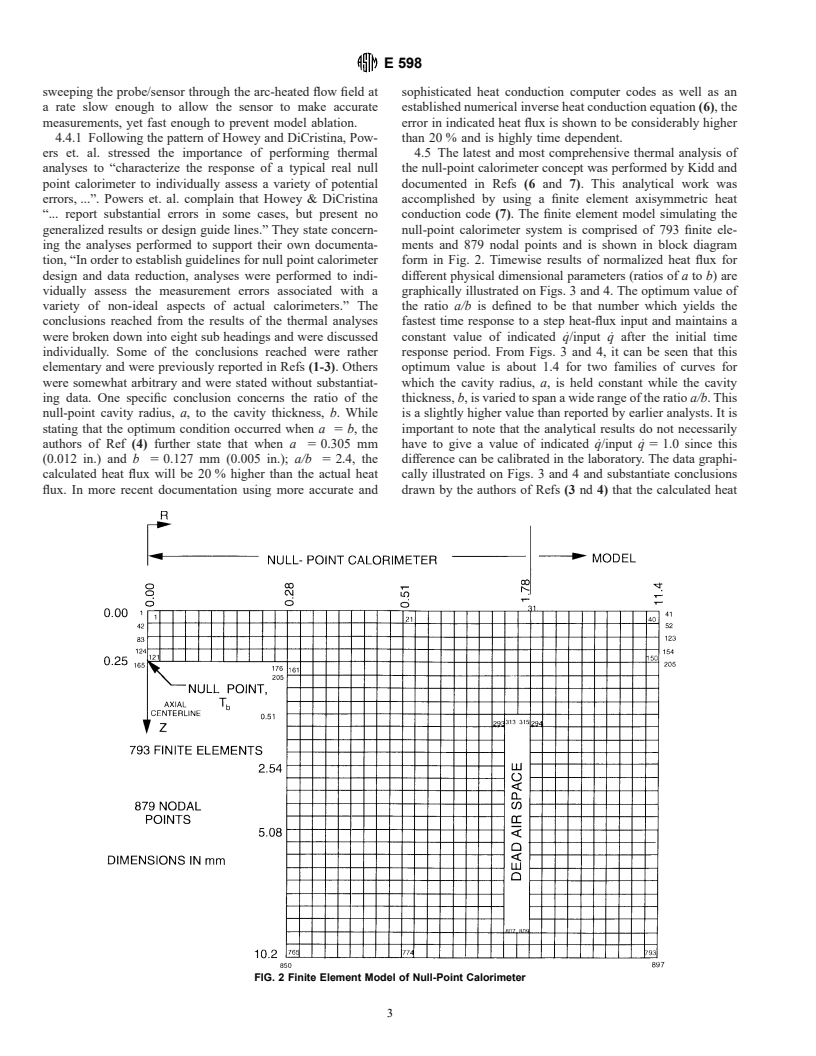
ing the analyses performed to support their own documenta- ments and 879 nodal points and is shown in block diagram
tion, “In order to establish guidelines for null point calorimeter form in Fig. 2. Timewise results of normalized heat flux for
design and data reduction, analyses were performed to indi- different physical dimensional parameters (ratios of a to b) are
vidually assess the measurement errors associated with a graphically illustrated on Figs. 3 and 4. The optimum value of
variety of non-ideal aspects of actual calorimeters.” The the ratio a/b is defined to be that number which yields the
conclusions reached from the results of the thermal analyses fastest time response to a step heat-flux input and maintains a
were broken down into eight sub headings and were discussed constant value of indicated q˙/input q˙ after the initial time
individually. Some of the conclusions reached were rather response period. From Figs. 3 and 4, it can be seen that this
elementary and were previously reported in Refs (1-3). Others optimum value is about 1.4 for two families of curves for
were somewhat arbitrary and were stated without substantiat- which the cavity radius, a, is held constant while the cavity
ing data. One specific conclusion concerns the ratio of the thickness, b, is varied to span a wide range of the ratio a/b. This
null-point cavity radius, a, to the cavity thickness, b. While is a slightly higher value than reported by earlier analysts. It is
stating that the optimum condition occurred when a 5 b, the important to note that the analytical results do not necessarily
authors of Ref (4) further state that when a 5 0.305 mm have to give a value of indicated q˙/input q˙ 5 1.0 since this
(0.012 in.) and b 5 0.127 mm (0.005 in.); a/b 5 2.4, the difference can be calibrated in the laboratory. The data graphi-
calculated heat flux will be 20 % higher than the actual heat cally illustrated on Figs. 3 and 4 and substantiate conclusions
flux. In more recent documentation using more accurate and drawn by the authors of Refs (3 nd 4) that the calculated heat
FIG. 2 Finite Element Model of Null-Point Calorimeter
E 598
FIG. 3 Null-Point Calorimeter Analytical Time Response Data
FIG. 4 Null-Point Calorimeter Analytical Time Response Data
flux can be considerably higher than the actual input heat body material is oxygen-free high conductivity (OFHC) cop-
flux—especially as the ratio of a/b is raised consistently above per. Temperature at the null point is measured by a 0.508 mm
1.5. All of the users of null-point calorimeters assume that the (0.020 in.) diam American National Standards Association
device simulates a semi-infinite body in the time period of (ANSI) type K stainless steel-sheathed thermocouple with
interest. Therefore, the sensor is subject to the finite body 0.102 mm (0.004 in.) diam thermoelements. Although no
1/2
length, L, defined by L/(at) # 1.8 in order that the error in thermocouple attachment is shown, it is assumed that the
indicated heat flux does not exceed one percent (6 and 7). This individual thermocouple wires are in perfect contact with the
restriction agrees well with the earlier work of Howey and backside of the cavity and present no added thermal mass to the
DiCristina (3). system. Details of installing thermocouples in the null point
4.6 A section view sketch of a typical null-point calorimeter cavity and making a proper attachment of the thermocouple
showing all important components and the physical configu- with the copper slug are generally considered to be proprietary
ration of the sensor is shown in Fig. 5. The outside diameter is by the sensor manufacturers. Kidd in Ref (7) states that the
2.36 mm (0.093 in.), the length is 10.2 mm (0.40 in.), and the attachment is made by thermal fusion without the addition of
E 598
FIG. 5 Section View Sketch of Null-Point Calorimeter
foreign materials. Note that the null-point body has a small (8). Most experimenters use the room temperature value of the
flange at the front and back which creates an effective dead air parameter in processing data from null-point calorimeters.
space along the length of the cylinder to enhance one- 4.8 The determination of surface heat flux as a function of
dimensional heat conduction and prevent radial conduction. time and temperature requires a digital computer, programmed
For aerodynamic heat-transfer measurements, the null-point to calculate the correct values of heat-transfer rate. Having the
sensors are generally pressed into the stagnation position of a measured null-point cavity temperature, the problem to be
sphere cone model of the same material (OFHC copper). solved is the inverse problem of heat conduction. Several
4.7 The value of the lumped thermal parameter of copper is versions of the well known Cook and Felderman numerical
not a strong
...