ASTM C885-87(2007)e1
(Test Method)Standard Test Method for Young's Modulus of Refractory Shapes by Sonic Resonance
Standard Test Method for Young's Modulus of Refractory Shapes by Sonic Resonance
SIGNIFICANCE AND USE
Young's modulus is a fundamental mechanical property of a material.
This test method is used to determine the dynamic modulus of elasticity of rectangular shapes. Since the test is nondestructive, specimens may be used for other tests as desired.
This test method is useful for research and development, engineering application and design, manufacturing process control, and for developing purchasing specifications.
The fundamental assumption inherent in this test method is that a Poisson's ratio of 1/6 is typical for heterogeneous refractory materials. The actual Poisson's ratio may differ.
SCOPE
1.1 This test method covers a procedure for measuring the resonance frequency in the flexural (transverse) mode of vibration of rectangular refractory brick or rectangularly shaped monoliths at room temperature. Young's modulus is calculated from the resonance frequency of the shape, its mass (weight) and dimensions.
1.2 Units—The values stated in inch-pound units are to be regarded as standard. The values given in parentheses are mathematical conversions to SI units that are provided for information only and are not considered standard.
1.2.1 Although the Hertz (Hz) is an SI unit, it is derived from seconds which is also an inch-pound unit.
1.3 This standard does not purport to address all of the safety concerns, if any,associated with its use. It is the responsibility of the user of this standard to establish appropriate safety and health practices and determine the applicability of regulatory limitations prior to use.
General Information
Relations
Standards Content (Sample)
NOTICE: This standard has either been superseded and replaced by a new version or withdrawn.
Please contact ASTM International (www.astm.org) for the latest information.
´1
Designation:C885–87 (Reapproved 2007)
Standard Test Method for
Young’s Modulus of Refractory Shapes by Sonic
Resonance
This standard is issued under the fixed designation C885; the number immediately following the designation indicates the year of
original adoption or, in the case of revision, the year of last revision. A number in parentheses indicates the year of last reapproval. A
superscript epsilon (´) indicates an editorial change since the last revision or reapproval.
´ NOTE—Section 1.2 was added and editorial changes made in November 2009.
1. Scope tal Frequencies of Carbon and Graphite Materials by Sonic
Resonance
1.1 This test method covers a procedure for measuring the
C848 Test Method for Young’s Modulus, Shear Modulus,
resonance frequency in the flexural (transverse) mode of
and Poisson’s Ratio For Ceramic Whitewares by Reso-
vibration of rectangular refractory brick or rectangularly
nance
shaped monoliths at room temperature. Young’s modulus is
calculated from the resonance frequency of the shape, its mass
3. Summary of Test Method
(weight) and dimensions.
3.1 Test specimens are vibrated in flexure over a broad
1.2 Units—The values stated in inch-pound units are to be
frequencyrange;mechanicalexcitationisprovidedthroughthe
regarded as standard. The values given in parentheses are
use of a vibrating driver that transforms an initial electrical
mathematical conversions to SI units that are provided for
signal into a mechanical vibration. A detector senses the
information only and are not considered standard.
resultingmechanicalvibrationsofthespecimenandtransforms
1.2.1 Although the Hertz (Hz) is an SI unit, it is derived
them into an electrical signal that can be displayed on the
from seconds which is also an inch-pound unit.
screen of an oscilloscope to detect resonance by a Lissajous
1.3 This standard does not purport to address all of the
figure.The calculation ofYoung’s modulus from the resonance
safety concerns, if any,associated with its use. It is the
frequency measured is simplified by assuming that Poisson’s
responsibility of the user of this standard to establish appro-
ratio is ⁄6 for all refractory materials.
priate safety and health practices and determine the applica-
bility of regulatory limitations prior to use.
4. Significance and Use
2. Referenced Documents 4.1 Young’s modulus is a fundamental mechanical property
2 of a material.
2.1 ASTM Standards:
4.2 This test method is used to determine the dynamic
C134 Test Methods for Size, Dimensional Measurements,
modulus of elasticity of rectangular shapes. Since the test is
and Bulk Density of Refractory Brick and Insulating
nondestructive, specimens may be used for other tests as
Firebrick
desired.
C215 Test Method for Fundamental Transverse, Longitudi-
4.3 Thistestmethodisusefulforresearchanddevelopment,
nal, and Torsional Resonant Frequencies of Concrete
engineering application and design, manufacturing process
Specimens
control, and for developing purchasing specifications.
C623 Test Method for Young’s Modulus, Shear Modulus,
4.4 The fundamental assumption inherent in this test
and Poisson’s Ratio for Glass and Glass-Ceramics by
method is that a Poisson’s ratio of ⁄6 is typical for heteroge-
Resonance
neous refractory materials. The actual Poisson’s ratio may
C747 Test Method for Moduli of Elasticity and Fundamen-
differ.
5. Apparatus
This test method is under the jurisdiction of ASTM Committee C08 on
5.1 A block diagram of a suggested test apparatus arrange-
Refractories and is the direct responsibility of Subcommittee C08.01 on Strength.
ment is shown in Fig. 1. Details of the equipment are as
Current edition approved March 1, 2007. Published April 2007. Originally
follows:
approved in 1978. Last previous edition approved in 2002 as C885 – 87 (2002).
DOI: 10.1520/C0885-87R07.
5.1.1 Audio Oscillator, having a continuously variable
For referenced ASTM standards, visit the ASTM website, www.astm.org, or
calibrated-frequency output from about 50 Hz to at least 10
contact ASTM Customer Service at service@astm.org. For Annual Book of ASTM
kHz.
Standards volume information, refer to the standard’s Document Summary page on
the ASTM website.
Copyright © ASTM International, 100 Barr Harbor Drive, PO Box C700, West Conshohocken, PA 19428-2959, United States.
NOTICE: This standard has either been superseded and replaced by a new version or withdrawn.
Please contact ASTM International (www.astm.org) for the latest information.
´1
C885–87 (2007)
FIG. 1 Block Diagram of Apparatus
5.1.2 Audio Amplifier, having a power output sufficient to rectangular straight shapes of monolithic refractories, or rect-
ensure that the type of driver used can excite the specimen; the angularspecimenscutfrommonolithicshapes.Forbestresults,
output of the amplifier must be adjustable. their length to thickness ratio should be at least 3 to 1.
5.1.3 Driver, which may consist of a transducer or a Maximumspecimensizeandmassareprimarilydeterminedby
loudspeaker from which the cone has been removed and the test system’s energy capability and by the resonance
replaced with a probe (connecting rod) oriented parallel to the
direction of the vibration; suitable vibration-isolating mounts.
NOTE 1—For small specimens, an air column may preferably be used
for “coupling” the loudspeaker to the specimen.
5.1.4 Detector, which may be a transducer or a balance-
mounted monaural (crystal or magnetic) phonograph pick-up
cartridge of good frequency response; the detector should be
movable across the specimen; suitable vibration-isolating
mounts.
FIG. 2 Typical Specimen Positioning for Measurement of Flexural
5.1.5 Pre-Scope Amplifier in the detector circuit,
Resonance
impedance-matched with the detector used; the output must be
adjustable.
5.1.6 Indicating Devices, including an oscilloscope, a reso-
response characteristics of the material. Minimum specimen
nance indicator (voltmeter or ammeter), and a frequency
size and mass are primarily determined by adequate and
indicator, which may be the control dial of the audio-oscillator
optimum coupling of the driver and the detector to the
(accurately readable to 6 30 Hz or better) or, preferably, a
specimen, and by the resonance response characteristics of the
frequency meter, for example, a digital frequency counter.
material. Measure the mass (weight) and dimensions of the dry
5.1.7 Specimen Support, consisting of two knife edges (can
specimens in accordance with Test Methods C134 and record.
be steel, rubber-coated steel, or medium-hard rubber) of a
length at least equal to the width of the specimens; the distance
7. Procedure
between the knife edges must be adjustable.
7.1 Refractories can vary markedly in their response to the
NOTE 2—The support for the knife edges may be a foam rubber pad,
driver’s frequency; the geometry of the specimens also plays a
and should be vibration-isolated from drive and detector supports.
significant role in their response characteristics. Variations in
NOTE 3—Alternatively, knife edges can be omitted and the specimen
the following procedure are permissible as long as flexural and
may be placed directly on a foam rubber pad if the test material is easily
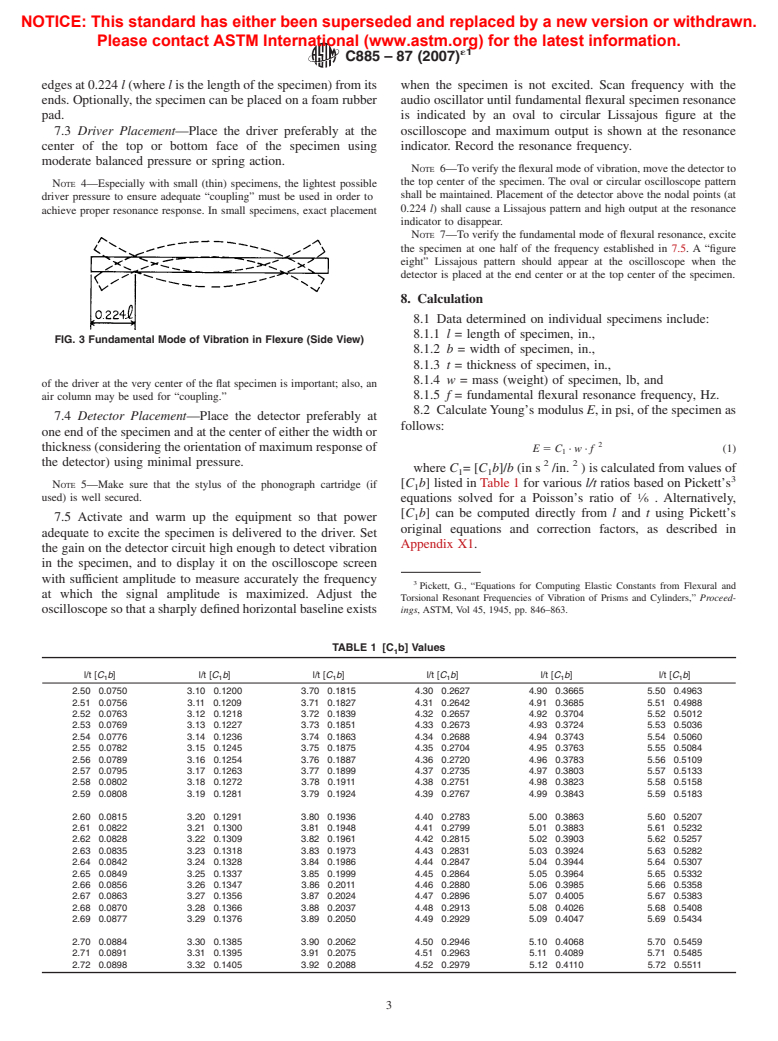
fundamental resonance are verified (Note 6 and Note 7). Fig. 2
excitable due to its composition and geometry.
and Fig. 3 illustrate a typical specimen positioning and the
6. Sampling and Specimen Preparation
desired mode of vibration, respectively.
6.1 Specimensmustberectangularprisms.Theymaybefull 7.2 Sample Placement—Place the specimen “flat” (thick-
straight brick or rectangular samples cut from brick shapes; ness dimension perpendicular to supports) on parallel knife
NOTICE: This standard has either been superseded and replaced by a new version or withdrawn.
Please contact ASTM International (www.astm.org) for the latest information.
´1
C885–87 (2007)
edges at 0.224 l (where l is the length of the specimen) from its when the specimen is not excited. Scan frequency with the
ends. Optionally, the specimen can be placed on a foam rubber audio oscillator until fundamental flexural specimen resonance
pad. is indicated by an oval to circular Lissajous figure at the
7.3 Driver Placement—Place the driver preferably at the oscilloscope and maximum output is shown at the resonance
center of the top or bottom face of the specimen using indicator. Record the resonance frequency.
moderate balanced pressure or spring action.
NOTE 6—To verify the flexural mode of vibration, move the detector to
the top center of the specimen. The oval or circular oscilloscope pattern
NOTE 4—Especially with small (thin) specimens, the lightest possible
shall be maintained. Placement of the detector above the nodal points (at
driver pressure to ensure adequate “coupling” must be used in order to
0.224 l) shall cause a Lissajous pattern and high output at the resonance
achieve proper resonance response. In small specimens, exact placement
indicator to disappear.
NOTE 7—To verify the fundamental mode of flexural resonance, excite
the specimen at one half of the frequency established in 7.5. A “figure
eight” Lissajous pattern should appear at the oscilloscope when the
detector is placed at the end center or at the top center of the specimen.
8. Calculation
8.1 Data determined on individual specimens include:
8.1.1 l = length of specimen, in.,
FIG. 3 Fundamental Mode of Vibration in Flexure (Side View)
8.1.2 b = width of specimen, in.,
8.1.3 t = thickness of specimen, in.,
8.1.4 w = mass (weight) of specimen, lb, and
of the driver at the very center of the flat specimen is important; also, an
8.1.5 f = fundamental flexural resonance frequency, Hz.
air column may be used for “coupling.”
8.2 CalculateYoung’s modulus E, in psi, of the specimen as
7.4 Detector Placement—Place the detector preferably at
follows:
one end of the specimen and at the center of either the width or
thickness (considering the orientation of maximum response of
E 5 C · w · f (1)
2 2
the detector) using minimal pressure.
where C =[C b]/b (in s /in. ) is calculated from values of
1 1
[C b] listed in Table 1 for various l/t ratios based on Pickett’s
NOTE 5—Make sure that the stylus of the phonograph cartridge (if
used) is well secured.
equations solved for a Poisson’s ratio of ⁄6 . Alternatively,
[C b] can be computed directly from l and t using Pickett’s
7.5 Activate and warm up the equipment so that power
original equations and correction factors, as described in
adequate to excite the specimen is delivered to the driver. Set
Appendix X1.
the gain on the detector circuit high enough to detect vibration
in the specimen, and to display it on the oscilloscope screen
with sufficient amplitude to measure accurately the frequency
Pickett, G., “Equations for Computing Elastic Constants from Flexural and
at which the signal amplitude is maximized. Adjust the
Torsional Resonant Frequencies of Vibration of Prisms and Cylinders,” Proceed-
oscilloscope so that a sharply defined horizontal baseline exists ings, ASTM, Vol 45, 1945, pp. 846–863.
TABLE 1 [C b] Values
l/t [C b] l/t [C b] l/t [C b] l/t [C b] l/t [C b] l/t [C b]
1 1 1 1 1 1
2.50 0.0750 3.10 0.1200 3.70 0.1815 4.30 0.2627 4.90 0.3665 5.50 0.4963
2.51 0.0756 3.11 0.1209 3.71 0.1827 4.31 0.2642 4.91 0.3685 5.51 0.4988
2.52 0.0763 3.12 0.1218 3.72 0.1839 4.32 0.2657 4.92 0.3704 5.52 0.5012
2.53 0.0769 3.13 0.1227 3.73 0.1851 4.33 0.2673 4.93 0.3724 5.53 0.5036
2.54 0.0776 3.14 0.1236 3.74 0.1863 4.34 0.2688 4.94 0.3743 5.54 0.5060
2.55 0.0782 3.15 0.1245 3.75 0.1875 4.35 0.2704 4.95 0.3763 5.55 0.5084
2.56 0.0789 3.16 0.1254 3.76 0.1887 4.36 0.2720 4.96 0.3783 5.56 0.5109
2.57 0.0795 3.17 0.1263 3.77 0.1899 4.37 0.2735 4.97 0.3803 5.57 0.5133
2.58 0.0802 3.18 0.1272 3.78 0.1911 4.38 0.2751 4.98 0.3823 5.58 0.5158
2.59 0.0808 3.19 0.1281 3.79 0.1924 4.39 0.2767 4.99 0.3843 5.59 0.5183
2.60 0.0815 3.20 0.1291 3.80 0.1936 4.40 0.2783 5.00 0.3863 5.60 0.5207
2.61 0.0822 3.21 0.1300 3.81 0.1948 4.41 0.2799 5.01 0.3883 5.61 0.5232
2.62 0.0828 3.22 0.1309 3.82 0.1961 4.42 0.2815 5.02 0.3903 5.62 0.5257
2.63 0.0835 3.23 0.1318 3.83 0.1973 4.43 0.2831 5.03 0.3924 5.63 0.5282
2.64 0.0842 3.24 0.1328 3.84 0.1986 4.44 0.2847 5.04 0.3944 5.64 0.5307
2.65 0.0849 3.25 0.1337 3.85 0.1999 4.45 0.2864 5.05 0.3964 5.65 0.5332
2.66 0.0856 3.26 0.1347 3.86 0.2011 4.46 0.2880 5.06 0.3985 5.66 0.5358
2.67 0.0863 3.27 0.1356 3.87 0.2024 4.47 0.2896 5.07 0.4005 5.67 0.5383
2.68 0.0870 3.28 0.1366 3.88 0.2037 4.48 0.2913 5.08 0.4026 5.68 0.5408
2.69 0.0877 3.29 0.1376 3.89 0.2050 4.49 0.2929 5.09 0.4047 5.69 0.5434
2.70 0.0884 3.30 0.1385 3.90 0.2062 4.50 0.2946 5.10 0.4068 5.70 0.5459
2.71 0.0891 3.31 0.1395 3.91 0.2075 4.51 0.2963 5.11 0.4089 5.71 0.5485
2.72 0.0898 3.32 0.1405 3.92 0.2088 4.52 0.2979 5.12 0.4110 5.72 0.5511
NOTICE: This standard has either been superseded and replaced by a new version or withdrawn.
Please contact ASTM International (www.astm.org) for the latest information.
´1
C885–87 (2007)
TABLE 1 Continued
l/t [C b] l/t [C b] l/t [C b] l/t [C b] l/t [C b] l/t [C b]
1 1 1 1 1 1
2.73 0.0905 3.33 0.1415 3.93 0.2101 4.53 0.2996 5.13 0.4131 5.73 0.5537
2.74 0.0912 3.34 0.1425 3.94 0.2115 4.54 0.3013 5.14 0.4152 5.74 0.5562
2.75 0.0920 3.35 0.1435 3.95 0.2128 4.55 0.3030 5.15 0.4173 5.75 0.5588
2.76 0.0927 3.36 0.1445 3.96 0.2141 4.56 0.3047 5.16 0.4194 5.76 0.5615
2.77 0.0934 3.37 0.1455 3.97 0.2154 4.57 0.3064 5.17 0.4216 5.77 0.5641
2.78 0.0942 3.38 0.1465 3.98 0.2168 4.58 0.3081 5.18 0.4237 5.78 0.5667
2.79 0.0949 3.39 0.1475 3.99 0.2181 4.59 0.3098 5.19 0.4258 5.79 0.5693
2.80 0.0957 3.40 0.1485 4.00 0.2194 4.60 0.3116 5.20 0.4280 5.80 0.5720
2.81 0.0964 3.41 0.1496 4.01 0.2208 4.61 0.3133 5.21 0.4302 5.81 0.5746
2.82 0.0972 3.42 0.1506 4.02 0.2222 4.62 0.3150 5.22 0.4323 5.82 0.5773
2.83 0.0979 3.43 0.1516 4.03 0.2235 4.63 0.3168 5.23 0.4345 5.83 0.5799
2.84 0.0987 3.44 0.1527 4.04 0.2249 4.64 0.3185 5.24 0.4367 5.84 0.5826
2.85 0.0994 3.45 0.1537 4.05 0.2263 4.65 0.3203 5.25 0.4389 5.85 0.5853
2.86 0.1002 3.46 0.1548 4.06 0.2277 4.66 0.3220 5.26 0.4411 5.86 0.5880
2.87 0.1010 3.47 0.1558 4.07 0.2290 4.67 0.3238 5.27 0.4433 5.87 0.5907
2.88 0.1018 3.48 0.1569 4.08 0.2304 4.68 0.3256 5.28 0.4455 5.88 0.5934
2.89 0.1026 3.49 0.1579 4.09 0.2318 4.69 0.3274 5.29 0.4478 5.89 0.5961
2.90 0.1033 3.50 0.1590 4.10 0.2332 4.70 0.3292 5.30 0.4500 5.90 0.5989
2.91 0.1041 3.51 0.1601 4.11 0.2347 4.71 0.3310 5.31 0.4522 5.91 0.6016
2.92 0.1049 3.52 0.1612 4.12 0.2361 4.72 0.3328 5.32 0.4545 5.92 0.6043
2.93 0.1057 3.53 0.1623 4.13 0.2375 4.73 0.3346 5.33 0.4568 5.93 0.6071
2.94 0.1065 3.54 0.1633 4.14 0.2389 4.74 0.3364 5.34 0.4590 5.94 0.609
...







Questions, Comments and Discussion
Ask us and Technical Secretary will try to provide an answer. You can facilitate discussion about the standard in here.