ASTM C1548-02(2020)
(Test Method)Standard Test Method for Dynamic Young’s Modulus, Shear Modulus, and Poisson’s Ratio of Refractory Materials by Impulse Excitation of Vibration
Standard Test Method for Dynamic Young’s Modulus, Shear Modulus, and Poisson’s Ratio of Refractory Materials by Impulse Excitation of Vibration
SIGNIFICANCE AND USE
4.1 This test method is nondestructive and is commonly used for material characterization and development, design data generation, and quality control purposes. The test assumes that the properties of the specimen are perfectly isotropic, which may not be true for some refractory materials. The test also assumes that the specimen is homogeneous and elastic. Specimens that are micro-cracked are difficult to test since they do not yield consistent results. Specimens with low densities have a damping effect and are easily damaged locally at the impact point. Insulating bricks can generally be tested with this technique, but fibrous insulating materials are generally too weak and soft to test.
4.2 For quality control use, the test method may be used for measuring only resonant frequencies of any standard size specimen. An elastic modulus calculation may not be needed or even feasible if the shape is nonstandard, such as a slide gate plate containing a hole. Since specimens will vary in both size and mass, acceptable frequencies for each shape and material must be established from statistical data.
4.3 Dimensional variations can have a significant effect on modulus values calculated from the frequency measurements. Surface grinding may be required to bring some materials into the specified tolerance range.
4.4 Since cylindrical shapes are not commonly made from refractory materials they are not covered by this test method, but are covered in Test Method C215.
SCOPE
1.1 This test method covers the measurement of the fundamental resonant frequencies for the purpose of calculating the dynamic Young’s modulus, the dynamic shear modulus (also known as the modulus of rigidity), and the dynamic Poisson’s ratio of refractory materials at ambient temperatures. Specimens of these materials possess specific mechanical resonant frequencies, which are determined by the elastic modulus, mass, and geometry of the test specimen. Therefore, the dynamic elastic properties can be computed if the geometry, mass, and mechanical resonant frequencies of a suitable specimen can be measured. The dynamic Young’s modulus is determined using the resonant frequency in the flexural mode of vibration and the dynamic shear modulus is determined using the resonant frequency in the torsional mode of vibration. Poisson’s ratio is computed from the dynamic Young’s modulus and the dynamic shear modulus.
1.2 Although not specifically described herein, this method can also be performed at high temperatures with suitable equipment modifications and appropriate modifications to the calculations to compensate for thermal expansion.
1.3 The values are stated in SI units and are to be regarded as the standard.
1.4 This standard does not purport to address all of the safety concerns, if any, associated with its use. It is the responsibility of the user of this standard to establish appropriate safety, health, and environmental practices and determine the applicability of regulatory limitations prior to use.
1.5 This international standard was developed in accordance with internationally recognized principles on standardization established in the Decision on Principles for the Development of International Standards, Guides and Recommendations issued by the World Trade Organization Technical Barriers to Trade (TBT) Committee.
General Information
Relations
Standards Content (Sample)
This international standard was developed in accordance with internationally recognized principles on standardization established in the Decision on Principles for the
Development of International Standards, Guides and Recommendations issued by the World Trade Organization Technical Barriers to Trade (TBT) Committee.
Designation: C1548 − 02 (Reapproved 2020)
Standard Test Method for
Dynamic Young’s Modulus, Shear Modulus, and Poisson’s
Ratio of Refractory Materials by Impulse Excitation of
Vibration
This standard is issued under the fixed designation C1548; the number immediately following the designation indicates the year of
original adoption or, in the case of revision, the year of last revision. A number in parentheses indicates the year of last reapproval. A
superscript epsilon (´) indicates an editorial change since the last revision or reapproval.
1. Scope 2. Referenced Documents
1.1 This test method covers the measurement of the funda- 2.1 ASTM Standards:
mental resonant frequencies for the purpose of calculating the C71 Terminology Relating to Refractories
dynamic Young’s modulus, the dynamic shear modulus (also C215 Test Method for Fundamental Transverse,
known as the modulus of rigidity), and the dynamic Poisson’s Longitudinal, and Torsional Resonant Frequencies of
ratio of refractory materials at ambient temperatures. Speci- Concrete Specimens
mens of these materials possess specific mechanical resonant C885 Test Method for Young’s Modulus of Refractory
frequencies, which are determined by the elastic modulus, Shapes by Sonic Resonance
mass, and geometry of the test specimen. Therefore, the C1259 Test Method for Dynamic Young’s Modulus, Shear
dynamic elastic properties can be computed if the geometry, Modulus, and Poisson’s Ratio for Advanced Ceramics by
mass, and mechanical resonant frequencies of a suitable Impulse Excitation of Vibration
specimen can be measured. The dynamic Young’s modulus is
3. Summary of Test Method
determined using the resonant frequency in the flexural mode
3.1 The fundamental resonant frequencies are determined
of vibration and the dynamic shear modulus is determined
by measuring the resonant frequency of specimens struck once
usingtheresonantfrequencyinthetorsionalmodeofvibration.
mechanically with an impacting tool. Frequencies are mea-
Poisson’s ratio is computed from the dynamic Young’s modu-
sured with a transducer held lightly against the specimen using
lus and the dynamic shear modulus.
a signal analyzer circuit. Impulse and transducer locations are
1.2 Although not specifically described herein, this method
selected to induce and measure one of two different modes of
can also be performed at high temperatures with suitable
vibration. The appropriate resonant frequencies, dimensions,
equipment modifications and appropriate modifications to the
and mass of each specimen may be used to calculate dynamic
calculations to compensate for thermal expansion.
Young’s modulus, dynamic shear modulus, and dynamic Pois-
1.3 The values are stated in SI units and are to be regarded
son’s ratio.
as the standard.
4. Significance and Use
1.4 This standard does not purport to address all of the
4.1 This test method is nondestructive and is commonly
safety concerns, if any, associated with its use. It is the
used for material characterization and development, design
responsibility of the user of this standard to establish appro-
data generation, and quality control purposes.The test assumes
priate safety, health, and environmental practices and deter-
that the properties of the specimen are perfectly isotropic,
mine the applicability of regulatory limitations prior to use.
which may not be true for some refractory materials. The test
1.5 This international standard was developed in accor-
also assumes that the specimen is homogeneous and elastic.
dance with internationally recognized principles on standard-
Specimensthataremicro-crackedaredifficulttotestsincethey
ization established in the Decision on Principles for the
do not yield consistent results. Specimens with low densities
Development of International Standards, Guides and Recom-
have a damping effect and are easily damaged locally at the
mendations issued by the World Trade Organization Technical
impactpoint.Insulatingbrickscangenerallybetestedwiththis
Barriers to Trade (TBT) Committee.
technique, but fibrous insulating materials are generally too
weak and soft to test.
This test method is under the jurisdiction of ASTM Committee C08 on
Refractories and is the direct responsibility of Subcommittee C08.01 on Strength. For referenced ASTM standards, visit the ASTM website, www.astm.org, or
Current edition approved Sept. 1, 2020. Published September 2020. Originally contact ASTM Customer Service at service@astm.org. For Annual Book of ASTM
approved in 2002. Last previous edition approved in 2012 as C1548 – 02 (2012). Standards volume information, refer to the standard’s Document Summary page on
DOI: 10.1520/C1548-02R20. the ASTM website.
Copyright © ASTM International, 100 Barr Harbor Drive, PO Box C700, West Conshohocken, PA 19428-2959. United States
C1548 − 02 (2020)
4.2 For quality control use, the test method may be used for However, hammer style impactors which have lightweight
measuring only resonant frequencies of any standard size handles with the impacting mass concentrated near the end are
specimen.Anelasticmoduluscalculationmaynotbeneededor preferred to dropping vertical impactors. Steel hammer style
even feasible if the shape is nonstandard, such as a slide gate impactors, with head weights between 0.3 and 3 % of the
plate containing a hole. Since specimens will vary in both size specimen weight, are recommended. To avoid damaging the
and mass, acceptable frequencies for each shape and material surface of insulating bricks or other weak materials, plastic or
must be established from statistical data. rubber shapes should be substituted for the steel impactors.
5.3 Specimen Support—The support shall permit the speci-
4.3 Dimensional variations can have a significant effect on
modulus values calculated from the frequency measurements. men to vibrate freely without restricting the desired mode of
vibration. For room temperature measurements, soft rubber or
Surface grinding may be required to bring some materials into
the specified tolerance range. plastic strips located at the nodal points are typically used.
Alternately, the specimen can be placed on a thick, soft rubber
4.4 Since cylindrical shapes are not commonly made from
pad. For elevated temperature measurements, the specimen
refractory materials they are not covered by this test method,
may be suspended from support wires wrapped around the
but are covered in Test Method C215.
specimen at nodal points and passing vertically out of the test
chamber.
5. Apparatus
5.1 Electronic System—The electronic system in Fig. 1
6. Test Specimen
consists of a signal conditioner/amplifier, a signal analyzer, a
6.1 Preparation—Test specimens shall be prepared to yield
frequency readout device, and a signal transducer for sensing
uniform rectangular shapes. Normally, brick-sized specimens
the vibrations. The system should have sufficient precision to
are used. Although smaller bars cut from bricks are easily
measure frequencies to an accuracy of 0.1 %. Commercial
tested for flexural resonant frequencies, it is more difficult to
instrumentation is available which meets this requirement.
obtain torsional resonance in specimens of square cross sec-
5.1.1 Frequency Analyzer—This consists of a signal
tion. Some pressed brick shapes are dimensionally uniform
conditioner/amplifier to power the transducer and a digital
enough to test without surface grinding, but specimens cut
waveform analyzer or frequency counter with storage capabil-
from larger shapes or prepared by casting or other means often
ity to analyze the signal from the transducer. The waveform
require surface grinding of one or more surfaces to meet the
analyzer shall have a sampling rate of at least 20 000 Hz. The
dimensional criteria noted below.
frequency counter should have an accuracy of 0.1 %.
6.2 Heat Treatment—All specimens shall be prefired to the
5.1.2 Sensor—Apiezeoelectric accelerometer contact trans-
desired temperature and oven dried before testing.
ducerismostcommonlyused,althoughnoncontacttransducers
basedonacoustic,magnetic,orcapacitancemeasurementsmay 6.3 Dimensional Ratios—Specimens having either very
also be used.The transducer shall have a frequency response in small or very large ratios of length to maximum transverse
therangeof50Hzto10 000Hz,andhavearesonantfrequency dimensions are frequently difficult to excite in the fundamental
above 20 000 Hz. The sensor shall have a mark identifying the modes of vibration. Best results are obtained when this ratio is
maximum sensitivity direction so that it can be properly between 3 and 5. For use of the equations in this method, the
oriented for each vibration mode. ratio must be at least 2.
5.2 Impactor—Because refractory materials are tested with
6.4 Dimensional Uniformity—Rectangular specimens shall
specimens of various sizes, it is not feasible to specify an have surfaces that are flat and parallel to within 60.5 % of the
impactor with a specific size, weight, or construction method. nominal measured value.
6.5 Weight (or Mass) and Dimensions—Determine the
weight (or mass) to the nearest 60.5 %. Measure each dimen-
Equipment found suitable is available from J. W. Lemmons, Inc., 3466
sion to within 60.5 %.
Bridgeland Drive, Suite 230, St. Louis, MO 63044-260.
FIG. 1 Diagram of Test Apparatus
C1548 − 02 (2020)
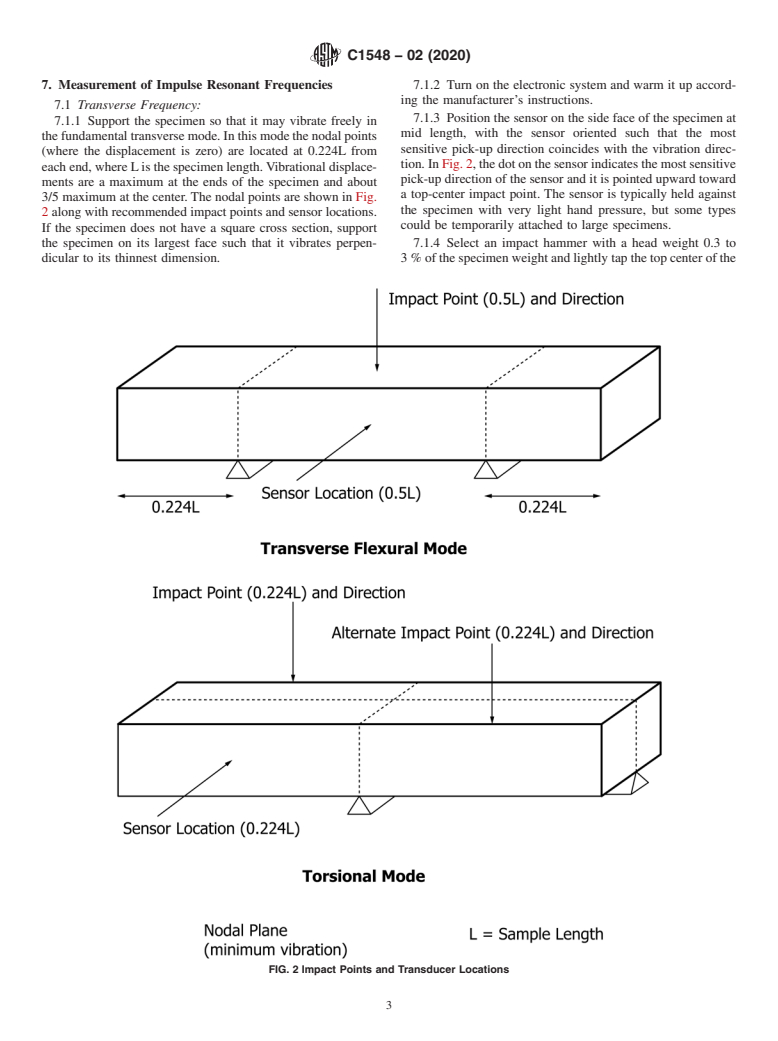
7. Measurement of Impulse Resonant Frequencies 7.1.2 Turn on the electronic system and warm it up accord-
ing the manufacturer’s instructions.
7.1 Transverse Frequency:
7.1.3 Position the sensor on the side face of the specimen at
7.1.1 Support the specimen so that it may vibrate freely in
mid length, with the sensor oriented such that the most
thefundamentaltransversemode.Inthismodethenodalpoints
sensitive pick-up direction coincides with the vibration direc-
(where the displacement is zero) are located at 0.224L from
tion.InFig.2,thedotonthesensorindicatesthemostsensitive
each end, where Lis the specimen length.Vibrational displace-
pick-up direction of the sensor and it is pointed upward toward
ments are a maximum at the ends of the specimen and about
a top-center impact point. The sensor is typically held against
3/5 maximum at the center. The nodal points are shown in Fig.
the specimen with very light hand pressure, but some types
2 along with recommended impact points and sensor locations.
could be temporarily attached to large specimens.
If the specimen does not have a square cross section, support
the specimen on its largest face such that it vibrates perpen- 7.1.4 Select an impact hammer with a head weight 0.3 to
dicular to its thinnest dimension. 3 %ofthespecimenweightandlightlytapthetopcenterofthe
FIG. 2 Impact Points and Transducer Locations
C1548 − 02 (2020)
specimen perpendicular to the surface. Note the reading 7.2.2 Turn on the electronic system and warm it up accord-
displayed by the electronic system, allow a few seconds for ing to the manufacturer’s instructions.
existing vibrations to dampen in the specimen, and repeat the
7.2.3 Position the sensor on the side face of the specimen at
procedure at least three times until a consistent value is
0.224L, with the sensor oriented such that the sensitive pick-up
reproduced. Record that value and calculate the resonant
direction coincides with the vibration direction. In Fig. 2, the
frequency from it per the manufacturer’s instructions if fre-
dot on the sensor indicates the most sensitive pick-up direction
quency is not displayed directly. If a consistent value cannot be
of the sensor and it is pointed upward toward a top impact
obtained, either the specimen is damaged or other modes of
point.
vibration are interfering with the measurement.
7.2.4 Select an impact hammer with a head weight 0.3 to
7.2 Torsional Frequency: 3 % of the specimen weight and lightly tap the top of the
7.2.1 Support the specimen so that it may vibrate freely in specimen at a 0.224L location perpendicular to the surface.
torsion. In this mode there is a single nodal point at the center Note the reading displayed by the electronic system, allow a
and vibrations are a maximum at the ends. The impact and few seconds for existing vibrations to dissipate, and repeat the
process at least three times until a consistent value is repro-
sensor pickup points are located at 0.224L from the ends. This
location is a nodal point for flexural vibration and minimizes duced. Record that value and calculate the resonant frequency
interference from flexural vibrations. from it if frequency is not displayed directly.
FIG. 3 Flow Chart for Iterative Determination of Poisson’s Ratio
C1548 − 02 (2020)
8. Calculations value and the final computed value of the Poisson’s ratio.
4,5 Self-consistent values for the moduli are thus obtained.
8.1 Dynamic Young’s Modulus:
6,7
8.1.1 From the fundamental flexural vibration of a rectan-
8.2 Dynamic Shear Modulus:
gular bar:
8.2.1 From the fundamental torsional vibration of a rectan-
2 3
gular bar:
mf L
f
E 5 0.9465 T (1)
S DS 3D
b t
~4Lmf ! B
t
G 5 (2)
H JH J
bt 11A
~ ! ~ !
where:
where:
E = Young’s modulus, Pa,
m = mass of the bar, g,
G = shear modulus, Pa, and
b = width of the bar, mm,
f = fundamental resonant frequency of the bar in torsion,
t
L = length of the bar, mm,
Hz.
t = thickness of the bar, mm,
b t
f = fundamental resonant frequency of the bar in flexure,
f
HS D S DJ
t b
Hz, and
B 5
2 6
T = correction factor for fundamental flexural made to t t t
4 2 2.52 10.21
H S D S D S D J
account for finite thickness of bar, Poisson’s ratio, etc. b b b
2 3
b b b
0.5062 2 0.8776 10.3504 2 0.0078
H S D S D S D J
t t t
t t A 5
b b
T 5 116.585 ~110.0752µ10.8109µ ! 2 0.868 2
S D S D
L L 12.03 19.892
H S D S D J
t t
t
8.340 110.2023µ12.173µ
HS ~ ! S D DJ
8.3 Poisson’s Ratio:
L
8.3.1 From E and G:
t
1.00016.338 110.1408µ11.536µ
H ~ ! J
S S D D
L
E
µ 5 21 (3)
S D
2G
µ = Poisson’s ratio.
where:
8.1.1.1 If L / t ≥ 20, T can be simplified to:
µ = Poisson’s ratio.
t
T 5 1.00016.585
S S D D
8.4 Use the following conversion factor:
L
1Pa 5 1.450 310 psi
and E can be calculated directly.
8.1.1.2 If L / t < 20, then an initial Poisson’s ratio must be
9. Report
assumed to start the computations.An iterative process is then
used to determine a value of Poisson’s ratio, based on experi-
9.1 The report shall include the following:
mental Young’s modulus and shear modulus. This iterative
9.1.1 Identification of specific tests performed, a detailed
process is shown in Fi
...







Questions, Comments and Discussion
Ask us and Technical Secretary will try to provide an answer. You can facilitate discussion about the standard in here.