ASTM D5457-93(1998)
(Specification)Standard Specification for Computing the Reference Resistance of Wood-Based Materials and Structural Connections for Load and Resistance Factor Design
Standard Specification for Computing the Reference Resistance of Wood-Based Materials and Structural Connections for Load and Resistance Factor Design
SCOPE
1.1 This specification covers procedures for computing the reference resistance of wood-based materials and structural connections for use in load and resistance factor design (LRFD). The reference resistance derived from this specification applies to the design of structures addressed by the load combinations in ASCE 7-88.
1.2 A commentary to this specification is provided in Appendix X1.
General Information
Relations
Standards Content (Sample)
NOTICE: This standard has either been superseded and replaced by a new version or withdrawn.
Contact ASTM International (www.astm.org) for the latest information
Designation: D 5457 – 93 (Reapproved 1998)
Standard Specification for
Computing the Reference Resistance of Wood-Based
Materials and Structural Connections for Load and
Resistance Factor Design
This standard is issued under the fixed designation D 5457; the number immediately following the designation indicates the year of
original adoption or, in the case of revision, the year of last revision. A number in parentheses indicates the year of last reapproval. A
superscript epsilon (e) indicates an editorial change since the last revision or reapproval.
INTRODUCTION
Load and resistance factor design (LRFD) is a structural design method that uses concepts from
reliability theory and incorporates them into a procedure usable by the design community. The basic
design equation requires establishing a reference resistance based on several material property
parameters. A standard method for calculating the required material property input data is critical so
that all wood-based structural materials can be treated equitably. This specification provides the
procedures that are required for the generation of reference resistance for LRFD.
1. Scope D 2719 Test Methods for Structural Panels in Shear
Through-the-Thickness
1.1 This specification covers procedures for computing the
D 2915 Practice for Evaluating Allowable Properties for
reference resistance of wood-based materials and structural
Grades of Structural Lumber
connections for use in load and resistance factor design
D 3043 Methods of Testing Structural Panels in Flexure
(LRFD). The reference resistance derived from this specifica-
D 3500 Test Method for Structural Panels in Tension
tion applies to the design of structures addressed by the load
D 3501 Methods of Testing Plywood in Compression
combinations in ASCE 7-88.
D 4761 Test Method for Mechanical Properties of Lumber
1.2 A commentary to this specification is provided in
and Wood-Base Structural Material
Appendix X1.
D 5055 Specification for Establishing and Monitoring
2. Referenced Documents Structural Capacities of Prefabricated Wood I-Joists
E 105 Practice for Probability Sampling of Materials
2.1 ASTM Standards:
2.2 ASCE Standard:
D 9 Terminology Relating to Wood
ASCE 7-88 Minimum Design Loads for Buildings and
D 143 Method of Testing Small Clear Specimens of Tim-
Other Structures
ber
D 198 Methods of Static Tests of Timbers in Structural
3. Terminology
Sizes
3.1 Definitions—For general definitions of terms related to
D 1037 Test Methods of Evaluating the Properties of Wood-
wood, refer to Terminology D 9.
Base Fiber and Particle Panel Materials
3.1.1 coeffıcient of variation, CV —a relative measure of
w
D 1761 Method of Testing Mechanical Fasteners in Wood
variability. For this specification, the calculation of CV is
D 1990 Practice for Establishing Allowable Properties for w
based on the shape parameter of the 2-parameter Weibull
Visually-Graded Dimension Lumber From In-Grade Tests
2 distribution. It is not the traditional sample standard deviation
of Full-Size Specimens
of the data divided by the sample mean.
D 2718 Test Method for Structural Panels in Planar Shear
2 3.1.2 data confidence factor, V—a factor that is used to
(Rolling Shear)
adjust member reference resistance for sample variability and
sample size.
This specification is under the jurisdiction of ASTM Committee D07 on Wood
and is the direct responsibility of Subcommittee D07.02 on Lumber and Engineered
Wood Products. Annual Book of ASTM Standards, Vol 14.02.
Current edition approved Oct. 15, 1993. Published December 1993. Available from American Society of Civil Engineers, 345 East 47th Street, New
Annual Book of ASTM Standards, Vol 04.10. York, NY 10017-2398.
Copyright © ASTM International, 100 Barr Harbor Drive, PO Box C700, West Conshohocken, PA 19428-2959, United States.
D 5457 – 93 (1998)
3.1.3 distribution percentile, R —the value of the distribu- 6. Reference Resistance for LRFD
p
tion associated with proportion, p, of the cumulative distribu-
6.1 The derivation of LRFD reference resistance is ad-
tion function.
dressed in this section. Parameters required for the derivation
3.1.4 format conversion factor, K —a factor applied to
F
of reference resistance are also presented. These parameters
convert resistance from the allowable stress design (ASD)
include the distribution percentile, coefficient of variation, data
format to the LRFD format.
confidence factor, and reliability normalization factor. An
3.1.5 lower tail—a portion of an ordered data set consisting
example derivation of reference resistance is provided in X1.7.
of all test specimens with the lowest property values (for
6.2 Reference Resistance, R —The following equation es-
n
example, lowest strengths).
tablishes reference resistance for LRFD:
3.1.6 reference resistance, R —the value used in LRFD
n
R 5 R 3V3 K (1)
n p R
equations to represent member resistance (that is, strength or
capacity).
where:
3.1.7 reliability normalization factor, K —a factor used to
R = distribution percentile estimate,
R
p
establish the reference resistance to achieve a target reliability V = data confidence factor, and
index for a reference set of conditions. K = reliability normalization factor.
R
3.1.8 resistance factor—a factor applied to the resistance 6.3 Distribution Percentile Estimate, R— :
p
side of the LRFD equation. 6.3.1 Eq (2) is intended to be used to calculate any percen-
tile of a two-parameter Weibull distribution. The percentile of
4. Sampling
interest depends on the property being estimated.
4.1 Samples selected for analysis and implementation with
1/a
R 5h@2ln~1 2 p!# (2)
p
this specification shall be representative of the population
about which inferences are to be made. Both manufacturing
where:
and material source variability shall be considered. The prin-
h = Weibull scale parameter,
ciples of Practice E 105 shall be maintained. Method D 2915
p = percentile of interest expressed as a decimal (for
provides methods for establishing a sampling plan. Special
example, 0.05), and
attention is directed to sampling procedures in which the
a = Weibull shape parameter.
variability is low and results can be influenced significantly by
6.3.2 The shape (a) and scale (h) parameters of the two-
manufacturing variables. It is essential that the sampling plan
parameter Weibull distribution shall be established to define
address the relative magnitude of the sources of variability.
the distribution of the material resistance. Algorithms for
4.1.1 Data generated from a quality control program shall be
common estimation procedures are provided in Appendix X2.
acceptable if the criteria of 4.1 are maintained.
6.4 Coeffıcient of Variation, CV —The coefficient of varia-
w
4.1.2 When data from multiple data sets are compiled or
tion of the material is necessary when determining the data
grouped, the criteria used to group such data shall be in
confidence factor, V, and the reliability normalization factor,
keeping with the provisions of 4.1. When such procedures are
K . The CV can be estimated from the shape parameter of the
R w
available in applicable product standards, they shall be used.
Weibull distribution as follows:
4.2 Sample Size:
20.92
CV > a (3)
w
4.2.1 For data sets in which all specimens are tested to
failure, the minimum sample size shall be 30. NOTE 2—The above approximation is within 1 % of the exact solution
for CV values between 0.09 and 0.50. An exact relationship of CV and
w w
NOTE 1—The confidence with which population properties can be
a is shown in Appendix X3.
estimated decreases with decreasing sample size. For sample sizes less
6.5 Data Confidence Factor, V—The data confidence fac-
than 60, extreme care must be taken during sampling to ensure a
tor, V, accounts for uncertainty associated with data sets. This
representative sample.
factor, which is a function of coefficient of variation, sample
4.2.2 For lower tail data sets, a minimum of 60 failed
size, and reference percentile, is applied as a multiplier on the
observations is required for sample sizes of n = 600 or less.
distribution estimate. Table 1 provides data confidence factors
(This represents at least the lower 10 % of the distribution.) For
appropriate for lower fifth-percentile estimates.
sample sizes greater than 600, a minimum of the lowest 10 %
of the distribution is required (for example, sample size, n
NOTE 3—When a distribution tolerance limit is developed on a basis
= 720, 0.10 (720) = 72 failed test specimens in the lower tail).
consistent with V, the data confidence factor is taken as unity.
Only parameter estimation procedures designed specifically for
6.6 Reliability Normalization Factor, K —The reliability
R
lower tail data sets shall be used (see Appendix X2).
normalization factor, K , is used to adjust the distribution
R
estimate (for example, R ) to achieve a target reliability
5. Testing 0.05
index. The reliability normalization factor is the ratio of the
5.1 Testing shall be conducted in accordance with appropri-
ate standard testing procedures. The intent of the testing shall
be to develop data that represent the capacity of the product in
Weibull, W., “A Statistical Theory of the Strength of Materials,” Proceedings of
service.
the Royal Swedish Institute of Engineering Research, Stockholm, Sweden, Report
5.2 Periodic Property Assessment—Periodic testing is rec-
No. 151, 1939, pp. 1–45.
ommended to verify that the properties of production material
Load and Resistance Factor Design for Engineered Wood Construction—A
remain representative of published properties. Pre-Standard Report, American Society of Civil Engineers, 1988.
D 5457 – 93 (1998)
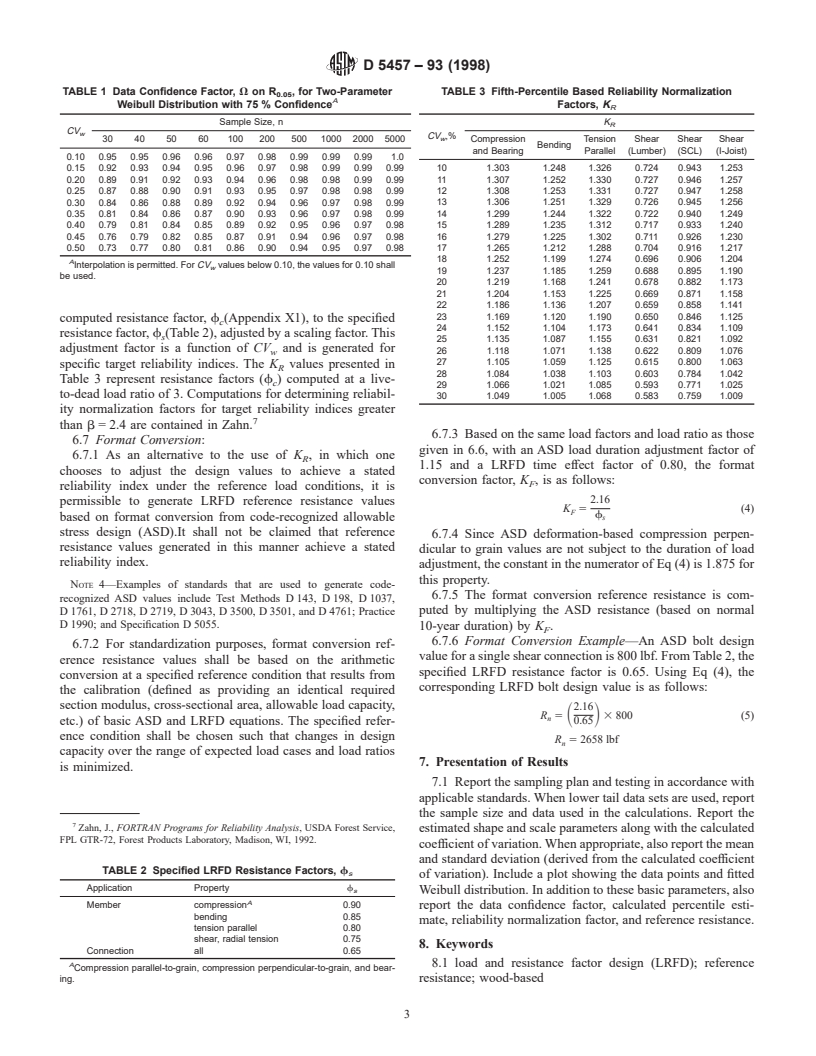
TABLE 1 Data Confidence Factor, V on R , for Two-Parameter TABLE 3 Fifth-Percentile Based Reliability Normalization
0.05
A
Weibull Distribution with 75 % Confidence Factors, K
R
Sample Size, n K
R
CV
w
CV ,%
30 40 50 60 100 200 500 1000 2000 5000 w Compression Tension Shear Shear Shear
Bending
and Bearing Parallel (Lumber) (SCL) (I-Joist)
0.10 0.95 0.95 0.96 0.96 0.97 0.98 0.99 0.99 0.99 1.0
0.15 0.92 0.93 0.94 0.95 0.96 0.97 0.98 0.99 0.99 0.99 10 1.303 1.248 1.326 0.724 0.943 1.253
11 1.307 1.252 1.330 0.727 0.946 1.257
0.20 0.89 0.91 0.92 0.93 0.94 0.96 0.98 0.98 0.99 0.99
0.25 0.87 0.88 0.90 0.91 0.93 0.95 0.97 0.98 0.98 0.99 12 1.308 1.253 1.331 0.727 0.947 1.258
0.30 0.84 0.86 0.88 0.89 0.92 0.94 0.96 0.97 0.98 0.99 13 1.306 1.251 1.329 0.726 0.945 1.256
0.35 0.81 0.84 0.86 0.87 0.90 0.93 0.96 0.97 0.98 0.99 14 1.299 1.244 1.322 0.722 0.940 1.249
0.40 0.79 0.81 0.84 0.85 0.89 0.92 0.95 0.96 0.97 0.98 15 1.289 1.235 1.312 0.717 0.933 1.240
0.45 0.76 0.79 0.82 0.85 0.87 0.91 0.94 0.96 0.97 0.98 16 1.279 1.225 1.302 0.711 0.926 1.230
0.50 0.73 0.77 0.80 0.81 0.86 0.90 0.94 0.95 0.97 0.98 17 1.265 1.212 1.288 0.704 0.916 1.217
18 1.252 1.199 1.274 0.696 0.906 1.204
A
Interpolation is permitted. For CV values below 0.10, the values for 0.10 shall
w
19 1.237 1.185 1.259 0.688 0.895 1.190
be used.
20 1.219 1.168 1.241 0.678 0.882 1.173
21 1.204 1.153 1.225 0.669 0.871 1.158
22 1.186 1.136 1.207 0.659 0.858 1.141
23 1.169 1.120 1.190 0.650 0.846 1.125
computed resistance factor, f (Appendix X1), to the specified
c
24 1.152 1.104 1.173 0.641 0.834 1.109
resistance factor, f (Table 2), adjusted by a scaling factor. This
s
25 1.135 1.087 1.155 0.631 0.821 1.092
adjustment factor is a function of CV and is generated for
26 1.118 1.071 1.138 0.622 0.809 1.076
w
27 1.105 1.059 1.125 0.615 0.800 1.063
specific target reliability indices. The K values presented in
R
28 1.084 1.038 1.103 0.603 0.784 1.042
Table 3 represent resistance factors (f ) computed at a live-
c
29 1.066 1.021 1.085 0.593 0.771 1.025
to-dead load ratio of 3. Computations for determining reliabil-
30 1.049 1.005 1.068 0.583 0.759 1.009
ity normalization factors for target reliability indices greater
than b = 2.4 are contained in Zahn.
6.7.3 Based on the same load factors and load ratio as those
6.7 Format Conversion:
given in 6.6, with an ASD load duration adjustment factor of
6.7.1 As an alternative to the use of K , in which one
R
1.15 and a LRFD time effect factor of 0.80, the format
chooses to adjust the design values to achieve a stated
conversion factor, K , is as follows:
F
reliability index under the reference load conditions, it is
2.16
permissible to generate LRFD reference resistance values
K 5 (4)
F
f
based on format conversion from code-recognized allowable s
stress design (ASD).It shall not be claimed that reference
6.7.4 Since ASD deformation-based compression perpen-
resistance values generated in this manner achieve a stated
dicular to grain values are not subject to the duration of load
reliability index.
adjustment, the constant in the numerator of Eq (4) is 1.875 for
this property.
NOTE 4—Examples of standards that are used to generate code-
6.7.5 The format conversion reference resistance is com-
recognized ASD values include Test Methods D 143, D 198, D 1037,
puted by multiplying the ASD resistance (based on normal
D 1761, D 2718, D 2719, D 3043, D 3500, D 3501, and D 4761; Practice
D 1990; and Specification D 5055.
10-year duration) by K .
F
6.7.6 Format Conversion Example—An ASD bolt design
6.7.2 For standardization purposes, format conversion ref-
value for a single shear connection is 800 lbf. From Table 2, the
erence resistance values shall be based on the arithmetic
specified LRFD resistance factor is 0.65. Using Eq (4), the
conversion at a specified reference condition that results from
corresponding LRFD bolt design value is as follows:
the calibration (defined as providing an identical required
section modulus, cross-sectional area, allowable load capacity, 2.16
R 5 3 800 (5)
S D
n
etc.) of basic ASD and LRFD equations. The specified refer- 0.65
ence condition shall be chosen such that changes in design
R 5 2658 lbf
n
capacity over the range of expected load cases and load ratios
7. Presentation of Results
is minimized.
7.1 Report the sampling plan and testing in accordance with
applicable standards. When lower tail data sets are used, report
the sample size and data used in the calculations. Report the
Zahn, J., FORTRAN Programs for Reliability Analysis, USDA Forest Service,
estimated shape and scale parameters along with the calculated
FPL GTR-72, Forest Products Laboratory, Madison, WI, 1992.
coefficient of variation. When appropriate, also report the mean
and standard deviation (derived from the calculated coefficient
TABLE 2 Specified LRFD Resistance Factors, f
s
of variation). Include a plot showing the data points and fitted
Application Property f
s
Weibull distribution. In addition to these basic parameters, also
A
Member compression 0.90 report the data confidence
...







Questions, Comments and Discussion
Ask us and Technical Secretary will try to provide an answer. You can facilitate discussion about the standard in here.